曲面薄膜结构褶皱失稳力学

徐凡; 杨易凡; 汪婷

doi:10.6052/1000-0992-20-038

曲面薄膜结构褶皱失稳力学

doi: 10.6052/1000-0992-20-038

复旦大学航空航天系力学与工程仿真研究所, 上海 200433

基金项目: 国家自然科学基金(11872150, 11602058, 11772094); 上海市青年科技启明星计划(19QA1400500)资助项目.

详细信息

作者简介:
徐凡, 复旦大学教授、博导. 中国力学学会青年工作委员会委员、中国力学学会软物质力学、微纳米力学工作组组员、上海市力学学会常务理事. 主要从事软物质与柔性结构力学、薄膜力学和智能材料力学研究. 工作以第一/通讯作者发表于《PRL》(封面)《JMPS》《Nature Biomed. Eng.》《Adv. Funct. Mater.》《IJSS》《IJES》《CMAME》《EML》《Sci. China Phys. Mech. Astron.》等国内外学术期刊, 成果被《Nature》《Nature Phys.》和《Nature Comput. Sci.》《Nature Biomed. Eng.》等　　　　　　　　　专题评论报道

通讯作者:
fanxu@fudan.edu.cn

中图分类号: O33, O34, O39
计量
- 文章访问数: 3804
- HTML全文浏览量: 835
- PDF下载量: 631
- 被引次数: 0
出版历程
- 收稿日期: 2020-12-26
- 录用日期: 2021-04-19
- 网络出版日期: 2021-05-01
- 刊出日期: 2021-06-25

Curvature-affected instabilities in membranes and surfaces: A review

Institute of Mechanics and Computational Engineering, Department of Aeronautics and Astronautics, Fudan University, Shanghai 200433, China

More Information

Corresponding author: fanxu@fudan.edu.cn

摘要

摘要: 薄膜结构褶皱失稳在微观和宏观尺度会出现相似的形貌, 在过去二十年里引发了学者们极大的研究兴趣. 而几何曲率对薄膜结构的失稳临界、形貌选择和后屈曲演化起着至关重要的作用. 本文回顾近二十年来平面和曲面薄膜结构褶皱失稳力学研究进展, 聚焦曲率影响下的薄膜拉伸和膜基结构在各种激励下的稳定性问题. 有限应变板壳理论模型和数值计算方法的发展推动了对曲率影响下薄膜结构表面形貌多重分岔转变的定量理解、预测和追踪, 不仅推进了对薄膜结构失稳机理的深入理解, 也为抑制褶皱或利用失稳实现多功能表面制造提供了理论基础, 可促进拓扑形貌相关的功能性膜结构的设计及优化.
- 薄膜 /
- 曲率 /
- 褶皱 /
- 分岔 /
- 大变形
Abstract: Instability of soft materials and membrane structures usually shows similar wrinkling patterns across length scales, which has aroused considerable interest in the past twenty years. Curvature and mechanics are intimately connected in thin-film structures, and curvature plays a key role in the critical threshold, mode selection, and post-buckling evolution. Here, we review the advancement of instability mechanics of thin-film structures in the past two decades, from planar to curved geometry, focusing on the curvature-affected instability of stretched membranes and film-substrate systems under various stimuli. Development of the theoretical models and numerical methods of finite strain plate/shell advances fundamental understanding, quantitative prediction, and tracking of multiple bifurcation transitions in morphology and shape of thin-film structures. It not only promotes the in-depth insight into instability mechanism but also guides wrinkle-resistant control and the effective design of morphology-related multifunctional surfaces and membrane structures via harnessing instability.
- thin films /
- curvature /
- wrinkling /
- bifurcation /
- large deformation

HTML全文

图 1 不同尺度下平面构型(a) ~ (e)与曲面构型(f) ~ (j)薄膜结构褶皱形貌对比: (a)石墨烯(Bao et al. 2009), (b)凝胶薄膜, (c)荷叶(Xu et al. 2020a), (d)窗帘(Vandeparre et al. 2011), (e)太阳光帆, (f)弯曲碳纳米管(Poncharal et al. 1999), (g) PDMS微球(Breid & Crosby 2013), (h)百合花瓣(Liang & Mahadevan 2011), (i)袖子(Yang et al. 2018), (j) Google气球

下载: 全尺寸图片幻灯片

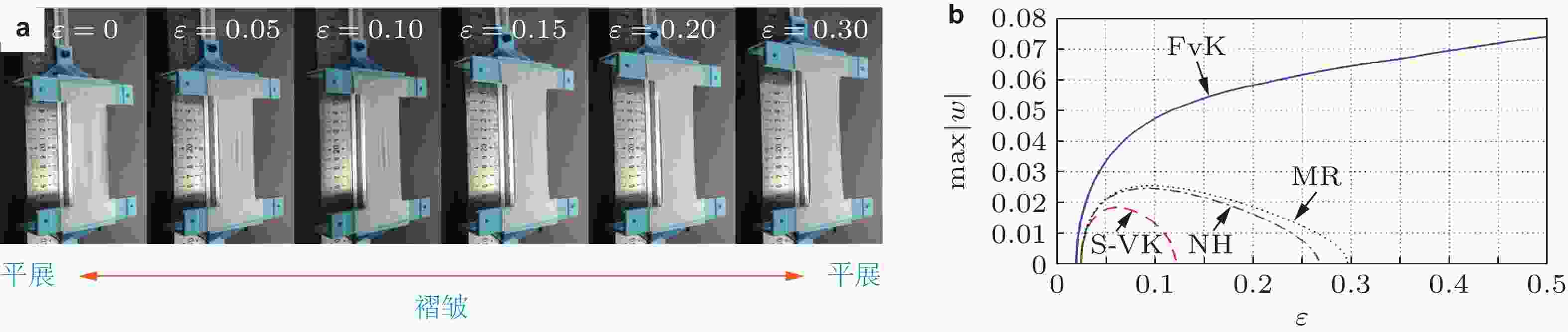
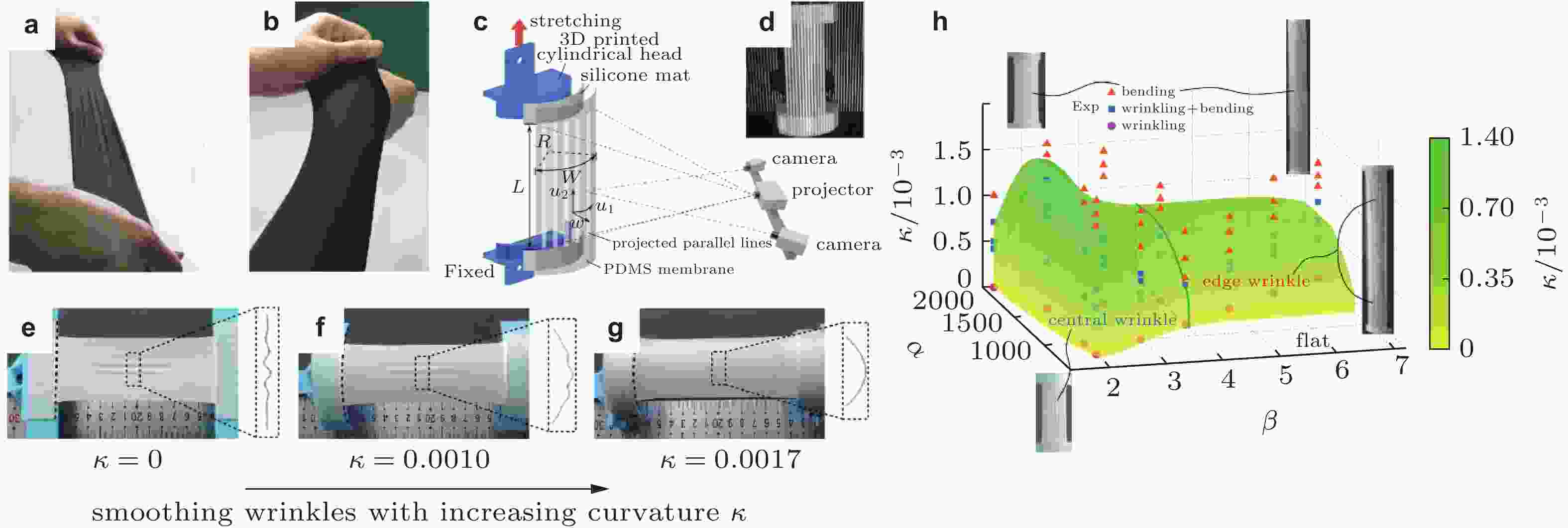
图 2 平面超弹性薄膜拉伸起皱−消皱演化. (a)单轴拉伸实验, 对应拉伸应变分别为$\varepsilon = 0,\;0.05,\; $$ 0.1,\;0.15,\;0.2,\;0.3$; (b)薄膜拉伸失稳分岔图(Li & Healey 2016), 其中点划线、点线、虚线及实线分别表示NH, MR, SVK, FvK模型

下载: 全尺寸图片幻灯片

图 3 (a) (b)平展的袖子受拉时出现横向褶皱, 穿起的袖子受拉时表面光滑. (c) (d)曲面薄膜拉伸实验装置及光栅表面形貌测量. (e) ~ (g)相同拉伸应变下增大曲率可抑制褶皱, 且大于临界曲率时薄膜保持光滑. (h)曲率影响下的稳定性边界三维相图. 理论预测的稳定性界面(整体弯曲变形与局部起褶的边界)与实验(散点)一致(Wang et al. 2020)

下载: 全尺寸图片幻灯片

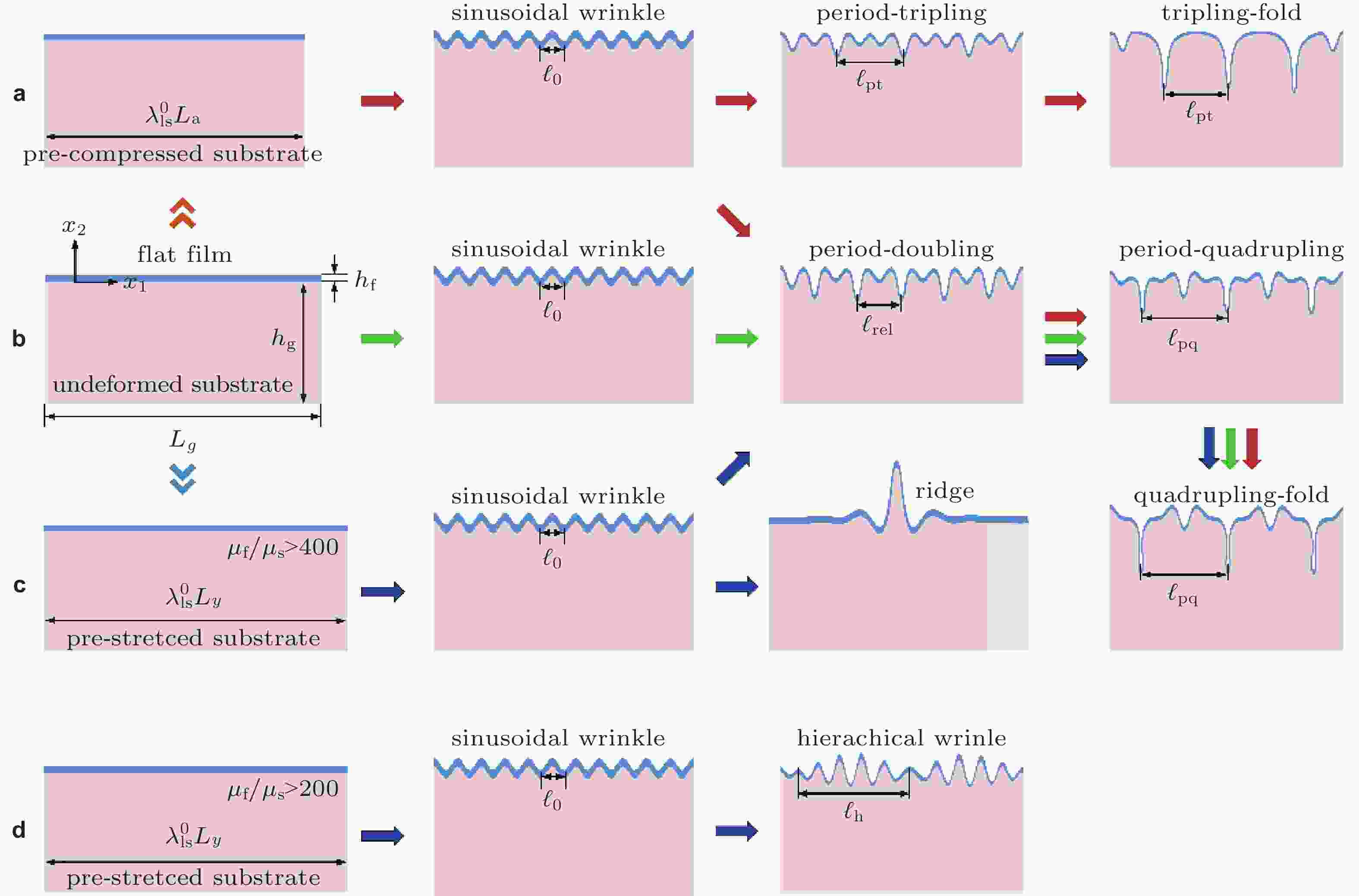
图 4 (a)应变−挠度分岔曲线, 当曲率$\kappa \geqslant {\kappa _{{\rm{cr}}}} \sim 0.0013$时, 只出现整体弯曲(无褶). (b)弯曲能−应变演化曲线, 绿色区域表示整体弯曲与局部起褶的耦合行为, 橙色区域表示只发生整体弯曲变形(无褶). (c)经典DMV壳模型无法预报褶皱消失现象. (d)理论计算演化与实验对比(Wang et al. 2020)

下载: 全尺寸图片幻灯片

图 5 膜基结构失稳模态演化示意图: (a)基底预压缩: 平整→褶皱→三倍周期→折痕, (b)直接压缩: 平整→褶皱→倍周期→四倍周期→折痕, (c)基底预拉伸(高模量比): 平整→褶皱→凸脊, (d)基底预拉伸(低模量比): 平整→褶皱→多级褶皱(Cheng & Xu 2021)

下载: 全尺寸图片幻灯片

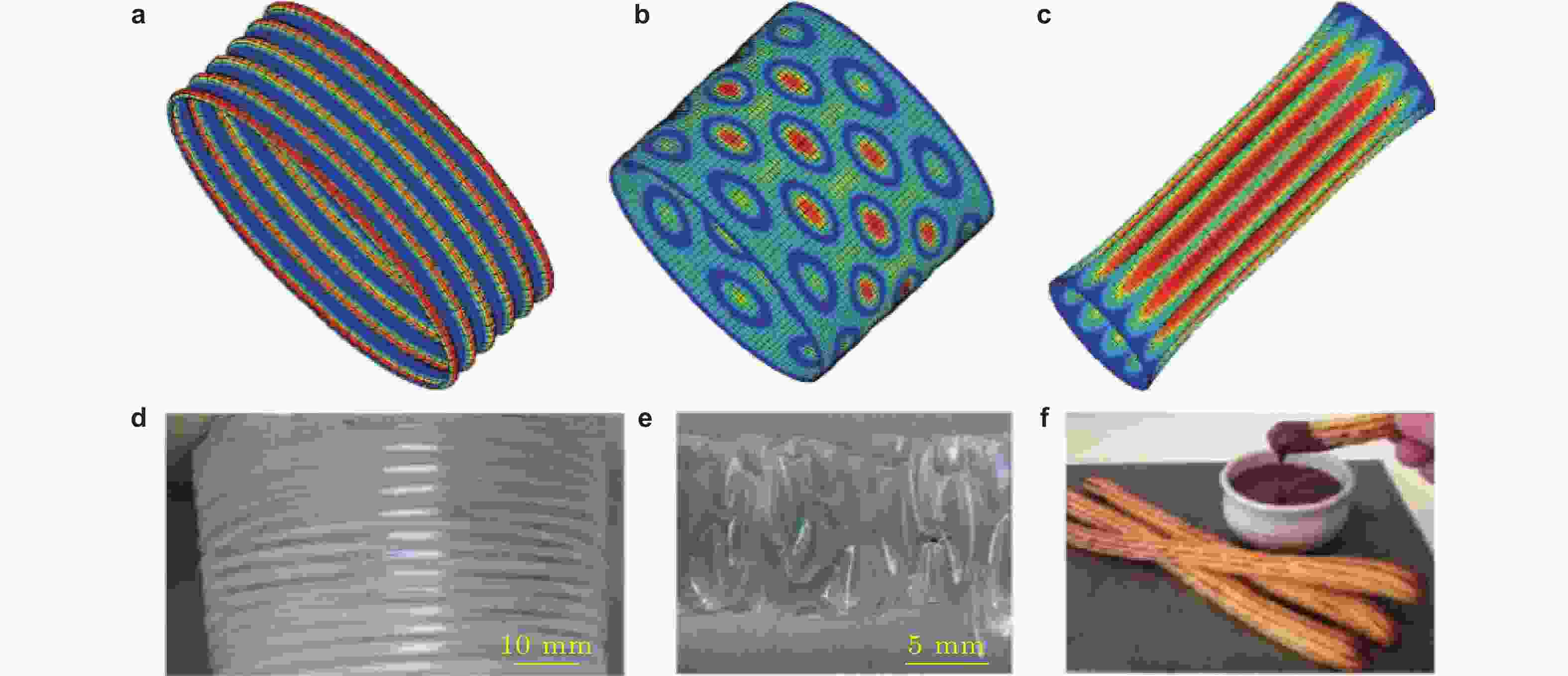
图 6 三种典型柱面核壳结构失稳形貌: (a)轴压下轴对称正弦形模态; (b)轴压下非轴对称钻石形模态; (c)热载荷下油条状失稳模态(Xu et al. 2017); (d)(e)为实验(Zhao et al. 2014)分别对应(a)(b)模态; (f)西班牙油条

下载: 全尺寸图片幻灯片

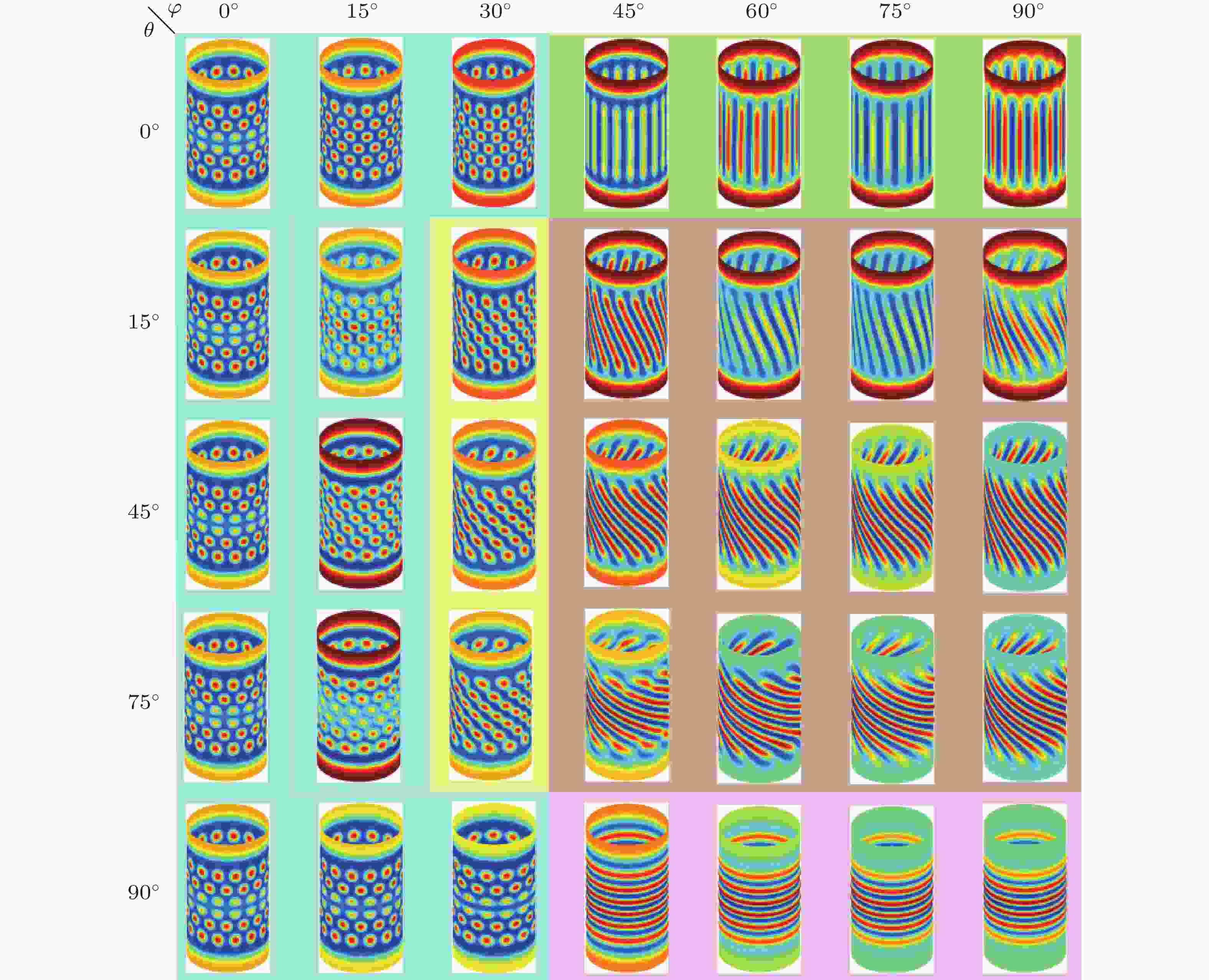
图 7 液晶高聚物网络(LCN)柱面核壳结构表面失稳形貌相图. 液晶分子指向矢取向显著影响褶皱失稳形貌选择(Zhao et al. 2021)

下载: 全尺寸图片幻灯片

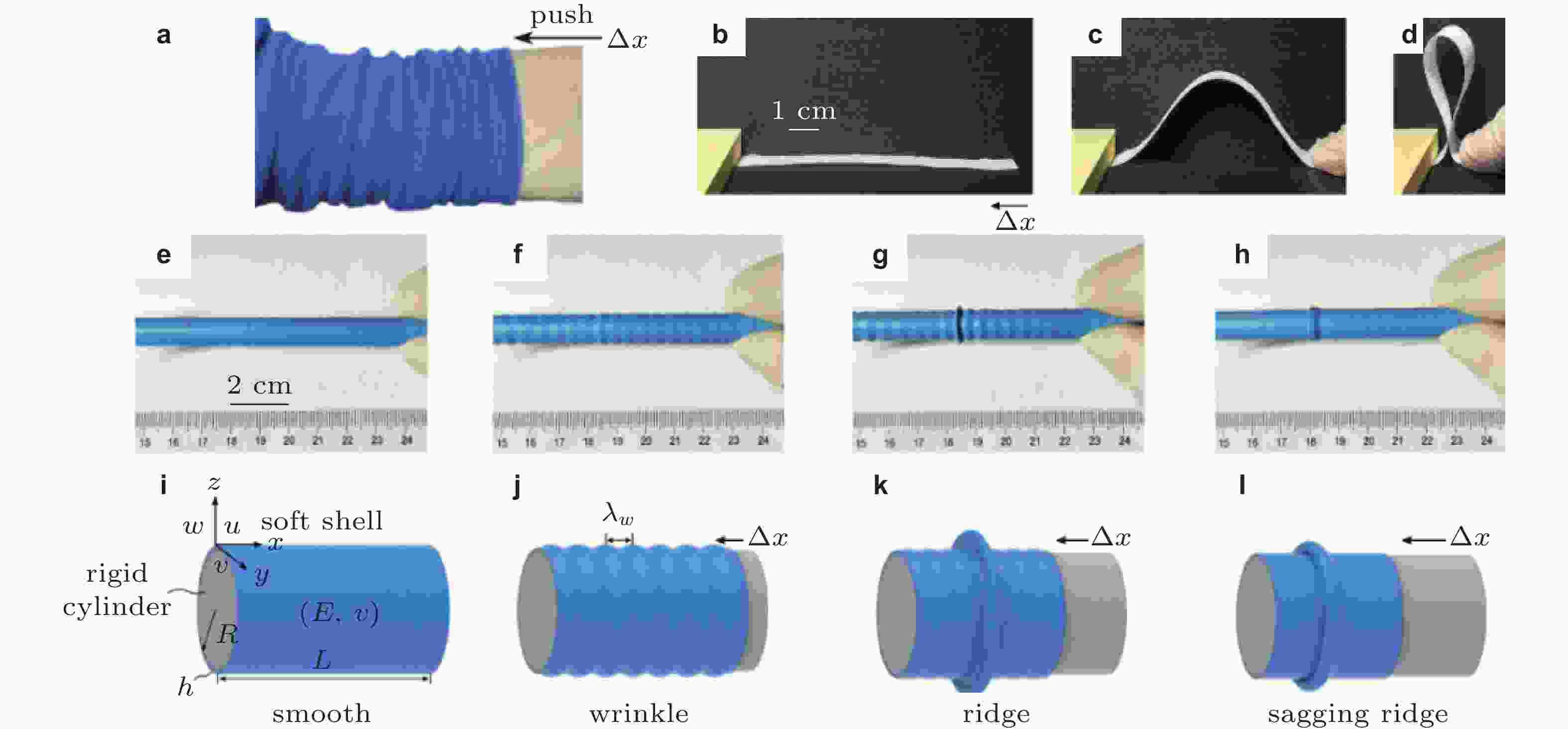
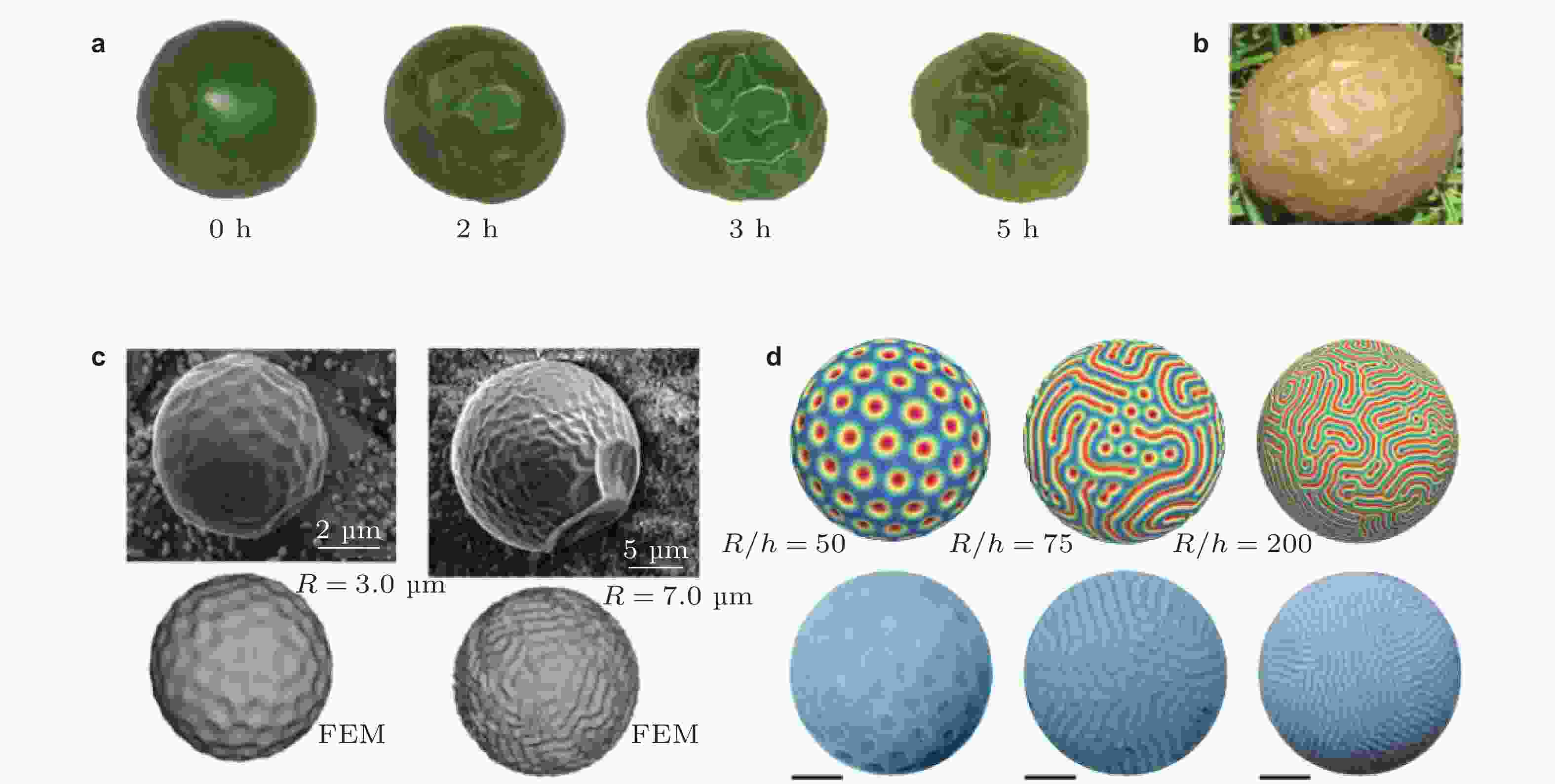
图 8 软壳在圆柱面上滑动受压失稳模态. (a)撸起的袖子上的褶皱. (b) ~ (d)单轴压缩桌面上的一张平坦的纸, 纸面隆起形成单一凸脊. (e)柱面乳胶气球厚度$h_{\rm{f}} = 0.2\;{\rm{mm}}$, 嵌套在半径$R = 3\;{\rm{mm}}$的亚克力圆柱上. 随着右侧压缩的增加, 出现四种不同的状态: (e)初始光滑构型; (f)正弦形褶皱; (g)凸脊; (h)松垂形凸脊. (i) ~ (l)分别对应于(e) ~ (h)的特征示意图(Yang et al. 2018)

下载: 全尺寸图片幻灯片

图 9 球面核壳结构表面失稳是一种复杂的跨尺度力学行为: (a) (b)植物果实失水皱缩形貌(Li et al. 2011, Xu & Potier-Ferry 2017); (c) Ag核/SiO₂壳微球结构表面失稳形貌(Cao et al. 2008); (d)不同径厚比下球面核壳结构的失稳模态(Stoop et al. 2015)

下载: 全尺寸图片幻灯片

图 10 核壳结构起皱模态相图. 无量纲参数${C_{\rm{s}}}$决定了局部凹陷、巴基球、迷宫和棋盘之间的模态选择. 局部凹陷到巴基球的相变边界为${C_{\rm{s}}} \thickapprox 1.3$, 巴基球到迷宫的相变边界为${C_s}\thickapprox 15$ (Xu et al. 2020b)

下载: 全尺寸图片幻灯片

参考文献(111)

[1]	曹进军, 张卉婷, 张亮, 彭福军, 恽卫东. 2019. 对角受拉方膜褶皱变形幅值的理论预测及实验验证. 力学学报, 51: 1403-1410 (Cao J J, Zhang H T, Zhang L, Peng F J, Yun W D. 2019. Theoretical prediction and experimental verification of wrinkle amplitude in a square membrane subjected to diagonal tension. Chinese Journal of Theoretical and Applied Mechanics, 51: 1403-1410). doi: 10.6052/0459-1879-19-109
[2]	冯西桥, 曹艳平, 李博. 2017. 软材料表面失稳力学. 北京: 科学出版社 Feng X Q, Cao Y P, Li B. 2017. Surface Wrinkling Mechanics of Soft Materials. Beijing: Science Press
[3]	倪勇, 刘佩琳, 马龙, 李世琛, 何陵辉. 2018. 基底上薄膜结构的非线性屈曲力学进展. 固体力学学报, 39: 113-138 (Ni Y, Liu P L, Ma L, Li S C, He L H. 2018. Nonlinear buckling mechanics of film-substrate systems: Recent progress. Chinese Journal of Solid Mechanics, 39: 113-138).
[4]	胡海岩. 2016. 太阳帆航天器的关键技术. 深空探测学报, 3: 334-344 (Hu H Y. 2016. Key technologies of solar sail spacecraft. Journal of Deep Space Exploration, 3: 334-344).
[5]	彭福军, 谢超, 张良俊. 2017. 面向空间应用的薄膜可展开结构研究进展及技术挑战. 载人航天, 23: 427-439 (Peng F J, Xie C, Zhang L J. 2017. Advancement and technical challenges of deployable membrane structure in space application. Manned Spaceflight, 23: 427-439). doi: 10.3969/j.issn.1674-5825.2017.04.001
[6]	杜星文, 王长国, 万志敏. 2006. 空间薄膜结构的褶皱研究进展. 力学进展, 36: 187-199 (Du X W, Wang C G, Wan Z M. 2006. Advances of the study on wrinkles of space membrane sturcures. Advances in Mechanics, 36: 187-199).
[7]	王长国, 杜星文, 万志敏. 2007. 空间薄膜结构褶皱的数值模拟最新研究进展. 力学进展, 37: 389-397 (Wang C G, Du X W, Wan Z M. 2007. Advances in the numerical investigations on wrinkles in space membrane structures. Advances in Mechanics, 37: 389-397).
[8]	Allen H G. 1969. Analysis and Design of Structural Sandwich Panels. New York: Pergamon Press.
[9]	Audoly B, Boudaoud A. 2008. Buckling of a stiff film bound to a compliant substrate—Part I: formulation, linear stability of cylindrical patterns, secondary bifurcations. Journal of the Mechanics and Physics of Solids, 56: 2401-2421. doi: 10.1016/j.jmps.2008.03.003
[10]	Auguste A, Yang J, Jin L, Chen D, Suo Z, Hayward R C. 2018. Formation of high aspect ratio wrinkles and ridges on elastic bilayers with small thickness contrast. Soft Matter, 14: 8545-8551. doi: 10.1039/C8SM01345D
[11]	Bao W, Miao F, Chen Z, Zhang H, Jang W, Dames C, Lau C N. 2009. Controlled ripple texturing of suspended graphene and ultrathin graphite membranes. Nature Nanotechnology, 4: 562-566. doi: 10.1038/nnano.2009.191
[12]	Bažant Z P. 2000. Structural stability. International Journal of Solids and Structures, 37: 55-67. doi: 10.1016/S0020-7683(99)00078-5
[13]	Ben Amar M, Jia F. 2013. Anisotropic growth shapes intestinal tissues during embryogenesis. Proceedings of the National Academy of Sciences of the United States of America, 110: 10525-10530. doi: 10.1073/pnas.1217391110
[14]	Bende N P, Evans A A, Innes-Gold S, Marin L A, Cohen I, Hayward R C, Santangelo C D. 2015. Geometrically controlled snapping transitions in shells with curved creases. Proceedings of the National Academy of Sciences of the United States of America, 112: 11175-11180. doi: 10.1073/pnas.1509228112
[15]	Biot M A. 1963. Surface instability of rubber in compression. Applied Scientific Research, 12: 168-182. doi: 10.1007/BF03184638
[16]	Bowden N, Brittain S, Evans A G, Hutchinson J W, Whitesides G W. 1998. Spontaneous formation of ordered structures in thin films of metals supported on an elastomeric polymer. Nature, 393: 146-149. doi: 10.1038/30193
[17]	Brau F, Vandeparre H, Sabbah A, Poulard C, Boudaoud A, Damman P. 2011. Multiple-lengthscale elastic instability mimics parametric resonance of nonlinear oscillators. Nature Physics, 7: 56-60. doi: 10.1038/nphys1806
[18]	Breid D, Crosby A J. 2013. Curvature-controlled wrinkle morphologies. Soft Matter, 9: 3624-3630. doi: 10.1039/c3sm27331h
[19]	Budday S, Andres S, Steinmann P, Kuhl E. 2015b. Primary and secondary instabilities in soft bilayered systems. Proceedings in Applied Mathematics and Mechanics, 15: 281-282. doi: 10.1002/pamm.201510131
[20]	Budday S, Kuhl E, Hutchinson J W. 2015a. Period-doubling and period-tripling in growing bilayered systems. Philosophical Magazine, 95: 3208-3224. doi: 10.1080/14786435.2015.1014443
[21]	Budday S, Steinmann P, Goriely A, Kuhl E. 2015. Size and curvature regulate pattern selection in the mammalian brain. Extreme Mechanics Letter, 4: 193-198. doi: 10.1016/j.eml.2015.07.004
[22]	Cai S, Breid D, Crosby A J, Suo Z, Hutchinson J W. 2011. Periodic patterns and energy states of buckled films on compliant substrates. Journal of the Mechanics Physics of Solids, 59: 1094-1114. doi: 10.1016/j.jmps.2011.02.001
[23]	Cao G, Chen X, Li C, Ji A, Cao Z. 2008. Self-assembled triangular and labyrinth buckling patterns of thin films on spherical substrates. Physical Review Letters, 100: 036102-1-036102-4.
[24]	Cao Y, Hutchinson J W. 2012. Wrinkling phenomena in neo-Hookean film/substrate bilayers. Journal of Applied Mechanics, 79: 031019-1-031019-9.
[25]	Cerda E, Mahadevan L. 2003. Geometry and physics of wrinkling. Physical Review Letters, 90: 074302-1-074302-4.
[26]	Cerda E, Ravi-Chandar K, Mahadevan L. 2002. Wrinkling of an elastic sheet under tension. Nature, 419: 579-580.
[27]	Chen P Y, Liu M, Wang Z, Hurt R H, Wong I Y. 2017. From flatland to space land: higher dimensional patterning with two-dimensional materials. Advanced Materials, 29: 1605096-1-1605096-31.
[28]	Chen X, Hutchinson J W. 2004. Herringbone buckling patterns of compressed thin films on compliant substrates. Journal of Applied Mechanics, 71: 597-603. doi: 10.1115/1.1756141
[29]	Chen X, Yin J. 2010. Buckling patterns of thin films on curved compliant substrates with applications to morphogenesis and three-dimensional micro-fabrication. Soft Matter, 6: 5667-5680. doi: 10.1039/c0sm00401d
[30]	Cheng Z, Xu F. 2021. Intricate evolutions of multiple-period post-buckling patterns in bilayers, Science China: Physics. Mechanics & Astronomy, 64: 214611-1-214611-10.
[31]	Chung J Y, Nolte A J, Stafford C M. 2011. Surface wrinkling: A versatile platform for measuring thin-film properties. Advanced Materials, 23: 349-368. doi: 10.1002/adma.201001759
[32]	Ding M, Xu F, Wang T, Fu C. 2021. Nanosleeves: Morphology transitions of infilled carbon nanotubes. Journal of the Mechanics and Physics of Solids, 152: 104398-1-104398-19.
[33]	Efimenko K, Rackaitis M, Manias E, Vaziri A, Mahadevan L, Genzer J. 2005. Nested self-similar wrinkling patterns in skins. Nature Materials, 4: 293-297. doi: 10.1038/nmat1342
[34]	Friedl N, Rammerstorfer F G, Fischer F D. 2000. Buckling of stretched strips. Computers and Structures, 78: 185-190.
[35]	Fu C, Wang T, Xu F, Huo Y, Potier-Ferry M. 2019. A modeling and resolution framework for wrinkling in hyperelastic sheets at finite membrane strain. Journal of the Mechanics and Physics of Solids, 124: 446-470. doi: 10.1016/j.jmps.2018.11.005
[36]	Fu C, Xu F, Huo Y. 2018. Photo-controlled patterned wrinkling of liquid crystalline polymer films on compliant substrates. International Journal of Solids and Structures, 132-133: 264-277. doi: 10.1016/j.ijsolstr.2017.10.018
[37]	Genzer J, Groenewold J. 2006. Soft matter with hard skin: from skin wrinkles to templating and material characterization. Soft Matter, 2: 310-323. doi: 10.1039/b516741h
[38]	Healey T J, Li Q, Cheng R B. 2013. Wrinkling behavior of highly stretched rectangular elastic films via parametric global bifurcation. Journal of Nonlinear Science, 23: 777-805. doi: 10.1007/s00332-013-9168-3
[39]	Hu X, Dou Y, Li J, Liu Z. 2019. Buckled structures: fabrication and applications in wearable electronics. Small, 15: 1804805-1-1804805-26.
[40]	Huang Z Y, Hong W, Suo Z. 2005. Nonlinear analyses of wrinkles in a film bonded to a compliant substrate. Journal of the Mechanics and Physics of Solids, 53: 2101-2118. doi: 10.1016/j.jmps.2005.03.007
[41]	Irvine W T M, Vitelli V, Chaikin P M. 2010. Pleats in crystals on curved surfaces. Nature, 468: 947-951. doi: 10.1038/nature09620
[42]	Janssens S D, Sutisna B, Giussani A, Vázquez-Cortés D, Fried E. 2020. Boundary curvature effect on the wrinkling of thin suspended films. Applied Physics Letters, 116: 193702-1-193702-5.
[43]	Jacques N, Potier-Ferry M. 2005. On mode localization in tensile plate buckling. Comptes Rendus Mecanique, 333: 804-809. doi: 10.1016/j.crme.2005.10.013
[44]	Jia F, Pearce S P, Goriely A. 2018. Curvature delays growth-induced wrinkling. Physical Review E, 98: 033003-1-033003-11.
[45]	Jiang H, Khang D Y, Song J, Sun Y, Huang Y, Rogers J A. 2007. Finite deformation mechanics in buckled thin films on compliant supports. Proceedings of the National Academy of Sciences of the United States of America, 104: 15607-15612. doi: 10.1073/pnas.0702927104
[46]	Jiang Y, Korpas L M, Raney J R. 2019. Bifurcation-based embodied logic and autonomous actuation. Nature Communications, 10: 128-1-128-10. doi: 10.1038/s41467-019-09322-7
[47]	Khang D Y, Jiang H, Huang Y, Rogers J A. 2006. A stretchable form of single-crystal silicon for high-performance electronics on rubber substrates. Science, 311: 208-212. doi: 10.1126/science.1121401
[48]	Kim T Y, Puntel E, Fried E. 2016. Numerical study of the wrinkling of a stretched thin sheet. International Journal of Solids and Structures, 49: 771-782.
[49]	Li B, Cao Y P, Feng X Q, Gao H. 2012. Mechanics of morphological instabilities and surface wrinkling in soft materials: A review. Soft Matter, 8: 5728-5745. doi: 10.1039/c2sm00011c
[50]	Li B, Jia F, Cao Y P, Feng X Q, Gao H. 2011. Surface wrinkling patterns on a core-shell soft sphere. Physical Review Letters, 106: 234301-1-234301-4.
[51]	Li Q, Han X, Hou J, Yin J, Jiang S, Lu C. 2015. Patterning poly (dimethylsiloxane) microspheres via combination of oxygen plasma exposure and solvent treatment. Journal of Physical Chemistry B, 119: 13450-13461.
[52]	Li Q, Healey T J. 2016. Stability boundaries for wrinkling in highly stretched elastic sheets. Journal of the Mechanics and Physics of Solids, 97: 260-274. doi: 10.1016/j.jmps.2015.12.001
[53]	Liang H, Mahadevan L. 2011. From the Cover: Growth, geometry, and mechanics of a blooming lily. Proceedings of the National Academy of Sciences of the United States of America, 108: 5516-5521. doi: 10.1073/pnas.1007808108
[54]	Liu F, Xu F, Fu C. 2019. Orientable wrinkles in stretched orthotropic films. Extreme Mechanics Letters, 33: 100579-1-100579-9.
[55]	López Jiménez F, Stoop N, Lagrange R, Dunkel J, Reis P M. 2016. Curvature-controlled defect localization in elastic surface crystals. Physical Review Letter, 116: 104301-1-104301-5.
[56]	Luo Y, Xing J, Kang Z, Zhan J, Li M. 2020. Uncertainty of membrane wrinkling behaviors considering initial thickness imperfections. International Journal of Solids and Structures, 191-192: 264-277. doi: 10.1016/j.ijsolstr.2020.01.022
[57]	Luo Y, Xing J, Niu Y, Li M, Kang Z. 2017. Wrinkle-free design of thin membrane structures using stress-based topology optimization. Journal of the Mechanics and Physics of Solids, 102: 277-293. doi: 10.1016/j.jmps.2017.02.003
[58]	Ma L, Liu X, Soh A K, He L, Wu C, Ni Y. 2019. Growth of curved crystals: competition between topological defect nucleation and boundary branching. Soft Matter, 15: 4391-4400. doi: 10.1039/C9SM00507B
[59]	Mitchell N P, Koning V, Vitelli V, Irvine W T M. 2017. Fracture in sheets draped on curved surfaces. Nature Materials, 16: 89-93. doi: 10.1038/nmat4733
[60]	Ohzono T, Monobe H. 2012. Microwrinkles: shape-tunability and applications. Journal of Colloid and Interface Science, 368: 1-8. doi: 10.1016/j.jcis.2011.11.075
[61]	Paulsen J D, Hohlfeld E, King H, Huang J, Qiu Z, Russell T P, Menon N, Vella D, Davidovitch B. 2016. Curvature-induced stiffness and the spatial variation of wavelength in wrinkled sheets. Proceedings of the National Academy of Sciences of the United States of America, 113: 1144-1149. doi: 10.1073/pnas.1521520113
[62]	Pezzulla M, Stoop N, Steranka M P, Bade A J, Holmes D P. 2018. Curvature-induced instabilities of shells. Physical Review Letters, 120: 048002-1-048002-5.
[63]	Pikul J H, Li S, Bai H, Hanlon R T, Cohen I, Shepherd R F. 2017. Stretchable surfaces with programmable 3D texture morphing for synthetic camouflaging skins. Science, 358: 210-214. doi: 10.1126/science.aan5627
[64]	Pocivavsek L, Dellsy R, Kern A, Johnson S, Lin B, Lee K Y C, Cerda E. 2008. Stress and fold localization in thin elastic membranes. Science, 320: 912-916. doi: 10.1126/science.1154069
[65]	Pocivavsek L, Pugar J, O'Dea R, Ye S H, Wagner W, Tzeng E, Velankar S, Cerda E. 2018. Topography-driven surface renewal. Nature Physics, 14: 948-953. doi: 10.1038/s41567-018-0193-x
[66]	Poncharal P, Wang Z L, Ugarte D, de Heer W A. 1999. Electrostatic deflections and electromechanical resonances of carbon nanotubes. Science, 283: 1513-1516. doi: 10.1126/science.283.5407.1513
[67]	Puntel E, Deseri L, Fried E. 2011. Wrinkling of a stretched thin sheet. Journal of Elasticity, 105: 137-170. doi: 10.1007/s10659-010-9290-5
[68]	Rafsanjani A, Jin L, Deng B, Bertoldi K. 2019. Propagation of pop ups in kirigami shells. Proceedings of the National Academy of Sciences of the United States of America, 116: 8200-8205. doi: 10.1073/pnas.1817763116
[69]	Reis P M, Brau F, Damman P. 2018. The mechanics of slender structures. Nature Physics, 14: 1150-1151. doi: 10.1038/s41567-018-0369-4
[70]	Reis P M. 2015. A perspective on the revival of structural (in) stability with novel opportunities for function: From buckliphobia to buckliphilia. Journal of Applied Mechanics, 82: 111001-1-111001-4.
[71]	Rodríguez-Hernández J. 2015. Wrinkled interfaces: taking advantage of surface instabilities to pattern polymer surfaces. Progress in Polymer Science, 42: 1-41. doi: 10.1016/j.progpolymsci.2014.07.008
[72]	Siéfert E, Reyssat E, Bico J, Roman B. 2019. Bio-inspired pneumatic shape-morphing elastomers. Nature Materials, 18: 24-28. doi: 10.1038/s41563-018-0219-x
[73]	Sipos A A, Fehér E. 2016. Disappearance of stretch-induced wrinkles of thin sheets: a study of orthotropic film. International Journal of Solids and Structures, 97-98: 275-283. doi: 10.1016/j.ijsolstr.2016.07.021
[74]	Song J, Jiang H, Huang Y, Rogers J A. 2009. Mechanics of stretchable inorganic electronic materials. Journal of Vacuum Science & Technology A, 27: 1107-1125.
[75]	Stoop N, Lagrange R, Terwagne D, Reis P M, Dunkel J. 2015. Curvature-induced symmetry breaking determines elastic surface patterns. Nature Materials, 14: 337-342. doi: 10.1038/nmat4202
[76]	Sultan E, Boudaoud A. 2008. The buckling of a swollen thin gel layer bound to a compliant substrate. Journal of Applied Mechanics, 75: 051002-1-051002-5.
[77]	Sun J Y, Xia S, Moon M W, Oh K H, Kim K S. 2012. Folding wrinkles of a thin stiff layer on a soft substrate. Proceedings of The Royal Society A: Mathematical Physical and Engineering Sciences, 468: 932-953.
[78]	Tallinen T, Chung J Y, Rousseau F, Girard N, Lefèvre J, Mahadevan L. 2016. On the growth and form of cortical convolutions. Nature Physics, 12: 588-593. doi: 10.1038/nphys3632
[79]	Tan Y, Yan J, Chu Z. 2019. Thermal-shrinking-induced ringpatterned boron nitride wrinkles on carbon fibers. Carbon, 152: 532-536. doi: 10.1016/j.carbon.2019.06.058
[80]	Taylor, M, Shirani, M, Dabiri, Y, Guccione, J M, Steigmann, D J. 2019. Finite elastic wrinkling deformations of incompressible fiber-reinforced plates. International Journal of Engineering Science, 144: 103138-1-103138-21.
[81]	Terwagne D, Brojan M, Reis P M. 2014. Smart morphable surfaces for aerodynamic drag control. Advanced Materials, 26: 6608-6611. doi: 10.1002/adma.201401403
[82]	Vandeparre H, Piñeirua M, Brau F, Roman B, Bico J, Gay C, Bao W, Lau C N, Reis P M, Damman P. 2011. Wrinkling hierarchy in constrained thin sheets from suspended graphene to curtains. Physical Review Letters, 106: 224301-1-224301-4.
[83]	van der Heijden A M A. 2009. W.T. Koiter's Elastic Stability of Solids and Structures. Cambridge: Cambridge University Press.
[84]	Wang C G, Liu Y P, Lan L, Li L, Tan H F. 2016. Post-wrinkling analysis of a torsionally sheared annular thin film by using a compound series method. International Journal of Mechanical Sciences, 110: 22-33. doi: 10.1016/j.ijmecsci.2016.02.011
[85]	Wang T, Fu C, Xu F, Huo Y, Potier-Ferry M. 2019. On the wrinkling and restabilization of highly stretched sheets. International Journal of Engineering Science, 136: 1-16. doi: 10.1016/j.ijengsci.2018.12.002
[86]	Wang T, Yang Y, Fu C, Liu F, Wang K, Xu F. 2020. Wrinkling and smoothing of a soft shell. Journal of the Mechanics and Physics of Solids, 134: 103738-1-103738-20.
[87]	Xu F, Abdelmoula R, Potier-Ferry M. 2017. On the buckling and post-buckling of core-shell cylinders under thermal loading. International Journal of Solids and Structures, 126-127: 17-36. doi: 10.1016/j.ijsolstr.2017.07.024
[88]	Xu F, Koustawa Y, Potier-Ferry M, Belouettar S. 2015. Instabilities in thin films on hyperelastic substrates by 3D finite elements. International Journal of Solids and Structures, 69-70: 71-85. doi: 10.1016/j.ijsolstr.2015.06.007
[89]	Xu F, Potier-Ferry M. 2017. Quantitative predictions of diverse wrinkling patterns in film/substrate systems. Scientific Reports, 7: 18081-1-18081-10.
[90]	Xu F, Potier-Ferry M, Belouettar S, Cong Y. 2014. 3D finite element modeling for instabilities in thin films on soft substrates. International Journal of Solids and Structures, 51: 3619-3632. doi: 10.1016/j.ijsolstr.2014.06.023
[91]	Xu F, Potier-Ferry M. 2016. On axisymmetric/diamond-like mode transitions in axially compressed core-shell cylinders. Journal of the Mechanics and Physics of Solids, 94: 68-87. doi: 10.1016/j.jmps.2016.04.025
[92]	Xu F, Fu C, Yang Y. 2020a. Water affects morphogenesis of growing aquatic plant leaves. Physical Review Letters, 124: 038003-1-038003-6.
[93]	Xu F, Zhao S, Lu C, Potier-Ferry M. 2020b. Pattern selection in core-shell spheres. Journal of the Mechanics and Physics of Solids, 137: 103892-1-103892-14.
[94]	Xu F, Zhao S. 2020. Thermal wrinkling of liquid crystal polymer shell/core spheres. Extreme Mechanics Letters, 40: 100860-1-100860-11.
[95]	Yamaki N. 1984. Elastic Stability of Circular Cylindrical Shells. North Holland, Amsterdam.
[96]	Yan D, Chang J, Zhang H, Liu J, Song H, Xue Z, Zhang F, Zhang Y. 2020. Soft three-dimensional network materials with rational bio-mimetic designs. Nature Communications, 11: 1180-1-1180-11.
[97]	Yan D, Zhang K, Peng F, Hu G. 2014. Tailoring the wrinkle pattern of a microstructured membrane. Applied Physics Letters, 105: 071905-1-071905-4.
[98]	Yang X, Zhao Y, Xie J, Han X, Wang J, Zong C, Ji H, Zhao J, Jiang S, Cao Y, Lu C. 2016. Bioinspired fabrication of free-standing conducting films with hierarchical surface wrinkling patterns. ACS Nano, 10: 3801-3808. doi: 10.1021/acsnano.6b00509
[99]	Yang Y, Dai H H, Xu F, Potier-Ferry M. 2018. Pattern transitions in a soft cylindrical shell. Physical Review Letters, 120: 215503-1-215503-5.
[100]	Yang Y, Fu C, Xu F. 2020. A finite strain model predicts oblique wrinkles in stretched anisotropic films. International Journal of Engineering Science, 155: 103354-1-103354-14.
[101]	Yin J, Chen X, Sheinman I. 2009. Anisotropic buckling patterns in spheroidal film/substrate systems and their implications in some natural and biological systems. Journal of the Mechanics and Physics of Solids, 57: 1470-1484. doi: 10.1016/j.jmps.2009.06.002
[102]	Yin J, Han X, Cao Y, Lu C. 2014. Surface wrinkling on polydimethylsiloxane microspheres via wet surface chemical oxidation. Scientific Reports, 4: 5710-1-5710-8.
[103]	Yuan H, Wu K, Zhang J, Wang Y, Liu G, Sun J. 2019. Curvature-controlled wrinkling surfaces for friction. Advanced Materials, 31: 1900933-1-1900933-6.
[104]	Zhang C, Hao Y K, Li B, Feng X Q, Gao H. 2018. Wrinkling patterns in soft shells. Soft Matter, 14: 1681-1688. doi: 10.1039/C7SM02261A
[105]	Zhao S, Xu F, Fu C, Huo Y. 2019. Controllable wrinkling patterns on liquid crystal polymer film/substrate systems by laser illumination. Extreme Mechanics Letters, 30: 100502-1-100502-12.
[106]	Zhao S, Xu F, Fu C, Huo Y. 2021. Oblique wrinkling patterns on liquid crystal polymer core-shell cylinders under thermal load. International Journal of Solids and Structures, 208-209: 181-193. doi: 10.1016/j.ijsolstr.2020.11.005
[107]	Zhao Y, Cao Y, Feng X Q, Ma K. 2014. Axial compression-induced wrinkles on a core-shell soft cylinder: Theoretical analysis, simulations and experiments. Journal of the Mechanics and Physics of Solids, 73: 212-227. doi: 10.1016/j.jmps.2014.09.005
[108]	Zhao Y, Han X, Li G, Lu C, Cao Y, Feng X Q, Gao H. 2015. Effect of lateral dimension on the surface wrinkling of a thin film on compliant substrate induced by differential growth/swelling. Journal of the Mechanics and Physics of Solids, 83: 129-145. doi: 10.1016/j.jmps.2015.06.003
[109]	Zhao Y, Zhu H, Jiang C, Cao Y, Feng X Q. 2020. Wrinkling pattern evolution on curved surfaces. Journal of the Mechanics and Physics of Solids, 135: 103798-1-103798-15.
[110]	Zheng L. 2009. Wrinkling of dielectric elastomer membranes. [PhD Thesis] Pasadena, USA: California Institute of Technology.
[111]	Zhu J, Zhang X, Wierzbicki T. 2018. Stretch-induced wrinkling of highly orthotropic thin film. International Journal of Solids and Structures, 139-140: 238-249. doi: 10.1016/j.ijsolstr.2018.02.005