-
摘要: 随着力学研究不断向多学科交叉、跨尺度关联及极端环境影响推进, 强非线性、强间断、多场强耦合、多尺度与复杂几何构型已成为解决各类力学问题定量分析所面临的共性特征. 长期的定量研究表明, 实现具有这类特征力学问题有效求解的核心之一, 是构建在多尺度情形、非线性因素作用下具有准确识别、定位、捕获以及分离各个尺度特征尤其是小尺度局部特征能力的数值工具, 这些能力包括大尺度低阶近似解与小尺度高阶微小截断误差的有效分离解耦. 而小波理论固有的多分辨分析和时频局部化特性, 以及丰富的基函数可选择性, 恰好能满足这一数学特征需求, 因此, 可为发展各类复杂力学问题的高效定量求解方法提供有效的理论支撑和丰富多样的技术途径. 基于这一事实, 本文对小波理论进行了全面探讨, 着重介绍了双正交多分辨分析的理论框架和常用小波基的构造方法. 在此基础上, 深入研究了有限区域上小波逼近格式的构建方法, 并系统阐述了各类基于小波理论的数值方法的基本原理、发展历程及其优缺点. 特别关注了近期出现的具有突出性能的几类新型小波方法, 并详细评述了它们在典型力学问题求解中的应用效果. 同时, 本文也指出了当前小波方法在复杂强非线性力学问题求解中所面临的挑战, 旨在为小波数值方法的未来发展及其在复杂力学与工程问题中的应用拓展提供有益的参考, 并为最终实现这些问题的高效、高精度普适定量求解提供新的视角和方法.Abstract: Mechanics research advances towards interdisciplinary research, cross-scale correlations, and extreme environmental impacts. Strong nonlinearity, strong discontinuity, significant multi-physics coupling, multiscale, and complex geometries have become common characteristics in solving various mechanics problems quantitatively. Long-term quantitative research indicates that one of the core ingredients for solving such problems effectively lies in constructing numerical methods that can accurately identify, locate, capture, and separate different scale characteristics especially small-scale local characteristics, under multiscale and nonlinearity circumstances. These numerical methods should also possess the capacity to isolate and decouple the large-scale lower-order approximation from the small-scale higher-order truncation error effectively. The intrinsic multiresolution analysis and time-frequency localization characteristics of wavelet theory, as well as the various selectivity of basis functions, align precisely with the demands of this mathematical feature. Therefore, they can provide fundamental theory and diverse approaches for developing efficient quantitative methods to address various complex mechanics problems. Based on this fact, this paper provides a comprehensive discussion of wavelet theory, focusing on the theoretical framework of biorthogonal multi-resolution analysis and the construction method of frequently used wavelet bases. Furthermore, wavelet approximation of the function defined on a finite domain is presented in detail. The fundamental principles, development, merits, and shortcomings of different wavelet-based numerical methods are systematically elucidated. Several novel wavelet-based methods with outstanding performance developed recently are elaborated especially, and their applications in solving typical mechanics problems are reviewed. Meanwhile, the present paper also points out challenges encountered by the existing wavelet-based numerical methods when addressing complex and strongly nonlinear mechanics problems. This may provide valuable references for the development of wavelet-based numerical methods and their applications in complex mechanics and engineering problems, and introduce new perspectives and methods for solving these problems efficiently, accurately, and universally.
-
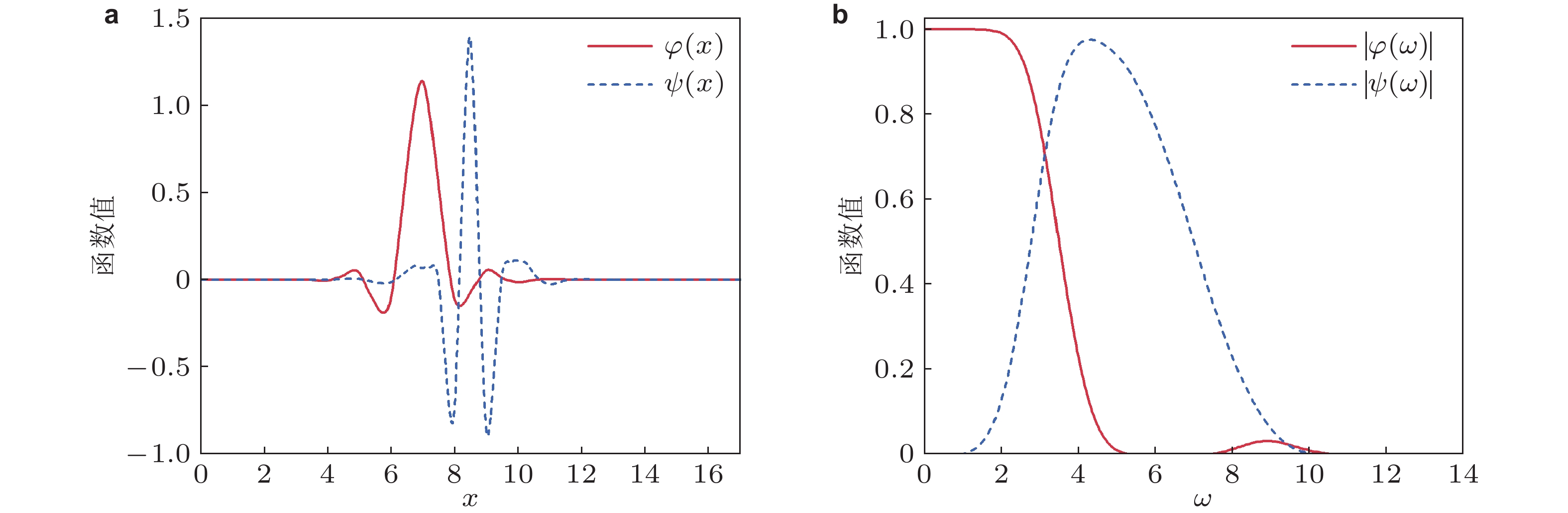
[1] 安效民, 徐敏, 陈士橹. 2009. 多场耦合求解非线性气动弹性的研究综述. 力学进展, 39: 284-298 (An X M, Xu M, Chen S L. 2009. An overview of CFD/CSD coupled solution for nonlinear aeroelasticity. Advances in Mechanics, 39: 284-298).An X M, Xu M, Chen S L. 2009. An overview of CFD/CSD coupled solution for nonlinear aeroelasticity. Advances in Mechanics, 39: 284-298. [2] 顾云风, 周又和. 2000. 简支梁式板的压电动力控制与数值仿真. 兰州大学学报: 自然科学版, 36: 20-24 (Gu Y F, Zhou Y H. 2000. Dynamic control of simply supported beam plates with piezoelectric layers and its numerical simulations. Journal of Lanzhou University(Natural Sciences), 36: 20-24).Gu Y F, Zhou Y H. 2000. Dynamic control of simply supported beam plates with piezoelectric layers and its numerical simulations. Journal of Lanzhou University(Natural Sciences), 36: 20-24. [3] 刘小靖, 周又和, 王记增. 2022. 小波方法及其力学应用研究进展. 应用数学和力学, 43: 1-13 (Liu X J, Zhou Y H, Wang J Z. 2022. Research progresses of wavelet methods and their applications in mechanics. Applied Mathematics and Mechanics, 43: 1-13). doi: 10.1007/s10483-021-2795-5Liu X J, Zhou Y H, Wang J Z. 2022. Research progresses of wavelet methods and their applications in mechanics. Applied Mathematics and Mechanics, 43: 1-13. doi: 10.1007/s10483-021-2795-5 [4] 司洪伟, 李东旭. 2003. 小波理论在大型空间智能结构振动控制中的应用. 国防科技大学学报, 25: 14-18 (Si H W, Li D X. 2003. Researches on wavelet-based vibration control of large space smart structures. Journal of National University of Defense Technology, 25: 14-18). doi: 10.3969/j.issn.1001-2486.2003.02.004Si H W, Li D X. 2003. Researches on wavelet-based vibration control of large space smart structures. Journal of National University of Defense Technology, 25: 14-18. doi: 10.3969/j.issn.1001-2486.2003.02.004 [5] 王记增. 2001. 正交小波统一理论与方法及其在压电智能结构等力学研究中的应用. 兰州: 兰州大学 (Wang J Z. 2001. Generalized theory and arithmetic of orthogonal wavelets and applications to researches of mechanics including piezoelectric smart structures. Lanzhou University).Wang J Z. 2001. Generalized theory and arithmetic of orthogonal wavelets and applications to researches of mechanics including piezoelectric smart structures. Lanzhou University. [6] 王记增, 王晓敏, 周又和. 2010. 基于正交小波尺度函数展开的强非线性微分方程求解. 兰州大学学报: 自然科学版, 46: 96-101 (Wang J Z, Wang X M, Zhou Y H. 2010. Numerical solutions to differential equations with strong nonlinearities based on series expansion of orthogonal scaling functions. Journal of Lanzhou University(Natural Sciences), 46: 96-101).Wang J Z, Wang X M, Zhou Y H. 2010. Numerical solutions to differential equations with strong nonlinearities based on series expansion of orthogonal scaling functions. Journal of Lanzhou University(Natural Sciences), 46: 96-101. [7] 王记增, 周又和. 1998. 广义小波高斯积分法的误差估计. 兰州大学学报: 自然科学版, 34: 26-30 (Wang J Z, Zhou Y H. 1998. An error estimation of generalized Gaussian integral method in wavelet theory. Journal of Lanzhou University(Natural Sciences), 34: 26-30).Wang J Z, Zhou Y H. 1998. An error estimation of generalized Gaussian integral method in wavelet theory. Journal of Lanzhou University(Natural Sciences), 34: 26-30. [8] 翁炯. 2023. 非线性波动问题求解的小波方法及其在黏弹性结构中的应用. 兰州: 兰州大学 (Weng J. 2023. A wavelet method for solving nonlinear wave problems and its application in viscoelastic structures. Lanzhou University).Weng J. 2023. A wavelet method for solving nonlinear wave problems and its application in viscoelastic structures. Lanzhou University. [9] 徐聪. 2020. 复杂区域强非线性力学问题求解的小波方法. 兰州: 兰州大学 (Xu C. 2020. Wavelet methods for highly nonlinear problems of mechanics in complex geometry. Lanzhou University).Xu C. 2020. Wavelet methods for highly nonlinear problems of mechanics in complex geometry. Lanzhou University. [10] 轩建平, 郑锋. 2014. 基于Coiflet的二维小波有限元构造与应用. 华中科技大学学报(自然科学版), 42: 21-24 (Xuan J P, Zheng F. 2014. Construction and application of two-dimensional wavelet finite element based on Coiflet. Journal of Huazhong University of Science and Technology (Natural Science Edition), 42: 21-24).Xuan J P, Zheng F. 2014. Construction and application of two-dimensional wavelet finite element based on Coiflet. Journal of Huazhong University of Science and Technology (Natural Science Edition), 42: 21-24. [11] 张磊. 2016. 高精度小波数值方法及其在结构非线性分析中的应用. 兰州大学 (Zhang L. 2016. High-precision wavelet numerical methods and applications to nonlinear structural analysis. Lanzhou University).Zhang L. 2016. High-precision wavelet numerical methods and applications to nonlinear structural analysis. Lanzhou University. [12] 周又和, 王记增. 1998. 基于小波理论的悬臂板压电动力控制模式. 力学学报, 30: 719-727 (Zhou Y H, Wang J Z. 1998. A dynamic control model of piezoelectric cantilevered beam-plate based on wavelet theory. Acta Mechanica Sinica, 30: 719-727).Zhou Y H, Wang J Z. 1998. A dynamic control model of piezoelectric cantilevered beam-plate based on wavelet theory. Acta Mechanica Sinica, 30: 719-727. [13] 周又和, 王记增. 1999. 小波尺度函数计算的广义高斯积分法及其应用. 数学物理学报, 19: 293-300 (Zhou Y H, Wang J Z. 1999. Generalized gaussian integral method for calculations of scaling function transform of wavelets and its application. Acta Mathematica Scientia, 19: 293-300). doi: 10.1016/S0252-9602(17)30509-XZhou Y H, Wang J Z. 1999. Generalized gaussian integral method for calculations of scaling function transform of wavelets and its application. Acta Mathematica Scientia, 19: 293-300. doi: 10.1016/S0252-9602(17)30509-X [14] 周又和, 王记增, 郑晓静. 2001. 一种基于小波尺度函数变换的 Laplace 反演方法. 数学物理学报, 21A: 86-93 (Zhou Y H, Wang J Z, Zheng X J. 2001. A numerical inversion of the Laplace transform by use of the scaling function transform of wavelet theory. Acta Mathematica Scientia, 21A: 86-93).Zhou Y H, Wang J Z, Zheng X J. 2001. A numerical inversion of the Laplace transform by use of the scaling function transform of wavelet theory. Acta Mathematica Scientia, 21A: 86-93. [15] Abdeljawad T, Amin R, Shah K, et al. 2020. Efficient sustainable algorithm for numerical solutions of systems of fractional order differential equations by Haar wavelet collocation method. Alexandria Engineering Journal, 59: 2391-2400. doi: 10.1016/j.aej.2020.02.035 [16] Alam J M, Kevlahan N K R, Vasilyev O V. 2006. Simultaneous space–time adaptive wavelet solution of nonlinear parabolic differential equations. Journal of Computational Physics, 214: 829-857. doi: 10.1016/j.jcp.2005.10.009 [17] Aldroubi A, Unser M. 1993. Families of multiresolution and wavelet spaces with optimal properties. Numerical Functional Analysis and Optimization, 14: 417-446. doi: 10.1080/01630569308816532 [18] Allen J B, Rabiner L R. 1977. A unified approach to short-time Fourier analysis and synthesis. Proceedings of the IEEE, 65: 1558-1564. doi: 10.1109/PROC.1977.10770 [19] Amaratunga K, Williams J R. 1993. Wavelet based Green's function approach to 2D PDEs. Engineering Computations, 10: 349-367. doi: 10.1108/eb023913 [20] Amin R, Shah K, Al-Mdallal Q M, et al. 2021. Efficient numerical algorithm for the solution of eight order boundary value problems by haar wavelet method. International Journal of Applied and Computational Mathematics, 7: 34. [21] Amin R, Shah K, Khan I, et al. 2020. Efficient numerical scheme for the solution of tenth order boundary value problems by the haar wavelet method. Mathematics, 8: 1874. doi: 10.3390/math8111874 [22] Bacry E, Mallat S, Papanicolaou G. 1992. A wavelet based space-time adaptive numerical method for partial differential equations. ESAIM: Mathematical Modelling and Numerical Analysis, 26: 793-834. doi: 10.1051/m2an/1992260707931 [23] Battle G. 1987. A block spin construction of ondelettes. Part I: lemarié functions. Communications in Mathematical Physics, 110: 601-615. doi: 10.1007/BF01205550 [24] Bertoluzza S. 1996. Adaptive wavelet collocation method for the solution of Burgers equation. Transport Theory and Statistical Physics, 25: 339-352. doi: 10.1080/00411459608220705 [25] Bertoluzza S, Naldi G. 1996. A wavelet collocation method for the numerical solution of partial differential equations. Applied and Computational Harmonic Analysis, 3: 1-9. doi: 10.1006/acha.1996.0001 [26] Beylkin G, Coifman R, Rokhlin V. 1991. Fast wavelet transforms and numerical algorithms I. Communications on Pure and Applied Mathematics, 44: 141-183. doi: 10.1002/cpa.3160440202 [27] Bihari B L, Harten A. 1995. Application of generalized wavelets: an adaptive multiresolution scheme. Journal of Computational and Applied Mathematics, 61: 275-321. doi: 10.1016/0377-0427(94)00070-1 [28] Bihari B L, Harten A. 1997. Multiresolution schemes for the numerical solution of 2-D conservation laws I. SIAM Journal on Scientific Computing, 18: 315-354. doi: 10.1137/S1064827594278848 [29] Bittner K, Urban K. 2007. On interpolatory divergence-free wavelets. Mathematics of Computation, 76: 903-929. [30] Brebbia C A, Telles J C F, Wrobel L C. 1984. Boundary element techniques: theory and applications in engineering. Springer Science & Business Media. [31] Burrus C S, Odegard I E. 1998. Coiflet systems and zero moments. IEEE Transactions on Signal Processing, 46: 761-766. doi: 10.1109/78.661342 [32] Castro L M S. 2010. Polynomial wavelets in hybrid-mixed stress finite element models. International Journal for Numerical Methods in Biomedical Engineering, 26: 1293-1312. doi: 10.1002/cnm.1215 [33] Charton P, Perrier V. 1996. A pseudo-wavelet sheme for the two-dimensional navier stokes equations. Computational and Applied Mathematics, 15: 139-160. [34] Chen M Q, Hwang C, Shih Y P. 1996. The computation of wavelet-Galerkin approximation on a bounded interval. International Journal for Numerical Methods in Engineering, 39: 2921-2944. doi: 10.1002/(SICI)1097-0207(19960915)39:17<2921::AID-NME983>3.0.CO;2-D [35] Chen X F, Yang S J, Ma J X, et al. 2004. The construction of wavelet finite element and its application. Finite Elements in Analysis and Design, 40: 541-554. doi: 10.1016/S0168-874X(03)00077-5 [36] Cheng J, Tu X, Ghosh S. 2020. Wavelet-enriched adaptive hierarchical FE model for coupled crystal plasticity-phase field modeling of crack propagation in polycrystalline microstructures. Computer Methods in Applied Mechanics and Engineering, 361: 112757. doi: 10.1016/j.cma.2019.112757 [37] Chorin A J. 1997. A numerical method for solving incompressible viscous flow problems. Journal of Computational Physics, 135: 118-125. doi: 10.1006/jcph.1997.5716 [38] Chui C K, Li C. 1996. Dyadic affine decompositions and functional wavelet transforms. SIAM journal on mathematical analysis, 27: 865-890. doi: 10.1137/0527046 [39] Chui C K, Quak E. Wavelets on a bounded interval//D. BRAESS, L. L. SCHUMAKER. Numerical Methods in Approximation Theory, Vol. 9. Basel: Birkhäuser Basel, 1992: 53-75. [40] Cockburn B, Shu C W. 1989. TVB Runge-Kutta local projection Discontinuous Galerkin finite element method for conservation laws II: general framework. Mathematics of Computation, 52: 411-435. [41] Cockburn B, Shu C W. 1991. The Runge-Kutta local projection p1-Discontinuous-Galerkin finite element method for scalar conservation laws. ESAIM: Mathematical Modelling and Numerical Analysis, 25: 337-361. doi: 10.1051/m2an/1991250303371 [42] Cohen A, Daubechies I, Feauveau J C. 1992. Biorthogonal bases of compactly supported wavelets. Communications on Pure and Applied Mathematics, 45: 485-560. doi: 10.1002/cpa.3160450502 [43] Cohen A, Daubechies I, Vial P. 1993. Wavelets on the interval and fast wavelet transforms. Applied and Computational Harmonic Analysis, 1: 54-81. doi: 10.1006/acha.1993.1005 [44] Cohen A, Kaber S, Müller S, et al. 2003. Fully adaptive multiresolution finite volume schemes for conservation laws. Mathematics of Computation, 72: 183-225. [45] Costa B, Don W S. 2007. Multi-domain hybrid spectral-WENO methods for hyperbolic conservation laws. Journal of Computational Physics, 224: 970-991. doi: 10.1016/j.jcp.2006.11.002 [46] Daftardar-Gejji V, Jafari H. 2005. Adomian decomposition: a tool for solving a system of fractional differential equations. Journal of Mathematical Analysis and Applications, 301: 508-518. doi: 10.1016/j.jmaa.2004.07.039 [47] Daubechies I. 1988. Orthonormal bases of compactly supported wavelets. Communications on Pure and Applied Mathematics, 41: 909-996. doi: 10.1002/cpa.3160410705 [48] Daubechies I. Ten lectures on wavelets. Philadelphia: society for industrial and applied mathematics, 1992. [49] Daubechies I. 1993. Orthonormal bases of compactly supported wavelets II. Variations on a theme. SIAM journal on mathematical analysis, 24: 499-519. doi: 10.1137/0524031 [50] De Stefano G, Nejadmalayeri A, Vasilyev O V. 2016. Wall-resolved wavelet-based adaptive large-eddy simulation of bluff-body flows with variable thresholding. Journal of Fluid Mechanics, 788: 303-336. doi: 10.1017/jfm.2015.708 [51] De Stefano G, Vasilyev O V. 2012. A fully adaptive wavelet-based approach to homogeneous turbulence simulation. Journal of Fluid Mechanics, 695: 149-172. doi: 10.1017/jfm.2012.6 [52] De stefano G, Vasilyev O V. 2014. Wavelet-based adaptive simulations of three-dimensional flow past a square cylinder. Journal of Fluid Mechanics, 748: 433-456. doi: 10.1017/jfm.2014.193 [53] Dehda B, Azeb Ahmed A, Yazid F, et al. 2023. Numerical solution of a class of Caputo–Fabrizio derivative problem using Haar wavelet collocation method. Journal of Applied Mathematics and Computing, 69: 2761-2774. doi: 10.1007/s12190-023-01859-7 [54] Deslauriers G, Dubuc S. 1989. Symmetric iterative interpolation processes//R. A. DEVORE, E. B. SAFF. Constructive approximation: special issue: fractal approximation. Boston, MA: Springer US, 1989: 49-68. [55] Díaz A R. 1999. A wavelet–Galerkin scheme for analysis of large-scale problems on simple domains. International Journal for Numerical Methods in Engineering, 44: 1599-1616. doi: 10.1002/(SICI)1097-0207(19990420)44:11<1599::AID-NME556>3.0.CO;2-P [56] Díaz Calle J L, Devloo P R B, Gomes S M. 2005. Wavelets and adaptive grids for the discontinuous Galerkin method. Numerical Algorithms, 39: 143-154. doi: 10.1007/s11075-004-3626-9 [57] Do S, Li H, Kang M. 2017. Wavelet-based adaptation methodology combined with finite difference WENO to solve ideal magnetohydrodynamics. Journal of Computational Physics, 339: 482-499. doi: 10.1016/j.jcp.2017.03.028 [58] Doetsch G. 2012. Introduction to the theory and application of the Laplace transformation. Springer Science & Business Media. [59] Dong W, Bovik A C. 1998. Generalized coiflets with nonzero-centered vanishing moments. IEEE Transactions on Circuits and Systems II: Analog and Digital Signal Processing, 45: 988-1001. [60] Donoho D L 1992. Interpolating wavelet transforms. Technical Report 3; Preprint; Department of Statistics, Stanford University: Stanford, CA, USA, . [61] Feng Y, Wang J, Liu X, et al. 2023. A wavelet method for large-deflection bending of irregular plates. International Journal of Mechanical Sciences, 252: 108358. doi: 10.1016/j.ijmecsci.2023.108358 [62] Fröhlich J, Schneider K. 1994. An adaptive wavelet Galerkin algorithm for one and two dimensional flame computations. European journal of mechanics. B, Fluids 13 : 439-471. [63] Fröhlich J, Schneider K. 1996. Numerical simulation of decaying turbulence in an adaptive wavelet basis. Applied and Computational Harmonic Analysis, 3: 393-397. doi: 10.1006/acha.1996.0033 [64] Fu L. 2023. Review of the high-order TENO schemes for compressible gas dynamics and turbulence. Archives of Computational Methods in Engineering, 30: 2493-2526. doi: 10.1007/s11831-022-09877-7 [65] Fu L, Hu X Y, Adams N A. 2016. A family of high-order targeted ENO schemes for compressible-fluid simulations. Journal of Computational Physics, 305: 333-359. doi: 10.1016/j.jcp.2015.10.037 [66] Gerhard N, Iacono F, May G, et al. 2015. A high-order discontinuous Galerkin discretization with multiwavelet-based grid adaptation for compressible flows. Journal of Scientific Computing, 62: 25-52. doi: 10.1007/s10915-014-9846-9 [67] Gerhard N, Müller S. 2016. Adaptive multiresolution discontinuous Galerkin schemes for conservation laws: multi-dimensional case. Computational and Applied Mathematics, 35: 321-349. doi: 10.1007/s40314-014-0134-y [68] Goswami J C, Chan A K, Chui C K. 1995. On solving first-kind integral-equations using wavelets on a bounded interval. IEEE Transactions On Antennas And Propagation, 43: 614-622. doi: 10.1109/8.387178 [69] Gottlieb D, Shu C W. 1997. On the Gibbs phenomenon and its resolution. SIAM Review, 39: 644-668. doi: 10.1137/S0036144596301390 [70] Haar A. 1910. Zur Theorie der orthogonalen Funktionensysteme. Mathematische Annalen, 69: 331-371. doi: 10.1007/BF01456326 [71] Harbrecht H, Paiva F, Pérez C, et al. 2002. Biorthogonal wavelet approximation for the coupling of FEM-BEM. Numerische Mathematik, 92: 325-356. doi: 10.1007/s002110100283 [72] Harten A. 1994. Adaptive multiresolution schemes for shock computations. Journal of Computational Physics, 115: 319-338. doi: 10.1006/jcph.1994.1199 [73] Harten A. 1995. Multiresolution algorithms for the numerical solution of hyperbolic conservation laws. Communications on Pure and Applied Mathematics, 48: 1305-1342. doi: 10.1002/cpa.3160481201 [74] Harten A, Engquist B, Osher S, et al. 1987. Uniformly high order accurate essentially non-oscillatory schemes, III. Journal of Computational Physics, 71: 231-303. doi: 10.1016/0021-9991(87)90031-3 [75] He W Y, Ren W X. 2012. Finite element analysis of beam structures based on trigonometric wavelet. Finite Elements in Analysis and Design, 51: 59-66. doi: 10.1016/j.finel.2011.11.005 [76] Holmström M. 1999. Solving hyperbolic PDEs using interpolating wavelets. SIAM Journal on Scientific Computing, 21: 405-420. doi: 10.1137/S1064827597316278 [77] Holmström M, Walden J. 1998. Adaptive wavelet methods for hyperbolic PDEs. Journal of Scientific Computing, 13: 19-49. doi: 10.1023/A:1023252610346 [78] Hosseini V R, Chen W, Avazzadeh Z. 2014. Numerical solution of fractional telegraph equation by using radial basis functions. Engineering Analysis with Boundary Elements, 38: 31-39. doi: 10.1016/j.enganabound.2013.10.009 [79] Hou Z, Weng J, Liu X, et al. 2022. A sixth-order wavelet integral collocation method for solving nonlinear boundary value problems in three dimensions. Acta Mechanica Sinica, 38: 421-453. doi: 10.1007/s10409-021-09039-x [80] Hovhannisyan N, Müller S, Schäfer R. 2014. Adaptive multiresolution discontinuous Galerkin schemes for conservation laws. Mathematics of Computation, 83: 113-151. [81] Hryniewicz Z. 2011. Dynamics of Rayleigh beam on nonlinear foundation due to moving load using Adomian decomposition and coiflet expansion. Soil Dynamics and Earthquake Engineering, 31: 1123-1131. doi: 10.1016/j.soildyn.2011.03.013 [82] Hughes T J R. 2012. The finite element method: linear static and dynamic finite element analysis. Courier Corporation. [83] Hughes T J R, Franca L P, Mallet M. 1986. A new finite element formulation for computational fluid dynamics: I. Symmetric forms of the compressible Euler and Navier-Stokes equations and the second law of thermodynamics. Computer Methods in Applied Mechanics and Engineering, 54: 223-234. doi: 10.1016/0045-7825(86)90127-1 [84] Hughes T J R, Mallet M. 1986a. A new finite element formulation for computational fluid dynamics: III. The generalized streamline operator for multidimensional advective-diffusive systems. Computer Methods in Applied Mechanics and Engineering, 58: 305-328. doi: 10.1016/0045-7825(86)90152-0 [85] Hughes T J R, Mallet M. 1986b. A new finite element formulation for computational fluid dynamics: IV. A discontinuity-capturing operator for multidimensional advective-diffusive systems. Computer Methods in Applied Mechanics and Engineering, 58: 329-336. doi: 10.1016/0045-7825(86)90153-2 [86] Hughes T J R, Mallet M, Akira M. 1986. A new finite element formulation for computational fluid dynamics: II. Beyond SUPG. Computer Methods in Applied Mechanics and Engineering, 54: 341-355. doi: 10.1016/0045-7825(86)90110-6 [87] Ii S, Xiao F. 2009. High order multi-moment constrained finite volume method. Part I: Basic formulation. Journal of Computational Physics, 228: 3669-3707. doi: 10.1016/j.jcp.2009.02.009 [88] Ingman D, Suzdalnitsky J. 2004. Control of damping oscillations by fractional differential operator with time-dependent order. Computer Methods in Applied Mechanics and Engineering, 193: 5585-5595. doi: 10.1016/j.cma.2004.06.029 [89] Jena S K, Chakraverty S, Mahesh V, et al. 2022. Application of Haar wavelet discretization and differential quadrature methods for free vibration of functionally graded micro-beam with porosity using modified couple stress theory. Engineering Analysis with Boundary Elements, 140: 167-185. doi: 10.1016/j.enganabound.2022.04.009 [90] Jia R Q, Liu S T. 2006. Wavelet bases of Hermite cubic splines on the interval. Advances in Computational Mathematics, 25: 23-39. doi: 10.1007/s10444-003-7609-5 [91] Jiang G S, Shu C W. 1996. Efficient implementation of weighted ENO schemes. Journal of Computational Physics, 126: 202-228. doi: 10.1006/jcph.1996.0130 [92] Jin J M, Xue P X, Xu Y X, et al. 2006. Compactly supported non-tensor product form two-dimension wavelet finite element. Applied Mathematics and Mechanics, 27: 1673-1686. doi: 10.1007/s10483-006-1210-z [93] Johnston H, Leake C, Mortari D. 2020. Least-squares solutions of eighth-order boundary value problems using the theory of functional connections. Mathematics, 8: 397. doi: 10.3390/math8030397 [94] Karniadakis G E, Israeli M, Orszag S A. 1991. High-order splitting methods for the incompressible Navier-Stokes equations. Journal of Computational Physics, 97: 414-443. doi: 10.1016/0021-9991(91)90007-8 [95] Kevlahan N K R, Vasilyev O V. 2005. An adaptive wavelet collocation method for fluid-structure interaction at high Reynolds numbers. SIAM Journal on Scientific Computing, 26: 1894-1915. doi: 10.1137/S1064827503428503 [96] Kim G, Han P, An K, et al. 2021a. Free vibration analysis of functionally graded double-beam system using Haar wavelet discretization method. Engineering Science and Technology, an International Journal, 24: 414-427. doi: 10.1016/j.jestch.2020.07.009 [97] Kim K, Kwak S, Choe K, et al. 2021b. Application of Haar wavelet method for free vibration of laminated composite conical–cylindrical coupled shells with elastic boundary condition. Physica Scripta, 96: 035223. doi: 10.1088/1402-4896/abd9f7 [98] Koro K, Abe K. 2001. Non-orthogonal spline wavelets for boundary element analysis. Engineering Analysis with Boundary Elements, 25: 149-164. doi: 10.1016/S0955-7997(01)00036-4 [99] Kozakevicius A d J, Zeidan D, Schmidt A A, et al. 2018. Solving a mixture model of two-phase flow with velocity non-equilibrium using WENO wavelet methods. International Journal of Numerical Methods for Heat & Fluid Flow, 28: 2052-2071. [100] Koziol P, Hryniewicz Z. 2006. Analysis of bending waves in beam on viscoelastic random foundation using wavelet technique. International Journal of Solids and Structures, 43: 6965-6977. doi: 10.1016/j.ijsolstr.2006.02.018 [101] Koziol P, Mares C. 2010. Wavelet approach for vibration analysis of fast moving load on a viscoelastic medium. Shock and Vibration, 17: 461-472. doi: 10.1155/2010/278538 [102] Krivodonova L, Xin J, Remacle J F, et al. 2004. Shock detection and limiting with discontinuous Galerkin methods for hyperbolic conservation laws. Applied Numerical Mathematics, 48: 323-338. doi: 10.1016/j.apnum.2003.11.002 [103] Kuhlman K L. 2013. Review of inverse Laplace transform algorithms for Laplace-space numerical approaches. Numerical Algorithms, 63: 339-355. doi: 10.1007/s11075-012-9625-3 [104] Lang F G, Xu X P. 2011. Quartic B-spline collocation method for fifth order boundary value problems. Computing, 92: 365-378. doi: 10.1007/s00607-011-0149-9 [105] Latto A, Tenenbaum E. 1990. Compactly supported wavelets and the numerical solution of Burgers’ equation. Comptes rendus de l'Académie des sciences. Série I, Mathématique, 311: 903-909. [106] Lee T S. 1996. Image representation using 2D Gabor wavelets. IEEE Transactions on Pattern Analysis and Machine Intelligence, 18: 959-971. doi: 10.1109/34.541406 [107] Lemarié P G. 1988. Ondelettes à localisation exponentielle. J. Math. Pures Appl., 67: 227-236. [108] LeVeque R J. 2002. Finite volume methods for hyperbolic problems. Cambridge university press. [109] Levin P L, Spasojevic M, Schneider R. 1998. Creation of sparse boundary element matrices for 2-D and axi-symmetric electrostatics problems using the bi-orthogonal Haar wavelet. IEEE Transactions on Dielectrics and Electrical Insulation, 5: 469-484. doi: 10.1109/94.708261 [110] Lewis R M. 1994. Cardinal interpolating multiresolutions. Journal of Approximation Theory, 76: 177-202. doi: 10.1006/jath.1994.1013 [111] Li B, Chen X. 2014. Wavelet-based numerical analysis: a review and classification. Finite Elements in Analysis and Design, 81: 14-31. doi: 10.1016/j.finel.2013.11.001 [112] Li B, Chen X F, Ma J X, et al. 2005. Detection of crack location and size in structures using wavelet finite element methods. Journal of Sound and Vibration, 285: 767-782. doi: 10.1016/j.jsv.2004.08.040 [113] Li C, Zeng F. 2012. Finite difference methods for fractional differential equations. International Journal of Bifurcation and Chaos, 22: 1230014. doi: 10.1142/S0218127412300145 [114] Liandrat J, Tchamitchian P H 1990. Resolution of the 1D regularized Burgers equation using a spatial wavelet approximation. NASA Contractor Rep. NASA Langley Research Center; Hampton. [115] Liu F, Zhuang P, Turner I, et al. 2014. A new fractional finite volume method for solving the fractional diffusion equation. Applied Mathematical Modelling, 38: 3871-3878. doi: 10.1016/j.apm.2013.10.007 [116] Liu G. 2009. Meshfree methods: moving beyond the finite element method. Boca Raton: CRC Press. [117] Liu G, Wu T. 2001. Application of generalized differential quadrature rule in Blasius and Onsager equations. International Journal for Numerical Methods in Engineering, 52: 1013-1027. doi: 10.1002/nme.251 [118] Liu G, Wu T. 2002. Differential quadrature solutions of eighth-order boundary-value differential equations. Journal of Computational and Applied Mathematics, 145: 223-235. doi: 10.1016/S0377-0427(01)00577-5 [119] Liu X, Liu G, Wang J, et al. 2019. A wavelet multiresolution interpolation Galerkin method for targeted local solution enrichment. Computational Mechanics, 64: 986-1096. [120] Liu X, Liu G R, Wang J, et al. 2020a. A wavelet multi-resolution enabled interpolation Galerkin method for two-dimensional solids. Engineering Analysis with Boundary Elements, 117: 251-268. doi: 10.1016/j.enganabound.2020.04.007 [121] Liu X, Liu G R, Wang J, et al. 2020b. A wavelet multiresolution interpolation Galerkin method with effective treatments for discontinuity for crack growth analyses. Engineering Fracture Mechanics, 225: 106836. doi: 10.1016/j.engfracmech.2019.106836 [122] Liu X, Wang J, Wang X, et al. 2014. Exact solutions of multi-term fractional diffusion-wave equations with Robin type boundary conditions. Applied Mathematics and Mechanics, 35: 49-62. doi: 10.1007/s10483-014-1771-6 [123] Liu X, Wang J, Zhou Y. 2013. A wavelet method for solving coupled viscous Burgers' equations. AIP Conference Proceedings, 1558: 935-937. [124] Liu X, Zhou Y, Wang J. 2016. A space-time fully decoupled wavelet Galerkin method for solving two-dimensional Burgers' equations. Computers & Mathematics with Applications, 72: 2908-2919. [125] Liu X, Zhou Y, Wang J. 2022. Highly accurate wavelet solution for bending and free vibration of circular plates over extra-wide ranges of deflections. Journal of Applied Mechanics, 90: 031009. [126] Liu X, Zhou Y, Wang X, et al. 2013. A wavelet method for solving a class of nonlinear boundary value problems. Communications in Nonlinear Science and Numerical Simulation, 18: 1939-1948. doi: 10.1016/j.cnsns.2012.12.010 [127] Liu X, Zhou Y, Zhang L, et al. 2014. Wavelet solutions of Burgers’ equation with high Reynolds numbers. Science China Technological Sciences, 57: 1285-1292. doi: 10.1007/s11431-014-5588-z [128] Liu X D, Osher S, Chan T. 1994. Weighted essentially non-oscillatory schemes. Journal of Computational Physics, 115: 200-212. doi: 10.1006/jcph.1994.1187 [129] Liu Y, Liu Y, Cen Z. 2008. Daubechies wavelet meshless method for 2-D elastic problems. Tsinghua Science and Technology, 13: 605-608. doi: 10.1016/S1007-0214(08)70099-3 [130] Liu Y, Qin F, Liu Y, et al. 2010. A Daubechies wavelet-based method for elastic problems. Engineering Analysis with Boundary Elements, 34: 114-121. doi: 10.1016/j.enganabound.2009.08.004 [131] Lu D, Ohyoshi T, Miura K. 1997. Treatment of boundary conditions in one-dimensional wavelet-Galerkin method. JSME International Journal Series A Solid Mechanics and Material Engineering, 40: 382-388. doi: 10.1299/jsmea.40.382 [132] Ma J X, Xue J J, Yang S J, et al. 2003. A study of the construction and application of a Daubechies wavelet-based beam element. Finite Elements in Analysis and Design, 39: 965-975. doi: 10.1016/S0168-874X(02)00141-5 [133] Malan A G, Lewis R W, Nithiarasu P. 2002. An improved unsteady, unstructured, artificial compressibility, finite volume scheme for viscous incompressible flows: Part I. Theory and implementation. International Journal for Numerical Methods in Engineering, 54: 695-714. doi: 10.1002/nme.447 [134] Mallat S, Hwang W L. 1992. Singularity detection and processing with wavelets. Ieee Transactions on Information Theory, 38: 617-643. doi: 10.1109/18.119727 [135] Mallat S, Peyré G. 2007. A review of bandlet methods for geometrical image representation. Numerical Algorithms, 44: 205-234. doi: 10.1007/s11075-007-9092-4 [136] Mallat S G. 1989. A theory for multiresolution signal decomposition: the wavelet representation. IEEE Transactions on Pattern Analysis and Machine Intelligence, 11: 674-693. doi: 10.1109/34.192463 [137] Mallat S G. 2010. A Wavelet tour of signal processing. Burlington: Elsevier. [138] Meyer Y. 1986. Principe d’incertitude, bases hilbertiennes et algebres d’operateurs. Astérisque, 1985/86: 209-223. [139] Meyer Y. 1992. Wavelets and operators. Cambridge: Cambridge university press. [140] Minbashian H, Adibi H, Dehghan M. 2017. An adaptive wavelet space-time SUPG method for hyperbolic conservation laws. Numerical Methods for Partial Differential Equations, 33: 2062-2089. doi: 10.1002/num.22180 [141] Monzón L, Beylkin G, Hereman W. 1999. Compactly supported wavelets based on almost interpolating and nearly linear phase filters (Coiflets). Applied and Computational Harmonic Analysis, 7: 184-210. doi: 10.1006/acha.1999.0266 [142] Nakagoshi S, Noguchi H. 2001. A modified wavelet Galerkin method for analysis of Mindlin plates. JSME International Journal Series A Solid Mechanics and Material Engineering, 44: 610-615. doi: 10.1299/jsmea.44.610 [143] Nastos C V, Theodosiou T C, Rekatsinas C S, et al. 2018. A 2D Daubechies finite wavelet domain method for transient wave response analysis in shear deformable laminated composite plates. Computational Mechanics, 62: 1187-1198. doi: 10.1007/s00466-018-1558-9 [144] Nejadmalayeri A, Vezolainen A, Brown-Dymkoski E, et al. 2015. Parallel adaptive wavelet collocation method for PDEs. Journal of Computational Physics, 298: 237-253. doi: 10.1016/j.jcp.2015.05.028 [145] Nejadmalayeri A, Vezolainen A, De stefano G, et al. 2014. Fully adaptive turbulence simulations based on Lagrangian spatio-temporally varying wavelet thresholding. Journal of Fluid Mechanics, 749: 794-817. doi: 10.1017/jfm.2014.241 [146] Noor M A, Mohyud-Din S T. 2008. Homotopy perturbation method for solving sixth-order boundary value problems. Computers & Mathematics with Applications, 55: 2953-2972. [147] Oertel H. 2004. Prandtl’s essentials of fluid mechanics. New York: Springer. [148] Patankar S. 1980. Numerical heat transfer and fluid flow. Taylor & Francis. [149] Pereira R M, Nguyen Van Yen N, Schneider K, et al. 2022. Adaptive solution of initial value problems by a dynamical Galerkin scheme. Multiscale Modeling & Simulation, 20: 1147-1166. [150] Pereira R M, Nguyen Van Yen N, Schneider K, et al. 2023. Are adaptive Galerkin schemes dissipative. SIAM Review, 65: 1109-1134. doi: 10.1137/23M1588627 [151] Pereira R M, Nguyen Van Yen R, Farge M, et al. 2013. Wavelet methods to eliminate resonances in the Galerkin-truncated Burgers and Euler equations. Physical Review E, 87: 033017. doi: 10.1103/PhysRevE.87.033017 [152] Pindza E, Owolabi K M. 2016. Fourier spectral method for higher order space fractional reaction–diffusion equations. Communications in Nonlinear Science and Numerical Simulation, 40: 112-128. doi: 10.1016/j.cnsns.2016.04.020 [153] Pirozzoli S. 2011. Numerical methods for high-speed flows. Annual Review of Fluid Mechanics, 43: 163-194. doi: 10.1146/annurev-fluid-122109-160718 [154] Portnoff M. 1980. Time-frequency representation of digital signals and systems based on short-time Fourier analysis. IEEE Transactions on Acoustics, Speech, and Signal Processing, 28: 55-69. doi: 10.1109/TASSP.1980.1163359 [155] Quak E, Weyrich N. 1994. Decomposition and reconstruction algorithms for spline wavelets on a bounded interval. Applied and Computational Harmonic Analysis, 1: 217-231. doi: 10.1006/acha.1994.1009 [156] Ray S S, Frisch U, Nazarenko S, et al. 2011. Resonance phenomenon for the Galerkin-Truncated Burgers and Euler equations. Physical Review E, 84: 016301. [157] Regele J D, Vasilyev O V. 2009. An adaptive wavelet-collocation method for shock computations. International Journal of Computational Fluid Dynamics, 23: 503-518. doi: 10.1080/10618560903117105 [158] Restrepo J M, Leaf G K. 1995. Wavelet-Galerkin discretization of hyperbolic equations. Journal of Computational Physics, 122: 118-128. doi: 10.1006/jcph.1995.1201 [159] Roe P L. 1986. Characteristic-based schemes for the Euler equations. Annual Review of Fluid Mechanics, 18: 337-365. doi: 10.1146/annurev.fl.18.010186.002005 [160] Ruch D K, Van Fleet P J. 2009. Wavelet theory: an elementary approach with applications. John Wiley & Sons. [161] Saito N, Beylkin G. 1993. Multiresolution representations using the autocorrelation functions of compactly supported wavelets. IEEE Transactions on Signal Processing, 41: 3584-3590. doi: 10.1109/78.258102 [162] Schmidt A A, Kozakevicius A J, Zeidan D, et al. 2020. Two-dimensional two-phase flow Riemann problem simulations using WENO wavelet methods. AIP Conference Proceedings, 2293: 030029. [163] Schneider K, Farge M 2002. Adaptive wavelet simulation of a flow around an impulsively started cylinder using penalisation. Applied and Computational Harmonic Analysis, 12 : 374-380. [164] Schneider K, Vasilyev O V. 2010. Wavelet methods in computational fluid dynamics. Annual Review of Fluid Mechanics, 42: 473-503. doi: 10.1146/annurev-fluid-121108-145637 [165] Shah F A, Abass R, Debnath L. 2017. Numerical solution of fractional differential equations using Haar wavelet operational matrix method. International Journal of Applied and Computational Mathematics, 3: 2423-2445. [166] Shen J, Tang T, Wang L L. 2011. Spectral methods: algorithms, analysis and applications. Springer Science & Business Media. [167] Shu C W, Osher S. 1988. Efficient implementation of essentially non-oscillatory shock-capturing schemes. Journal of Computational Physics, 77: 439-471. doi: 10.1016/0021-9991(88)90177-5 [168] Sod G A. 1978. A survey of several finite difference methods for systems of nonlinear hyperbolic conservation laws. Journal of Computational Physics, 27: 1-31. doi: 10.1016/0021-9991(78)90023-2 [169] Shu C W. 2009. High order weighted essentially nonoscillatory schemes for convection dominated problems. SIAM Review, 51: 82-126. [170] Song L, Zhang H. 2007. Application of homotopy analysis method to fractional KdV–Burgers–Kuramoto equation. Physics Letters A, 367: 88-94. doi: 10.1016/j.physleta.2007.02.083 [171] Spasojevic M, Schneider R, Levin P L. 1997. On the creation of sparse boundary element matrices for two dimensional electrostatics problems using the orthogonal Haar wavelet. IEEE Transactions on Dielectrics and Electrical Insulation, 4: 249-258. doi: 10.1109/94.598281 [172] Sun Z X, Jin G Y, Ye T G, et al. 2022. A three-dimensional B-spline wavelet finite element method for structural vibration analysis. Journal of Vibration and Control, 29: 5683-5697. [173] Sweldens W. 1996. The lifting scheme: a custom-design construction of biorthogonal wavelets. Applied and Computational Harmonic Analysis, 3: 186-200. doi: 10.1006/acha.1996.0015 [174] Sweldens W. 1998. The lifting scheme: a construction of second generation wavelets. SIAM journal on mathematical analysis, 29: 511-546. doi: 10.1137/S0036141095289051 [175] Sweldens W, Schroder P. 2005. Building your own wavelets at home//Wavelets in the Geosciences. Berlin Heidelberg: Springer. [176] Tanaka S, Okada H, Okazawa S, et al. 2013. Fracture mechanics analysis using the wavelet Galerkin method and extended finite element method. International Journal for Numerical Methods in Engineering, 93: 1082-1108. doi: 10.1002/nme.4433 [177] Tanaka S, Suzuki H, Ueda S, et al. 2015. An extended wavelet Galerkin method with a high-order B-spline for 2D crack problems. Acta Mechanica, 226: 2159-2175. doi: 10.1007/s00707-015-1306-6 [178] Tang J, Chen C, Shen X, et al. 2022. A three-dimensional positivity-preserving and conservative multimoment finite-volume transport model on a cubed-sphere grid. Quarterly Journal of the Royal Meteorological Society, 148: 3622-3638. doi: 10.1002/qj.4377 [179] Tezduyar T E, Liou J, Ganjoo D K, et al. 1990. Solution techniques for the vorticity–streamfunction formulation of two-dimensional unsteady incompressible flows. International Journal for Numerical Methods in Fluids, 11: 515-539. doi: 10.1002/fld.1650110505 [180] Timoshenko S, Woinowsky-Krieger S. 1959. Theory of plates and shells. New York: McGraw-hill. [181] Uchaikin V V. 2013. Fractional derivatives for physicists and engineers. Springer. [182] Vasilyev O V, Bowman C. 2000. Second-generation wavelet collocation method for the solution of partial differential equations. Journal of Computational Physics, 165: 660-693. doi: 10.1006/jcph.2000.6638 [183] Vasilyev O V, Kevlahan N K R. 2002. Hybrid wavelet collocation–brinkman penalization method for complex geometry flows. International Journal for Numerical Methods in Fluids, 40: 531-538. doi: 10.1002/fld.307 [184] Vasilyev O V, Paolucci S. 1996. A dynamically adaptive multilevel wavelet collocation method for solving partial differential equations in a finite domain. Journal of Computational Physics, 125: 498-512. doi: 10.1006/jcph.1996.0111 [185] Wang J, Feng Y, Xu C, et al. 2023. Multiresolution method for bending of plates with complex shapes. Applied Mathematics and Mechanics, 44: 561-582. doi: 10.1007/s10483-023-2972-8 [186] Wang J, Gao H. 2005. A simplified formula of Laplace inversion based on wavelet theory. Communications in Numerical Methods in Engineering, 21: 527-530. doi: 10.1002/cnm.765 [187] Wang J, Liu X, Zhou Y. 2024. Application of wavelet methods in computational physics. Annalen der Physik. [188] Wang J, Zhang L, Zhou Y. 2018. A simultaneous space-time wavelet method for nonlinear initial boundary value problems. Applied Mathematics and Mechanics, 39: 1547-1566. doi: 10.1007/s10483-018-2384-6 [189] Wang J, Zhou Y, Gao H. 2003. Computation of the Laplace inverse transform by application of the wavelet theory. International Journal for Numerical Methods in Biomedical Engineering, 19: 959-975. [190] Wang X, Liu X, Wang J, et al. 2015. A wavelet method for bending of circular plate with large deflection. Acta Mechanica Solida Sinica, 28: 83-90. doi: 10.1016/S0894-9166(15)60018-0 [191] Wang Y M, Chen X F, He Z J. 2012. A second-generation wavelet-based finite element method for the solution of partial differential equations. Applied Mathematics Letters, 25: 1608-1613. doi: 10.1016/j.aml.2012.01.021 [192] Wang Z J. 2002. Spectral (finite) volume method for conservation laws on unstructured grids. Basic formulation: basic formulation. Journal of Computational Physics, 178: 210-251. doi: 10.1006/jcph.2002.7041 [193] Wang Z J, Liu Y. 2002. Spectral (finite) volume method for conservation laws on unstructured grids: II. Extension to two-dimensional scalar equation. Journal of Computational Physics, 179: 665-697. doi: 10.1006/jcph.2002.7082 [194] Wei D. Coiflet-type wavelets: theory, design, and applications. Austin: The University of Texas, 1998. [195] Weng J, Liu X, Zhou Y, et al. 2021. A space-time fully decoupled wavelet integral collocation method with high-order accuracy for a class of nonlinear wave equations. Mathematics, 9: 2957. doi: 10.3390/math9222957 [196] Weng J, Liu X, Zhou Y, et al. 2022. An explicit wavelet method for solution of nonlinear fractional wave equations. Mathematics, 10: 4011. doi: 10.3390/math10214011 [197] Xiang J W, Liang M, Zhong Y T. 2016. Computation of stress intensity factors using wavelet-based element. Journal of Mechanics, 32: N1-N6. doi: 10.1017/jmech.2016.2 [198] Xiao J Y, Wen L H, Zhang D. 2007. A wavelet‐integration‐free periodic wavelet Galerkin BEM for 2D potential problems. Engineering Computations, 24: 306-318. doi: 10.1108/02644400710748661 [199] Xie X, Jin G, Ye T, et al. 2014. Free vibration analysis of functionally graded conical shells and annular plates using the Haar wavelet method. Applied Acoustics, 85: 130-142. doi: 10.1016/j.apacoust.2014.04.006 [200] Xu C, Wang J, Liu X, et al. 2017. Coiflet solution of strongly nonlinear p-Laplacian equations. Applied Mathematics and Mechanics, 38: 1031-1042. doi: 10.1007/s10483-017-2212-6 [201] Xu J C, Shann W C. 1992. Galerkin-wavelet methods for two-point boundary value problems. Numerische Mathematik, 63: 123-144. doi: 10.1007/BF01385851 [202] Yaghmaie R, Guo S, Ghosh S. 2016. Wavelet transformation induced multi-time scaling (WATMUS) model for coupled transient electro-magnetic and structural dynamics finite element analysis. Computer Methods in Applied Mechanics and Engineering, 303: 341-373. doi: 10.1016/j.cma.2016.01.016 [203] Yang B, Wang J, Liu X, et al. 2023. Stability and resolution analysis of the wavelet collocation upwind schemes for hyperbolic conservation laws. Fluids, 8 . [204] Yang B, Wang J, Liu X, et al. 2024. High-order adaptive multiresolution wavelet upwind schemes for hyperbolic conservation laws. Computers & Fluids, 269: 106111. [205] Yang Z, Liao S. 2017a. A HAM-based wavelet approach for nonlinear ordinary differential equations. Communications in Nonlinear Science and Numerical Simulation, 48: 439-453. doi: 10.1016/j.cnsns.2017.01.005 [206] Yang Z, Liao S. 2017b. A HAM-based wavelet approach for nonlinear partial differential equations: two dimensional Bratu problem as an application. Communications in Nonlinear Science and Numerical Simulation, 53: 249-262. doi: 10.1016/j.cnsns.2017.05.005 [207] Yang Z B, Chen X F, Li B, et al. 2012. Vibration analysis of curved shell using b-spline wavelet on the interval (BSWI) finite elements method and general shell theory. Cmes-Computer Modeling in Engineering & Sciences, 85: 129-155. [208] Yang Z B, Chen X F, Xie Y, et al. 2016. Wave motion analysis and modeling of membrane structures using the wavelet finite element method. Applied Mathematical Modelling, 40: 2407-2420. doi: 10.1016/j.apm.2015.09.071 [209] Yu Q. 2021. A hierarchical wavelet method for nonlinear bending of materially and geometrically anisotropic thin plate. Communications in Nonlinear Science and Numerical Simulation, 92: 105498. doi: 10.1016/j.cnsns.2020.105498 [210] Yu Q, Xu H, Liao S. 2018. Coiflets solutions for Föppl-von Kármán equations governing large deflection of a thin flat plate by a novel wavelet-homotopy approach. Numerical Algorithms, 79: 993-1020. doi: 10.1007/s11075-018-0470-x [211] Yu Q, Xu H, Liao S, et al. 2019. A novel homotopy-wavelet approach for solving stream function-vorticity formulation of Navier–Stokes equations. Communications in Nonlinear Science and Numerical Simulation, 67: 124-151. doi: 10.1016/j.cnsns.2018.07.001 [212] Zeid S S. 2019. Approximation methods for solving fractional equations. Chaos, Solitons & Fractals, 125 : 171-193. [213] Zeidan D, Kozakevicius A J, Schmidt A A, et al. 2016. WENO wavelet method for a hyperbolic model of two-phase flow in conservative form. AIP Conference Proceedings, 1738: 030022. [214] Zeidan D, Romenski E, Slaouti A, et al. 2007. Numerical study of wave propagation in compressible two-phase flow. International Journal for Numerical Methods in Fluids, 54: 393-417. doi: 10.1002/fld.1404 [215] Zeidan D, Schmidt A A, Kozakevicius A J, et al. 2022. Towards parallel WENO wavelet methods for the simulation of compressible two-fluid models. AIP Conference Proceedings, 2425: 020017. [216] Zhang L, Wang J, Liu X, et al. 2017. A wavelet integral collocation method for nonlinear boundary value problems in physics. Computer Physics Communications, 215: 91-102. doi: 10.1016/j.cpc.2017.02.017 [217] Zhang L, Wang J, Zhou Y H. 2015. Wavelet solution for large deflection bending problems of thin rectangular plates. Archive of Applied Mechanics, 85: 355-365. doi: 10.1007/s00419-014-0960-9 [218] Zhang L, Wang J, Zhou Y H. 2016. Large deflection and post-buckling analysis of non-linearly elastic rods by wavelet method. International Journal of Non-Linear Mechanics, 78: 45-52. doi: 10.1016/j.ijnonlinmec.2015.10.002 [219] Zhang T, Tian Y C, Tadé M O, et al. 2007. Comments on ‘The computation of wavelet‐Galerkin approximation on a bounded interval’. International Journal for Numerical Methods in Engineering, 72: 244-251. doi: 10.1002/nme.2022 [220] Zhang X, Chen X, He Z, et al. 2011. The analysis of shallow shells based on multivariable wavelet finite element method. Acta Mechanica Solida Sinica, 24: 450-460. doi: 10.1016/S0894-9166(11)60044-X [221] Zhang X W, Chen X F, Yang Z B, et al. 2014. A stochastic wavelet finite element method for 1D and 2D structures analysis. Shock and Vibration, 2014 . [222] Zhao B, Song H Y. 2021. Fuzzy Shannon wavelet finite element methodology of coupled heat transfer analysis for clearance leakage flow of single screw compressor. Engineering with Computers, 37: 2493-2503. doi: 10.1007/s00366-020-01259-6 [223] Zhou X, He Y. 2005. Using divergence free wavelets for the numerical solution of the 2-D stationary Navier–Stokes equations. Applied Mathematics and Computation, 163: 593-607. doi: 10.1016/j.amc.2004.04.001 [224] Zhou Y H, Wang J. 2004. Vibration control of piezoelectric beam-type plates with geometrically nonlinear deformation. International Journal of Non-Linear Mechanics, 39: 909-920. doi: 10.1016/S0020-7462(03)00074-X [225] Zhou Y H, Zhou J. 2008. A modified wavelet approximation for multi-resolution AWCM in simulating nonlinear vibration of MDOF systems. Computer Methods in Applied Mechanics and Engineering, 197: 1466-1478. doi: 10.1016/j.cma.2007.11.017 [226] Zhou Y. 2021. Wavelet numerical method and its applications in nonlinear problems. Singapore: Springer Singapore. [227] Zhou Y, Wang X, Wang J, et al. 2011. A wavelet numerical method for solving nonlinear fractional vibration, diffusion and wave equations. Cmes Computer Modeling in Engineering & Sciences, 77: 137-160. [228] Zhou Y H, Wang J, Zheng X J, et al. 2000. Vibration control of variable thickness plates with piezoelectric sensors and actuators based on wavelet theory. Journal of Sound and Vibration, 237: 395-410. doi: 10.1006/jsvi.2000.3031 [229] Zhu L, Fan Q. 2012. Solving fractional nonlinear Fredholm integro-differential equations by the. Communications in Nonlinear Science and Numerical Simulation, 17: 2333-2341. doi: 10.1016/j.cnsns.2011.10.014 [230] Zuo H, Chen Y, Jia F, et al. 2021. Unified wavelet finite element formulation for static and vibration analysis of laminated composite shells. Composite structures, 272: 114207. doi: 10.1016/j.compstruct.2021.114207 [231] Zuo H, Yang Z B, Chen X F, et al. 2015. Analysis of laminated composite plates using wavelet finite element method and higher-order plate theory. Composite structures, 131: 248-258. doi: 10.1016/j.compstruct.2015.04.064 -
下载:
图(5)
计量
- 文章访问数: 755
- HTML全文浏览量: 160
- PDF下载量: 297
- 被引次数: 0