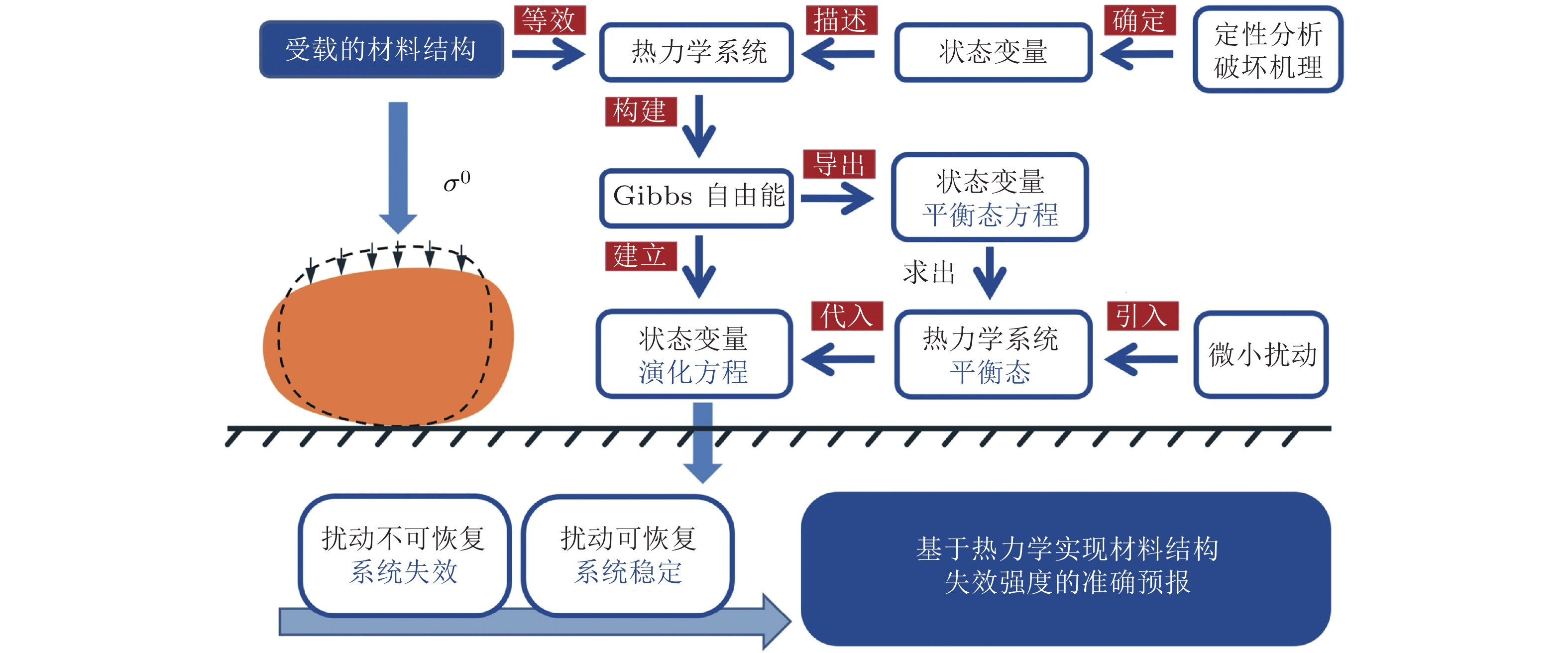
-
摘要: 材料结构强度的准确预报是工程结构设计与优化的关键, 也是固体力学的核心问题之一. 传统强度理论主要依赖于经验公式, 虽种类繁多, 但适用的材料和工况有较大的局限性. 为确保安全, 工程结构设计往往采用较大的安全系数, 造成了极大的材料浪费, 且依然无法杜绝恶性事故的发生. 如何从普适的原理出发, 突破传统强度理论的经验桎梏, 发展新的材料结构强度评估理论, 是一个亟待解决的科学与工程难题. 本文简要总结了传统强度理论所存在的问题, 概述了一些基于能量思想预报材料结构失效行为的方法, 并重点介绍了作者提出的热力学强度理论体系. 该理论体系将材料结构视为一个热力学系统, 把材料结构失效强度的预报纳入被广泛认可的热力学框架. 原则上, 该理论对材料结构的失效模式没有限制, 适用于多种失效模式的强度预报. 以几个代表性实例来说明理论的正确性和广泛适用性, 体现了极好的工程应用前景.Abstract: The accurate prediction of structural strength of materials is the key issue to the design and optimization of engineering structures and is one of the core problems in solid mechanics. Traditional strength theories mainly rely on empirical formulas, which are largely limited by the applicable materials and working conditions. To ensure safety, engineering structural design often adopts large safety factors, resulting in a significant waste of materials and still can not eliminate the occurrence of catastrophic accidents. How to break through the empirical shackles of traditional strength theories and develop a new theory of structural strength assessment of materials from universal principles is an urgent scientific and engineering problem to be solved. This article briefly summarizes the problems with traditional strength theories, outlines some methods for predicting the failure behavior of the material structures based on the energy theory, and highlights the thermodynamic strength theory proposed by the author. In this theory we treat the material structure as a thermodynamic systems and establish the relationship between the prediction of the failure strength of a material structure with the thermodynamic stability analysis. In principle, this theory has no restrictions on the failure modes of material structures and is applicable to strength prediction for a wide range of failure modes. Several representative examples are used to demonstrate the correctness and wide applicability of the theory, which reflects excellent prospects for engineering applications.
-
Key words:
- Strength theory /
- Thermodynamics /
- Failure criterion /
- Stability analysis
-
图 2 外载作用下初始裂纹为a0的薄板
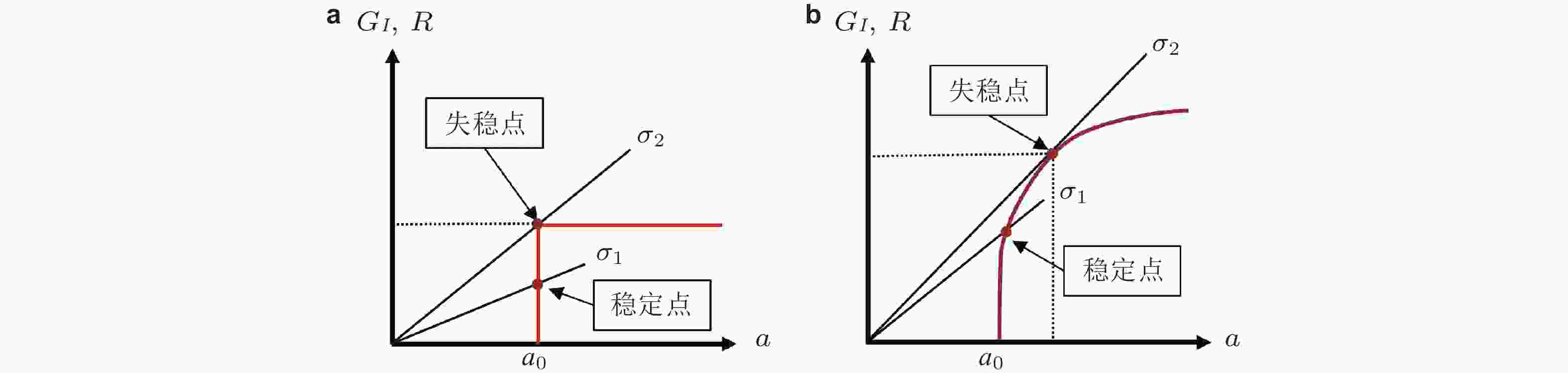
$ {G_I} = {{2\pi \sigma _{}^2ah} \mathord{\left/ {\vphantom {{2\pi \sigma _{}^2ah} {{E_{\text{e}}}}}} \right. } {{E_{\text{e}}}}} $ ,$ R = 4h\gamma + 4ah{{\partial \gamma } \mathord{\left/ {\vphantom {{\partial \gamma } {\partial a}}} \right. } {\partial a}} $ 与裂纹长度的关系图. (a)$ \gamma $ 与裂纹长度无关, R阻力是定值. 当荷载为$ \sigma _1^{} $ 时, 裂纹不扩展; 当荷载增加至$ \sigma _2^{} $ 时, 裂纹失稳扩展. (b) R阻力曲线单调递增, 当荷载为$ \sigma _1^{} $ 时, 裂纹稳定扩展; 当荷载增加至$ \sigma _2^{} $ 时, 裂纹失稳扩展图 3 (a)单轴荷载作用下无限板上的圆孔示及虚拟裂纹意图, (b) 岩石的轴向临界载荷与孔洞半径的关系, 实验数据 (黑点) 取自文献(Carter 1992)
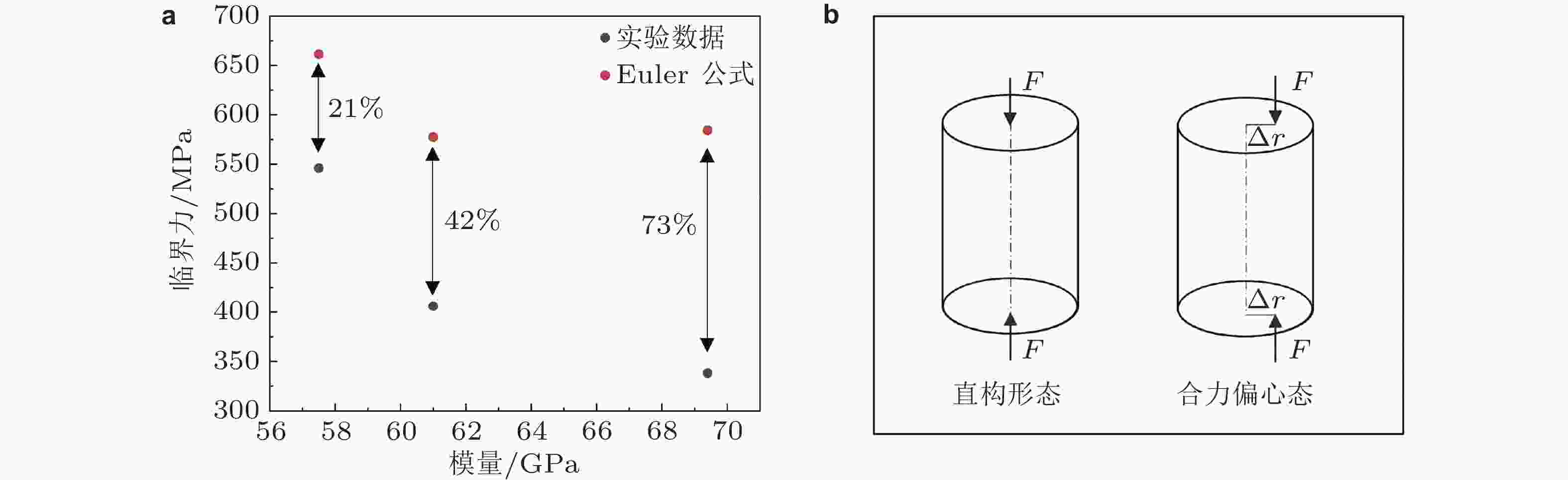
图 4 (a) 不同模量玻璃纤维增强聚合物压缩实验所得的临界荷载与Euler公式结果的对比(AlAjarmeh et al. 2019), (b) 压杆直构型态和合力偏心态示意图
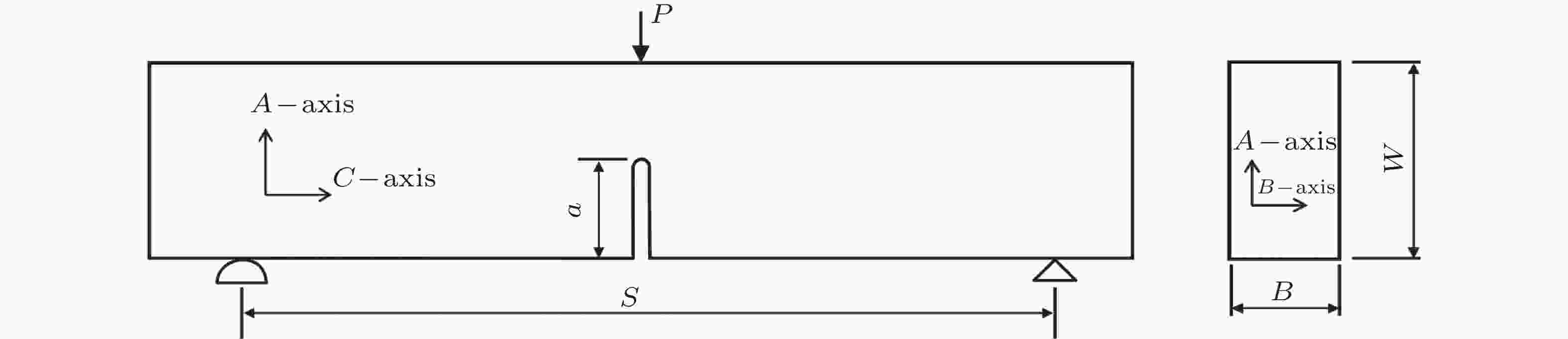
图 7 最大应力准则和全局能量准则的预报结果与铌酸锂单晶实验数据的比较(Wang 2020)
表 1 LiNbO3单晶样品结构信息和实验结果
样品编号 S/mm W/mm B/mm a/mm Pmax/N a/W 1-1 16 3.90 1.76 0.8 66.52 0.21 1-2 16 4.00 1.71 1.0 60.38 0.25 1-3 16 4.03 1.73 1.3 50.83 0.32 1-4 16 4.20 1.76 1.6 47.20 0.38 1-5 16 4.13 1.70 2.0 32.85 0.48 1-6 16 4.20 1.73 0.8 77.52 0.19 1-7 16 4.10 1.70 1.5 46.49 0.37 1-8 16 4.30 1.73 1.7 47.11 0.40 -
[1] AlAjarmeh O, Manalo A, Benmokrane B, et al. 2019. Novel testing and characterization of GFRP bars in compression. Construction and Building Materials, 225: 1112-1126. doi: 10.1016/j.conbuildmat.2019.07.280 [2] Anderson T L. Fracture mechanics: Fundamentals and applications. CRC Press, 2007. [3] Audoly B, Hutchinson J W. 2016. Analysis of necking based on a one-dimensional model. Journal of the Mechanics and Physics of Solids, 97: 68-91. doi: 10.1016/j.jmps.2015.12.018 [4] Born M. 1939. Thermodynamics of crystals and melting. The Journal of Chemical Physics, 7: 591-603. doi: 10.1063/1.1750497 [5] Born M. On the stability of crystal lattices: I. Cambridge University Press, 1940. [6] Born M, Fürth R. The stability of crystal lattices. III: An attempt to calculate the tensile strength of a cubic lattice by purely static considerations. Cambridge University Press, 1940. [7] Carter B. 1992. Size and stress gradient effects on fracture around cavities. Rock Mechanics and Rock Engineering, 25: 167-186. doi: 10.1007/BF01019710 [8] Chaboche J L. 1981. Continuous damage mechanics: a tool to describe phenomena before crack initiation. Nuclear Engineering and Design, 64: 233-247. doi: 10.1016/0029-5493(81)90007-8 [9] Chaboche J L. 1988. Continuum damage mechanics: Part I:General concepts. Journal of Applied Mechanics, 55: 59-64. doi: 10.1115/1.3173661 [10] Chen B, Chen J, Li R, Wang B. 2023. Experimental validation of the thermodynamic theory for predicting the strength of 6061 Al alloy with complex loadings. Engineering Fracture Mechanics, 277: 109006. doi: 10.1016/j.engfracmech.2022.109006 [11] Chen J, Liu W, Wang B. 2021. Prediction of theoretical strength of diamond under complex loadings. Extreme Mechanics Letters, 44: 101233. doi: 10.1016/j.eml.2021.101233 [12] Griffith A A. 1921. The phenomena of rupture and flow in solids. Philosophical Transactions of the Royal Society of London. Series A, Containing Papers of a Mathematical or Physical Character, 221: 163-198. doi: 10.1098/rsta.1921.0006 [13] Gurson A L. 1977. Continuum theory of ductile rupture by void nucleation and growth: Part I: Yield criteria and flow rules for porous ductile media. Journal of Engineering Materials and Technology, 99: 297-300. [14] Hill R. 1975. On the elasticity and stability of perfect crystals at finite strain. Mathematical Proceedings of the Cambridge Philosophical Society, 77: 225-240. doi: 10.1017/S0305004100049549 [15] Hu Y, Chen J, Wang B. 2022. Global nonequilibrium energy criterion for predicting strength of 316L stainless steel under complex loadings: Theoretical modeling and experimental validation. Science China Physics, Mechanics & Astronomy, 65: 244611. [16] Hutchinson J. 1968. Singular behaviour at the end of a tensile crack in a hardening material. Journal of the Mechanics and Physics of Solids, 16: 13-31. doi: 10.1016/0022-5096(68)90014-8 [17] Irwin G R. Analysis of stresses and strains near the end of a crack traversing a plate. Journal of Applied Mechanics, 1957: 24. [18] Kachanov L. 1958. Rupture time under creep conditions. Izv. Akad. Nauk SSSR, 8, 26-31. [19] Kaddour A S, Hinton M J. 2013. Maturity of 3D failure criteria for fibre-reinforced composites: Comparison between theories and experiments: Part B of WWFE-II. Journal of Composite Materials, 47: 925-966. doi: 10.1177/0021998313478710 [20] Kaddour A, Hinton M. 2012. Benchmarking of triaxial failure criteria for composite laminates: Comparison between models of ‘Part (A)’of ‘WWFE-II’. Journal of Composite Materials, 46: 2595-2634. doi: 10.1177/0021998312449887 [21] Karki B, Ackland G, Crain J. 1997. Elastic instabilities in crystals from ab initio stress-strain relations. Journal of Physics: Condensed Matter, 9: 8579. doi: 10.1088/0953-8984/9/41/005 [22] Kolupaev V. 2017. Generalized strength criteria as functions of the stress angle. Journal of Engineering Mechanics, 143: 04017095. doi: 10.1061/(ASCE)EM.1943-7889.0001322 [23] Kondepudi D, Prigogine I. Modern thermodynamics: from heat engines to dissipative structures. John wiley & sons, 2014. [24] Krajcinovic. 1983. Constitutive Equations for Damaging Materials. Journal of Applied Mechanics, 50: 355-360. doi: 10.1115/1.3167044 [25] Lemaitre J. Evalution of dissipation and damage in metals submitted to dynamic loading. Proceedings of International Conference of Mechanical Behavior of Materials, 1971. [26] Lemaitre J. A course on damage mechanics. Springer Science & Business Media, 2012. [27] Lestringant C, Audoly B. 2020. A one-dimensional model for elasto-capillary necking. Proceedings of the Royal Society A, 476: 20200337. doi: 10.1098/rspa.2020.0337 [28] Liu M, Gan Y, Hanaor D A, Liu B, Chen C. 2015. An improved semi-analytical solution for stress at round-tip notches. Engineering Fracture Mechanics, 149: 134-143. doi: 10.1016/j.engfracmech.2015.10.004 [29] Liu Z, Wang B. 2021. Prediction on the theoretical strength of diamond, c-BN, Cu, and CeO2. AIP Advances, 11: 095111. doi: 10.1063/5.0063928 [30] Liu Z, Wang B. 2023. Prediction of ideal strength by machine learning. Materials Chemistry and Physics, 299: 127476. doi: 10.1016/j.matchemphys.2023.127476 [31] Milstein F, Hill R. 1979. Divergences among the born and classical stability criteria for cubic crystals under hydrostatic loading. Physical Review Letters, 43: 1411. doi: 10.1103/PhysRevLett.43.1411 [32] Mizushima K, Yip S, Kaxiras E. 1994. Ideal crystal stability and pressure-induced phase transition in silicon. Physical Review B, 50: 14952. doi: 10.1103/PhysRevB.50.14952 [33] Mohr O. 1900. Welche Umstände bedingen die Elastizitätsgrenze und den Bruch eines Materials. Zeitschrift des Vereins Deutscher Ingenieure, 46: 1572-1577. [34] Murakami S. 1988. Mechanical modeling of material damage. Journal of Applied Mechanics, 55: 280-286. doi: 10.1115/1.3173673 [35] Murakami Y. 1993. Stress intensity factors handbook: vol. 3. Journal of Applied Mechanics, 60: 1063. doi: 10.1115/1.2900983 [36] Onsager L. 1931. Reciprocal relations in irreversible processes: I. Physical Review, 37: 405. doi: 10.1103/PhysRev.37.405 [37] Onsager L. 1931. Reciprocal relations in irreversible processes: II. Physical Review, 38: 2265. doi: 10.1103/PhysRev.38.2265 [38] Rabotnov Y N. 1963. Paper 68: On the equation of state of creep. Proceedings of the Institution of Mechanical Engineers, Conference Proceedings, 178: 2-117-112-122. [39] Rice J R. 1968. A path independent integral and the approximate analysis of strain concentration by notches and cracks. Journal of Applied Mechanics, 35: 379-386. doi: 10.1115/1.3601206 [40] Timoshenko S. History of strength of materials: with a brief account of the history of theory of elasticity and theory of structures. Courier Corporation, 1983. [41] Wang B. 2020. The intrinsic nature of materials failure and the global non-equilibrium energy criterion. Science China Physics, Mechanics & Astronomy, 63: 124611. [42] Wang B. 2021. A general thermodynamic theory for predicting the failure property of material structures with complex loadings. Engineering Fracture Mechanics, 254: 107936. doi: 10.1016/j.engfracmech.2021.107936 [43] Wang B. Material strength: a rational nonequilibrium energy model for complex loadings. Journal of Applied Mechanics, 2021, 88(2). [44] Wang H, Li M. 2013. Estimate of the maximum strength of metallic glasses from finite deformation theory. Physical Review Letters, 111: 065507. doi: 10.1103/PhysRevLett.111.065507 [45] Wang J, Li J, Yip S, Phillpot S, Wolf D. 1995. Mechanical instabilities of homogeneous crystals. Physical Review B, 52: 12627. doi: 10.1103/PhysRevB.52.12627 [46] Wang J, Li J, Yip S, Wolf D, Phillpot S. 1997. Unifying two criteria of Born: Elastic instability and melting of homogeneous crystals. Physica a: Statistical Mechanics and its Applications, 240: 396-403. doi: 10.1016/S0378-4371(97)00161-1 [47] Wang J, Yip S, Phillpot S, Wolf D. 1993. Crystal instabilities at finite strain. Physical Review Letters, 71: 4182. doi: 10.1103/PhysRevLett.71.4182 [48] Wang M, Fu Y. 2021. Necking of a hyperelastic solid cylinder under axial stretching: Evaluation of the infinite-length approximation. International Journal of Engineering Science, 159: 103432. doi: 10.1016/j.ijengsci.2020.103432 [49] Wells A. Application of fracture mechanics at and beyond general yielding. British Welding Journal, 1963: 10. [50] Wu Z J, Zhao E J, Xiang H P, Hao X F, Liu X J, Meng J. 2007. Crystal structures and elastic properties of superhard Ir N 2 and Ir N 3 from first principles. Physical Review B, 76: 054115. doi: 10.1103/PhysRevB.76.054115 [51] Yao Y, Hu J, Zhou A, Luo T, Wang N. 2015. Unified strength criterion for soils, gravels, rocks, and concretes. Acta Geotechnica, 10: 749-759. doi: 10.1007/s11440-015-0404-x [52] Yasnikov I S, Vinogradov A, Estrin Y. 2014. Revisiting the Considère criterion from the viewpoint of dislocation theory fundamentals. Scripta Materialia, 76: 37-40. doi: 10.1016/j.scriptamat.2013.12.009 [53] Yip S, Li J, Tang M, Wang J. 2001. Mechanistic aspects and atomic-level consequences of elastic instabilities in homogeneous crystals. Materials Science and Engineering: A, 317: 236-240. doi: 10.1016/S0921-5093(01)01162-5 [54] Yu M. 2002. Advances in strength theories for materials under complex stress state in the 20th century. Applied Mechanics Reviews, 55: 169-218. [55] Yu M. Unified strength theory and its applications. Springer-Verlag, 2004. [56] Yu X, Fu Y. 2022. An analytic derivation of the bifurcation conditions for localization in hyperelastic tubes and sheets. Zeitschrift Für Angewandte Mathematik und Physik, 73: 116. [57] Zhang C, Wang B. 2022. Influence of nonlinear spatial distribution of stress and strain on solving problems of solid mechanics. Applied Mathematics and Mechanics, 43: 1355-1366. doi: 10.1007/s10483-022-2899-7 [58] Zhang Z, Eckert J. 2005. Unified tensile fracture criterion. Physical Review Letters, 94: 094301. doi: 10.1103/PhysRevLett.94.094301 [59] Zhao Y, Deng S, Liu H, Zhang J, Guo Z, Hou H. 2018. First-principle investigation of pressure and temperature influence on structural, mechanical and thermodynamic properties of Ti3AC2 (A= Al and Si). Computational Materials Science, 154: 365-370. doi: 10.1016/j.commatsci.2018.07.007 [60] 王自强, 段祝平. 1995. 塑性细观力学. 北京: 科学出版社Wang Z Z, Duan Z P. 1995. Micromechanics of plasticity. Beijing: Science Press [61] 魏宇杰. 2020. 固体工程科学——工程材料的应用力学理论与实践. 北京: 高等教育出版社Wei Y J. 2020. Engineering Science of Solids---Applied mechanics theory and applications to engineering materials. Beijing: Reducation Press [62] 杨卫. 1992. 细观力学和细观损伤力学. 力学进展, 22: 1-9 (Yang W. 1992. Meso-Mechanics and Meso-Damage Mchanics. Advances in Mechanics, 22: 1-9).Yang W . Meso-Mechanics and Meso-Damage Mchanics[J]. Advances in mechanics, 1992, 22: 1-9 [63] 杨卫. 1995. 宏微观断裂力学. 北京: 国防工业出版社Yang W. 1995. Macro and micro fracture mechanics. Beijing: National Defence Industry Press [64] 余寿文, 冯西桥. 1997. 损伤力学. 北京: 清华大学出版社Yu S W, Feng X Q. 1997. Damage mechanics. Beijing: Tsinghua University Press -




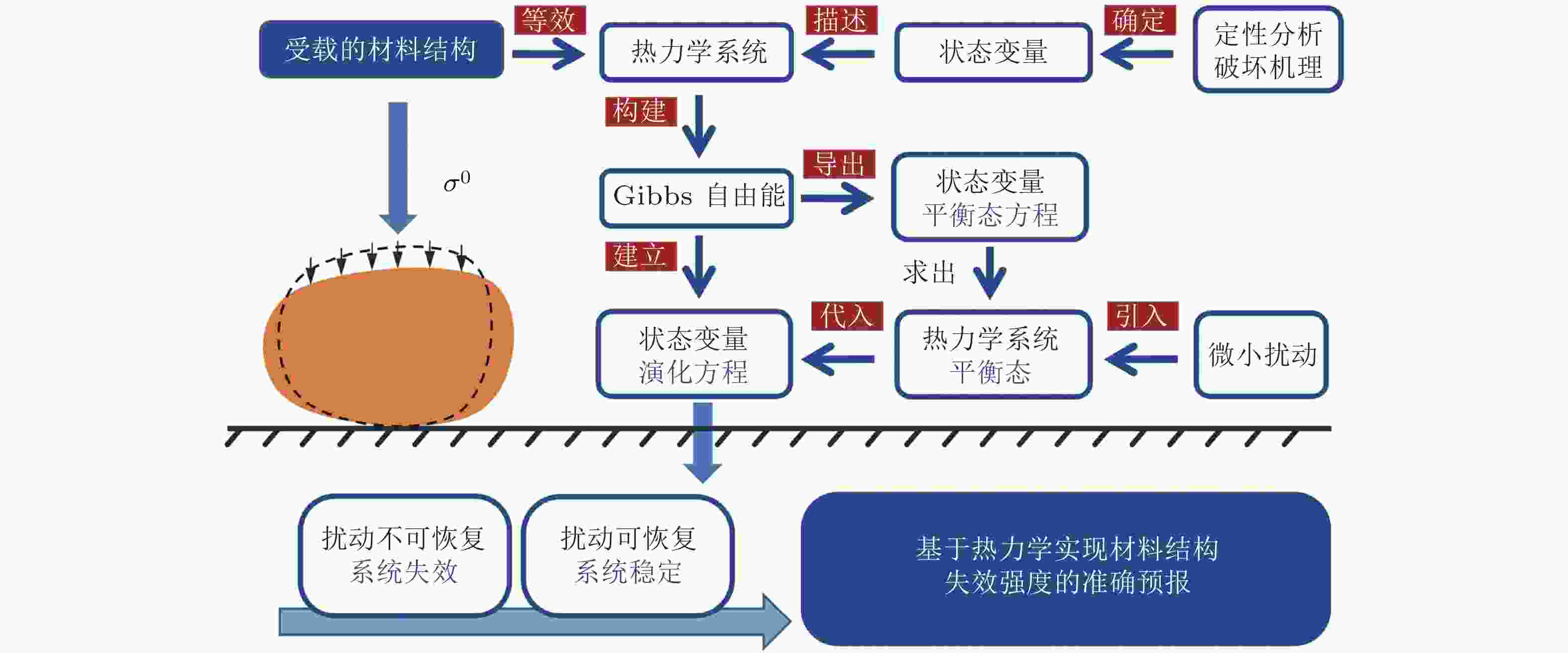
 下载:
下载: