虚单元计算方法的最新理论与应用进展

刘传奇; 许广涛; 魏宇杰

doi:10.6052/1000-0992-22-037

虚单元计算方法的最新理论与应用进展

doi: 10.6052/1000-0992-22-037

刘传奇^1,,
许广涛^{1, 2},
魏宇杰^{1, 2, 3, ,}

1.
中国科学院力学研究所非线性力学国家重点实验室, 北京 100190
2.
中国科学院大学未来技术学院, 北京 100049
3.
中国科学院大学工程科学学院, 北京 100049

基金项目: 作者们感谢国家自然科学基金委基础科学中心项目“非线性力学的多尺度问题研究”(资助号 11988102)支持和面上项目支持(资助号 12172368, 刘传奇), 刘传奇同时感谢中科院百人计划项目支持; 作者们对意大利 Milano-Bicocca 大学 L. Beirão da Veiga 教授和美国加州大学 N.Sukumar 教授关于论文算法富有成效的讨论深表谢意.

详细信息

作者简介:
刘传奇, 项目研究员, 清华大学博士, 普林斯顿大学、哥伦比亚大学博士后, 中科院BR. 2020年加入中科院力学所, 主要从事计算固体力学与颗粒材料的相关研究工作, 侧重计算方法的数学完备性以及颗粒材料宏观力学行为的物理本征. 发表专著1部、期刊论文20余篇, 主持国家自然科学基金面上项目以及若干横向项目

魏宇杰, 研究员, 1997 年从北京大学力学系获学士学位, 2000 年从中国科学院获硕士, 2006 年获麻省理工学院博士; 之后在布朗大学开展博士后研究, 2008 年加入阿拉巴马大学担任助理教授, 2010 年加入中国科学院力学研究所. 主要从事固体力学中强度与变形机理研究, 材料疲劳与断裂理论; 同时关注力学在国家重大工程中的应用. 曾获2013 年中国力学学会“青年科技奖”, 2013, 2019, 2020年中国科学院“优秀导师奖”, 获自然科学基金委杰出青年基金资助（2015—2020）

通讯作者:
yujie_wei@lnm.imech.ac.cn

中图分类号: O34
计量
- 文章访问数: 2061
- HTML全文浏览量: 1234
- PDF下载量: 853
- 被引次数: 0
出版历程
- 收稿日期: 2022-07-04
- 录用日期: 2022-08-19
- 网络出版日期: 2022-08-19
- 刊出日期: 2022-12-29

Virtual element method: Theory and applications

LIU Chuanqi^1
,,
XU Guangtao^{1, 2},
WEI Yujie^{1, 2, 3
, ,}

1.
State Key Laboratory of Nonlinear Mechanics, Institute of Mechanics, Chinese Academy of Sciences, Beijing 100190, China
2.
School of Future Technology, University of Chinese Academy of Sciences, Beijing 100049, China
3.
School of Engineering Science, University of Chinese Academy of Sciences, Beijing 100049, China

More Information

Corresponding author: yujie_wei@lnm.imech.ac.cn

摘要

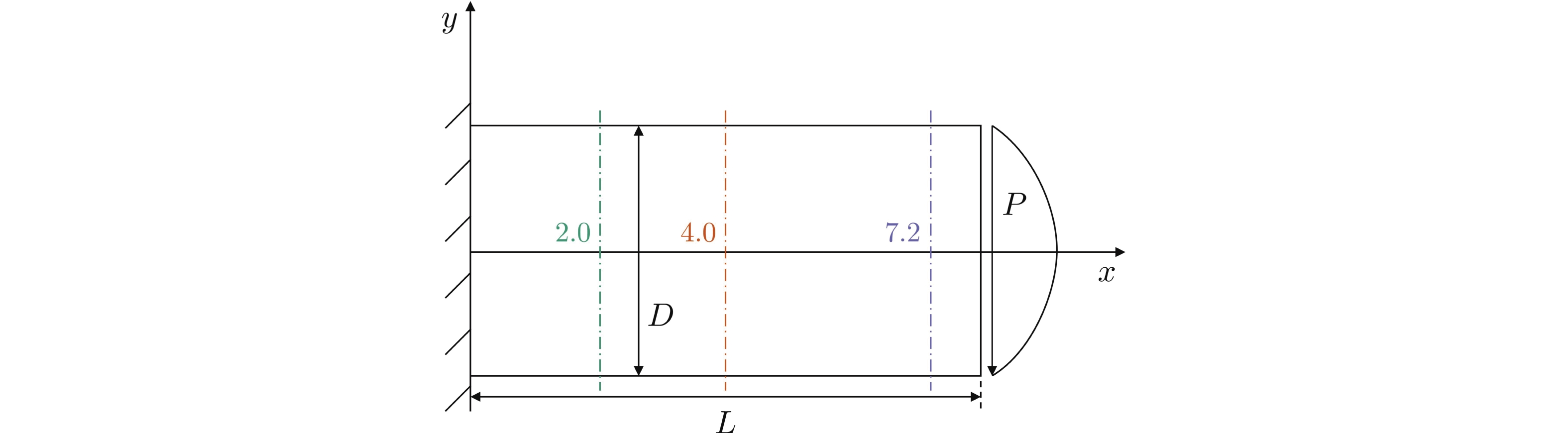
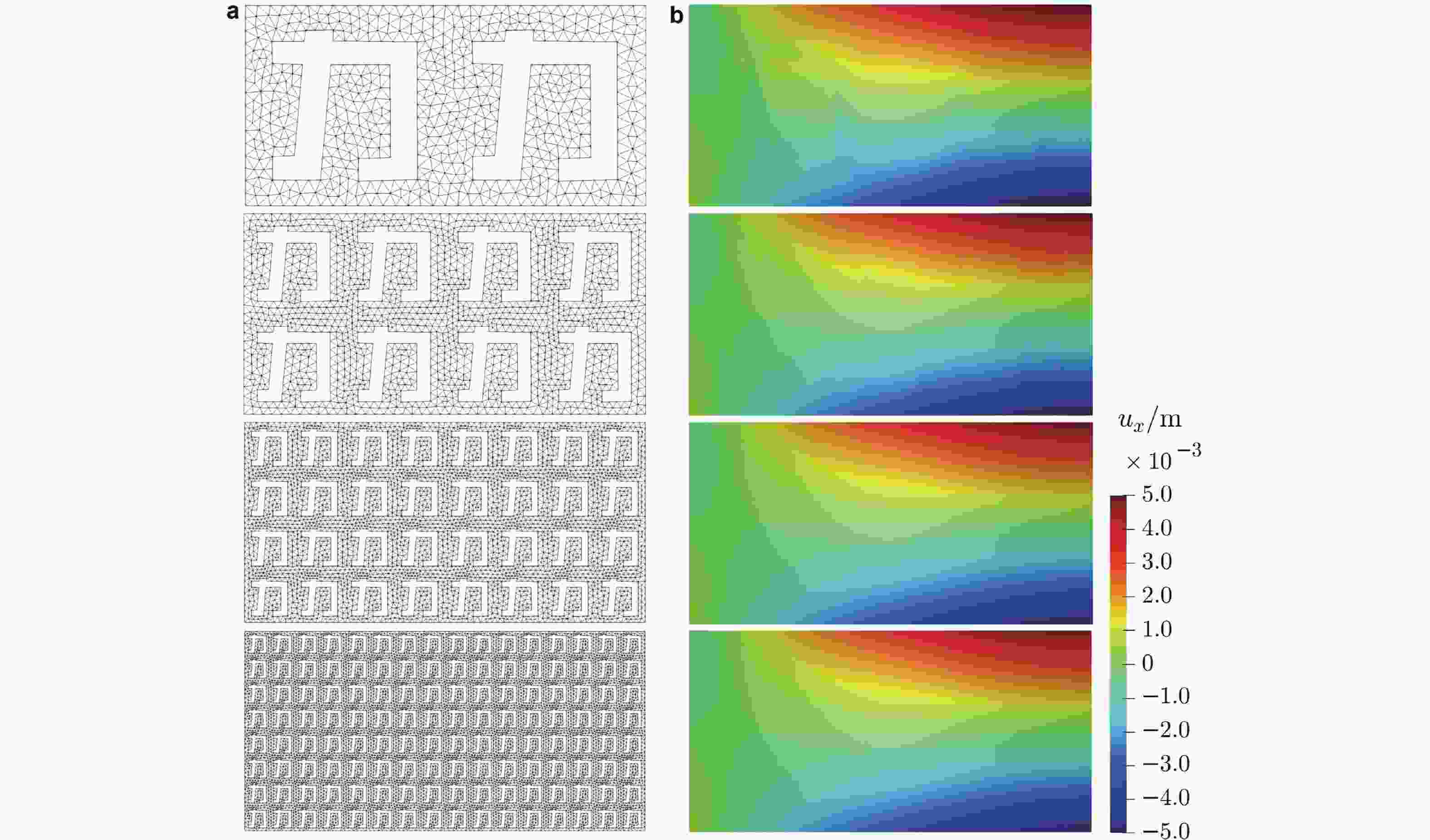
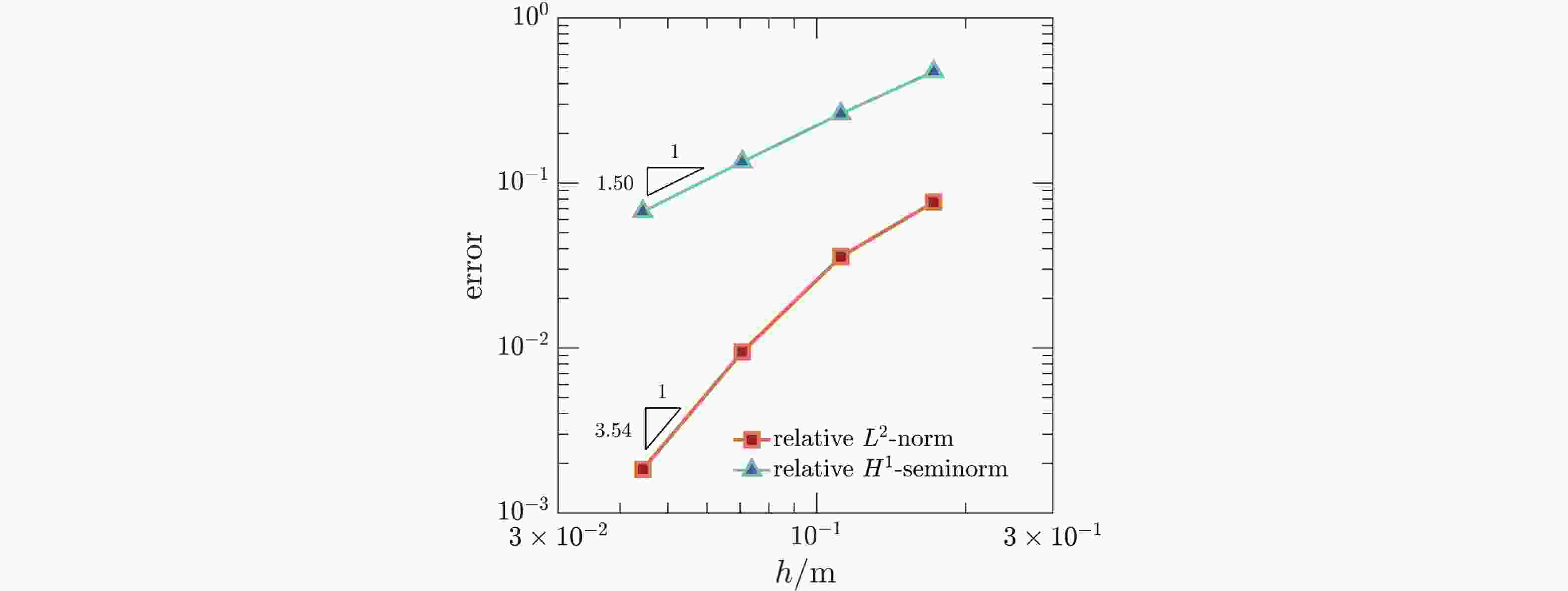
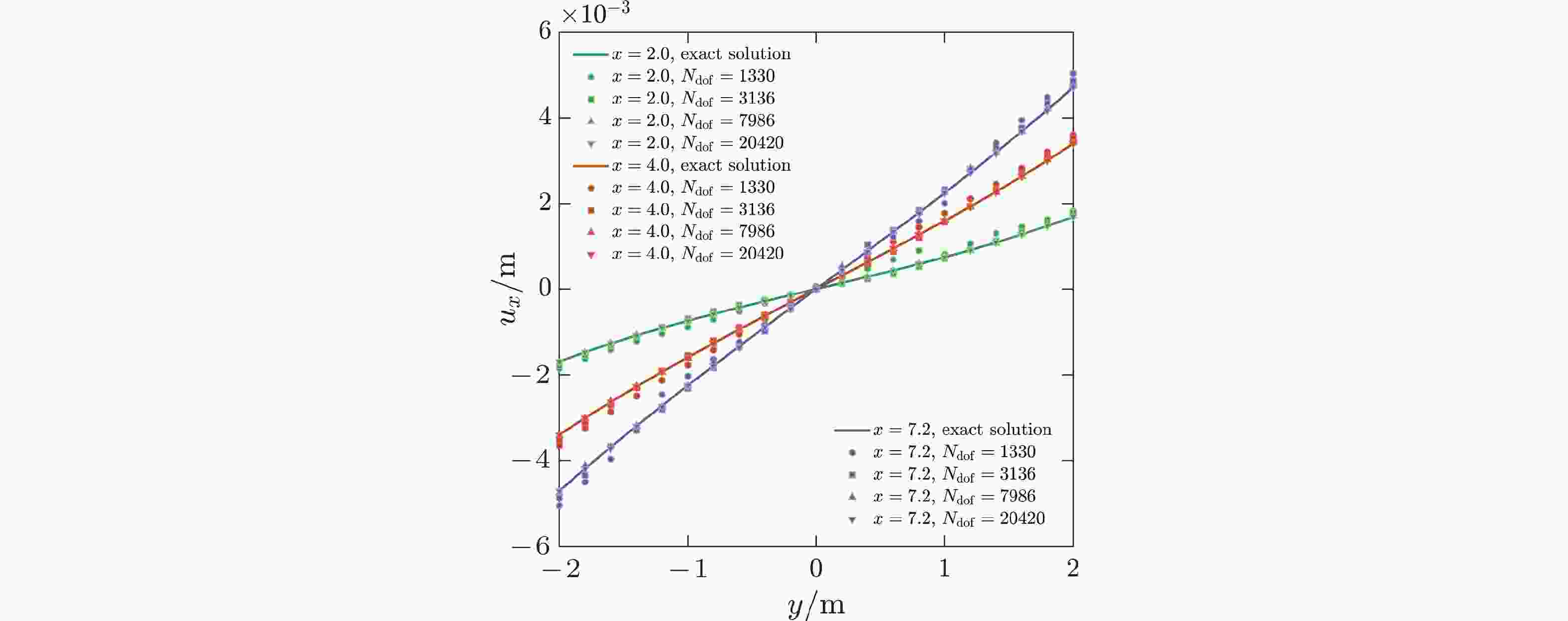
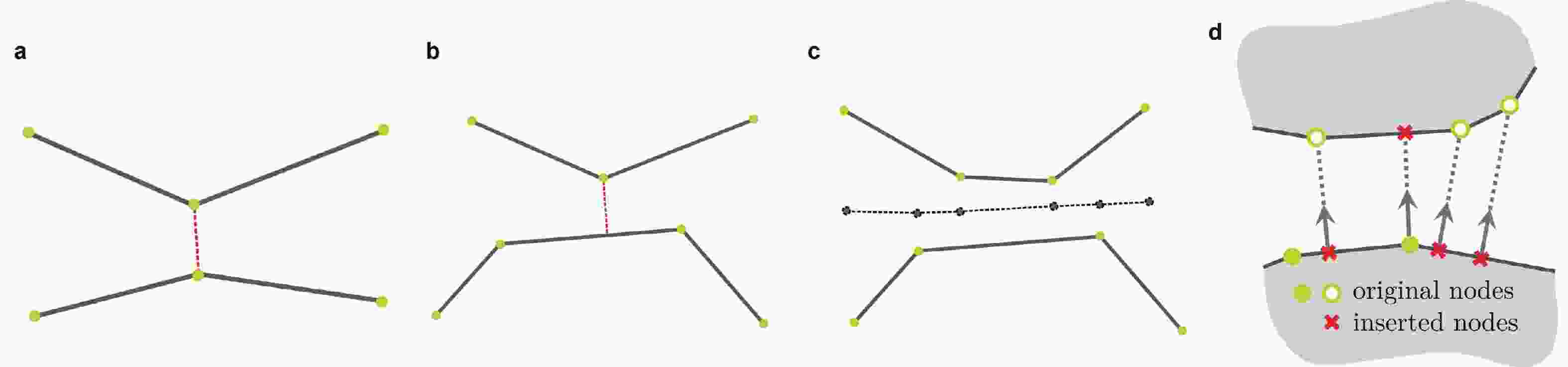
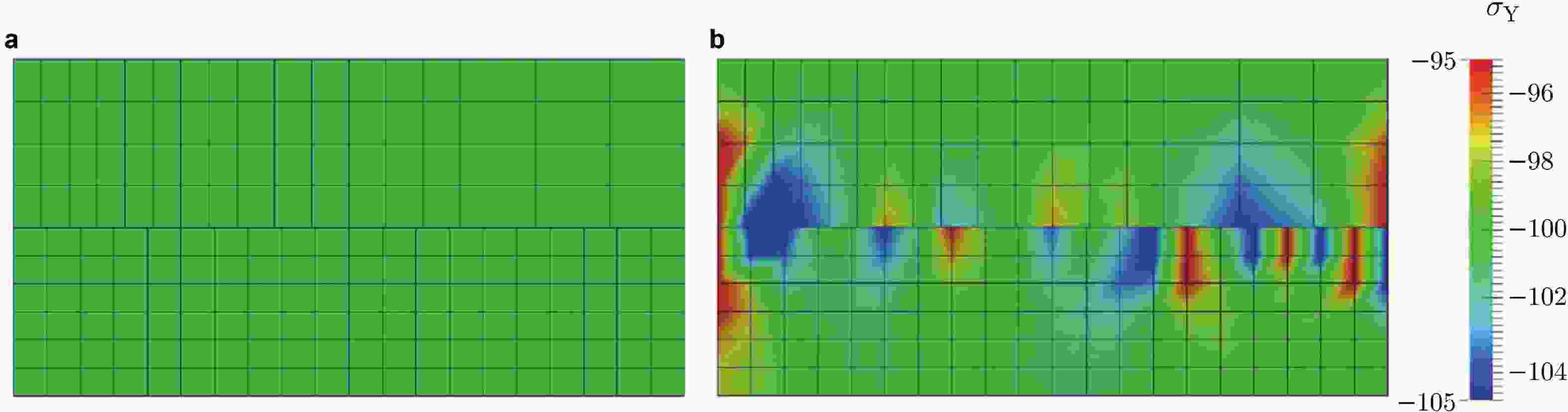
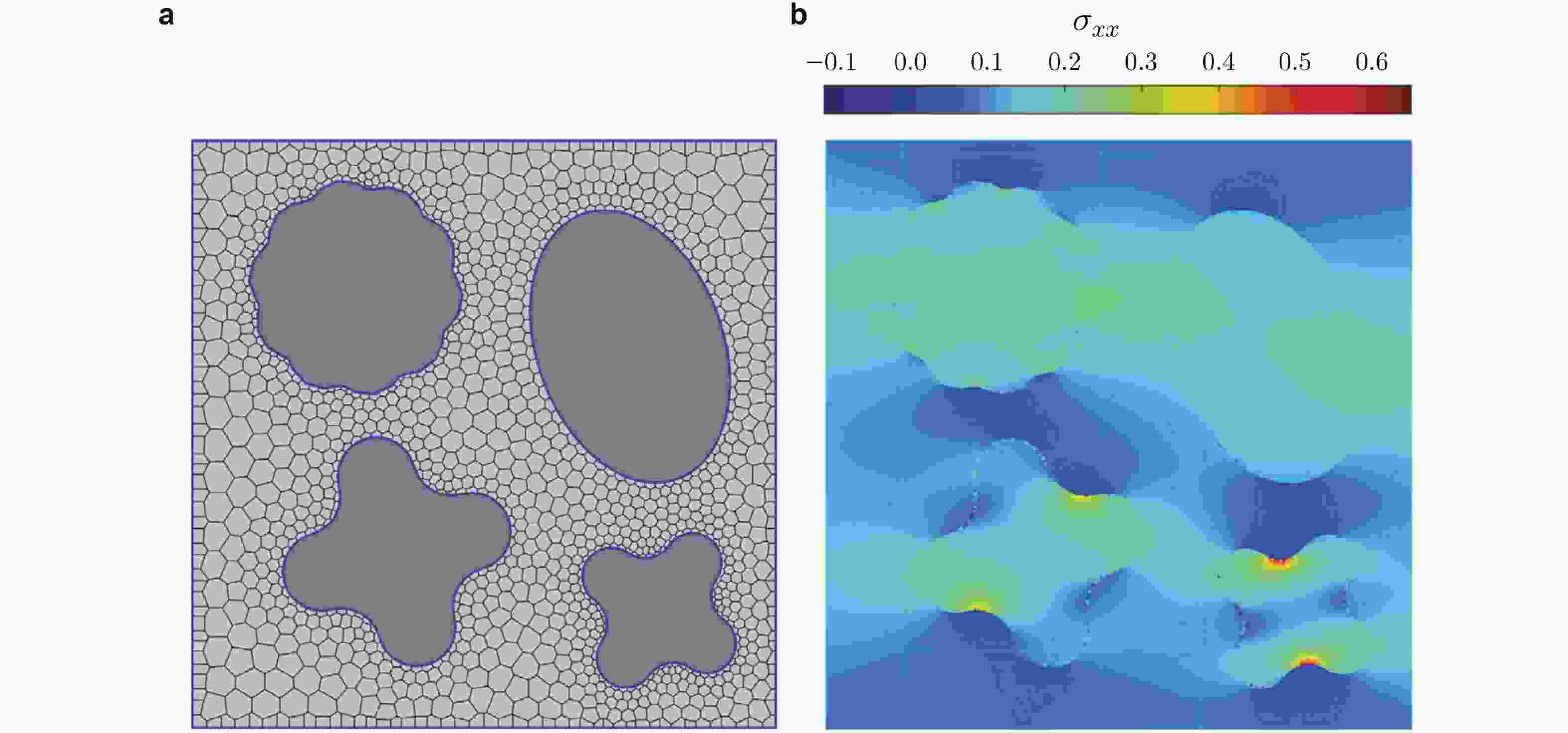
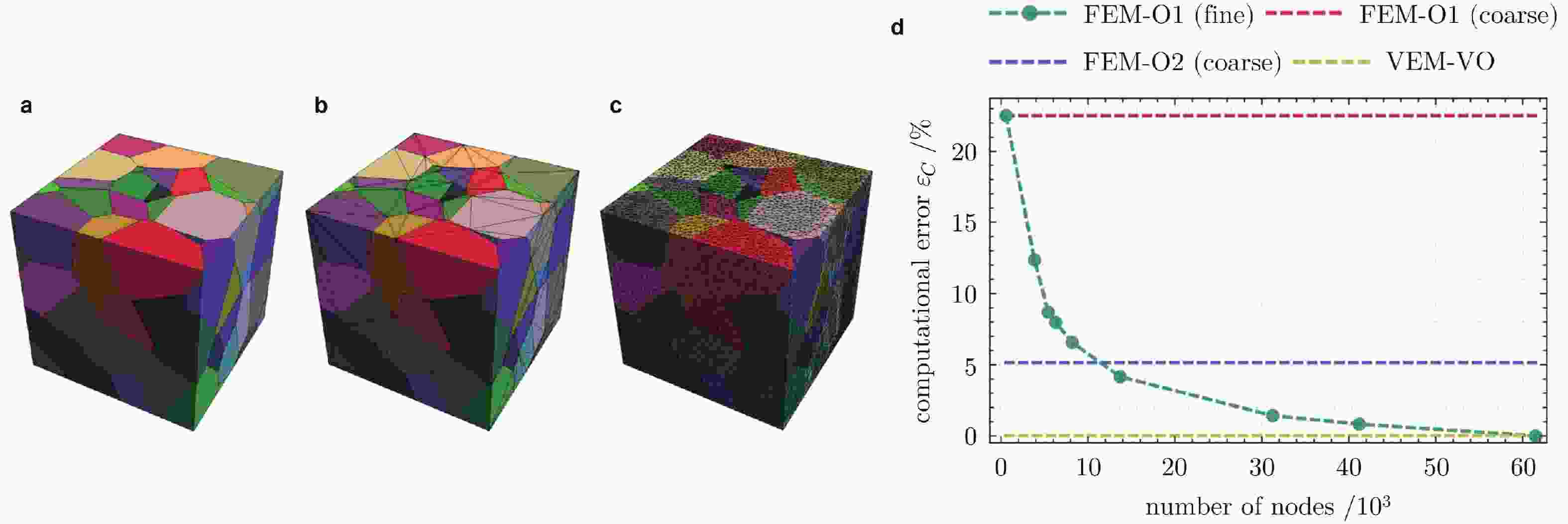
摘要: 虚单元方法是近几年在计算领域迅速发展的一种先进数值方法, 相比于有限元方法, 该方法放松了对单元凸凹性的限制, 可适用于任意形状的多边形单元, 因而在处理悬挂节点、接触、多晶体变形等特定问题方面具有优势, 是当前计算力学领域的国际前沿与热点方向. 本文全面综述了虚单元方法的理论发展, 通过介绍该方法在泊松方程、线弹性、非线性等问题中的应用, 向读者展示了虚单元法的理论核心以及它和有限元方法的异同. 尽管虚单元法的发展目前还处在起步阶段, 但该方法在诸多的非线性问题、接触问题、裂纹扩展以及多场耦合等方面展现出了巨大潜力. 通过对虚单元方法最新理论与应用进展的综述, 为面临单元凸凹性等问题苦恼的计算领域科研工作者提供一种新的解决方案; 同时为对工程科学计算感兴趣的青年科研人员提供关于虚单元方法的快速而系统的全面认知, 以期青年学者能融会贯通, 发展出适应我国计算力学需求的新型算法与高性能软件.
- 虚单元法 /
- 非多项式函数 /
- 工程科学计算 /
- 单元凸凹性
Abstract: Virtual Element Method (VEM) is a recently-developed numerical method suitable for arbitrarily convex or concave cells. This benefits the VEM to handle hanging nodes, contacts and polycrystalline deformations. We here illustrate the theory of the VEM via the Poisson equation and the elastic problem, and summarize its applications to non-linear problems. Compared to the Finite Element Method (FEM), the characteristics of the VEM are explained in details. The VEM has demonstrated potentials to model contacts, cracks, coupling of multiple physics, and etc. We hope that this review can provides an alternative means for software developers in computational mechanics.
- virtual element method /
- non-polynomial function /
- computational mechanics /
- convexity and concavity
注释:

1) $ h $ 一般代表空间离散尺寸, 考虑到单元离散后必对应近似函数空间, 为避免引入过多符号, 直接采用 $ h $ 符号表示近似空间, 且在定义变量时是上标、在定义运算时是下标.

2) 计算数学的微元符号常采用 dx(如上章所述), 本章更侧重于力学解释, 因而体积微元为${\rm{d}} \varOmega $, 界面微元为 ${\rm{d}}\partial \varOmega $

HTML全文

图 1 $ k=2 $ 的单元自由度设定位置($ E $: 单元；$ e $: 单元边界)

下载: 全尺寸图片幻灯片

图 2 二维弹性边值问题

下载: 全尺寸图片幻灯片

图 3 悬臂梁模型

下载: 全尺寸图片幻灯片

图 4 网格与水平向位移场. (a)网格, (b)水平向位移

下载: 全尺寸图片幻灯片

图 5 收敛曲线

下载: 全尺寸图片幻灯片

图 6 不同纵向剖面的水平向位移与理论解比较

下载: 全尺寸图片幻灯片

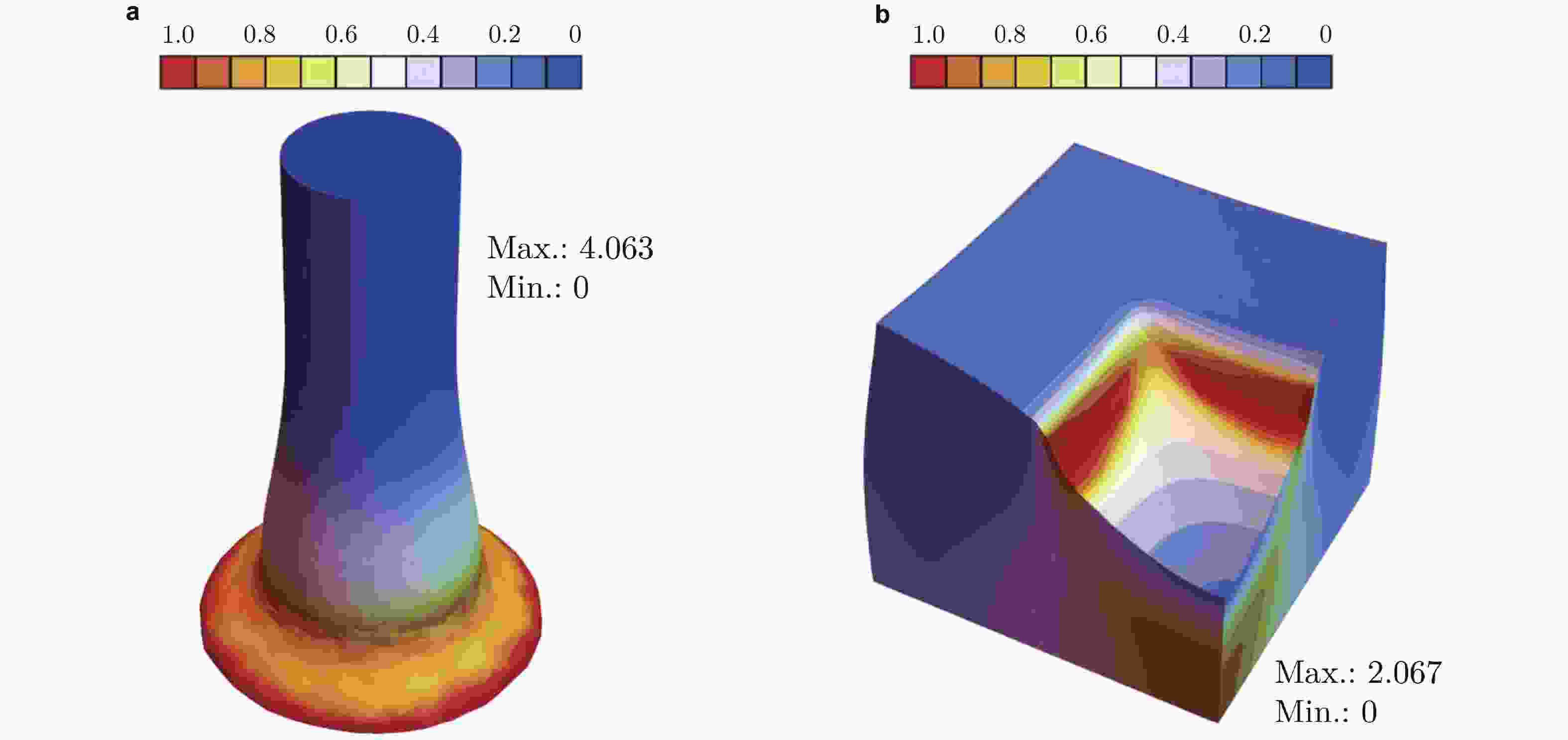
图 7 有限变形下的冲压问题. (a)模型, (b)可压缩材料的冲压变形, (c)不可压缩材料冲压变形(修改自 (Wriggers et al. 2017))

下载: 全尺寸图片幻灯片

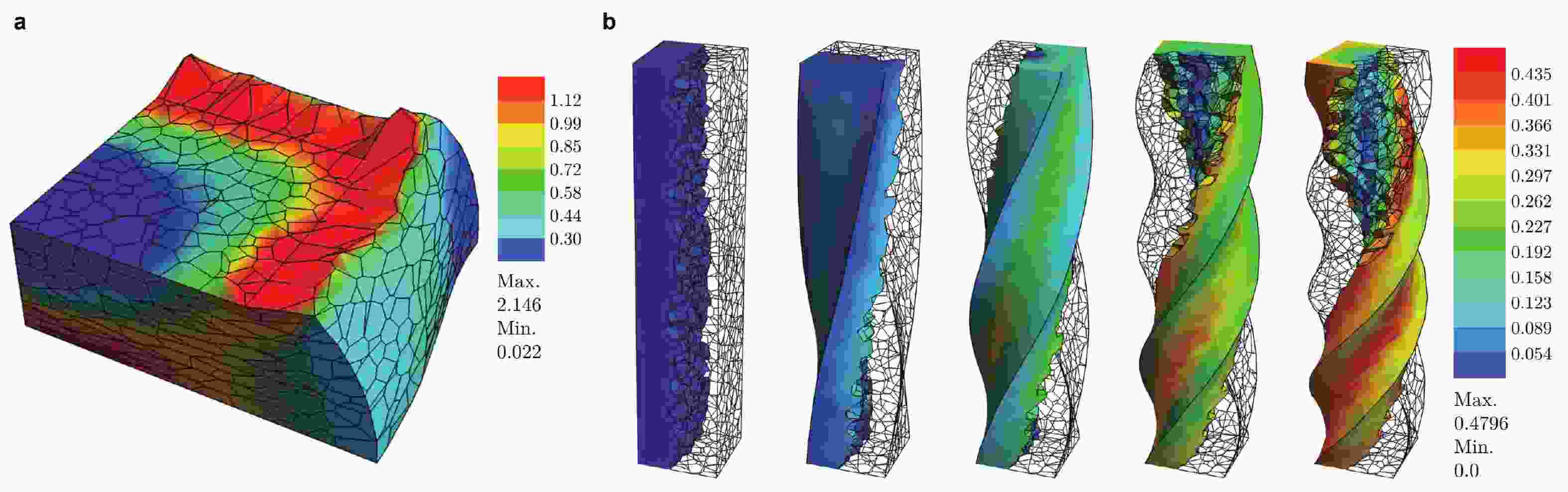
图 8 弹性多孔材料的有限变形. (a)模型, (b)剪应变为 1.345 下的最大应变分布(修改自(Chi et al. 2017))

下载: 全尺寸图片幻灯片

图 9 准静态大变形弹塑性问题的等效塑性应变分布. (a)冲压, (b)扭转 (修改自(Hudobivnik et al. 2019))

下载: 全尺寸图片幻灯片

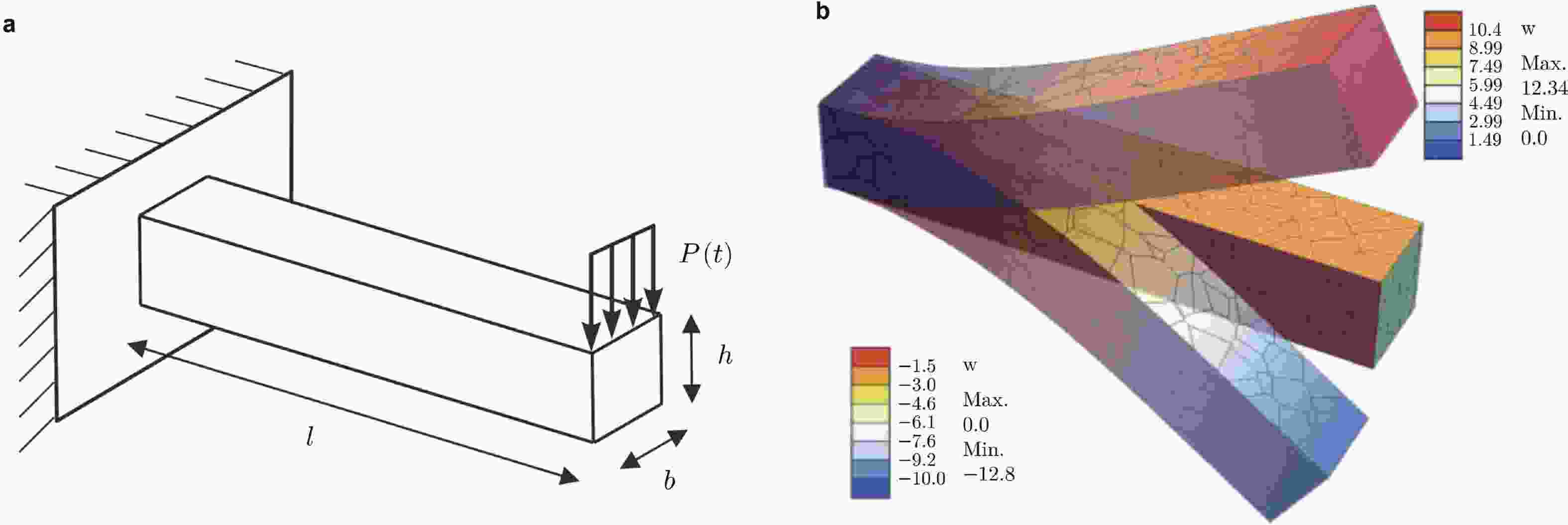
图 10 悬臂梁振动问题. (a)模型, (b)垂向位移时间演化(修改自 (Cihan et al. 2021b))

下载: 全尺寸图片幻灯片

图 11 弹塑性动力学问题的塑性应变. (a)泰勒杆, (b)冲压 (修改自 (Cihan et al. 2021a))

下载: 全尺寸图片幻灯片

图 12 判断两物体间相对位置的常用方法. (a)点−点, (b)点−线, (c)Mortar 型, (d)虚单元法插节点

下载: 全尺寸图片幻灯片

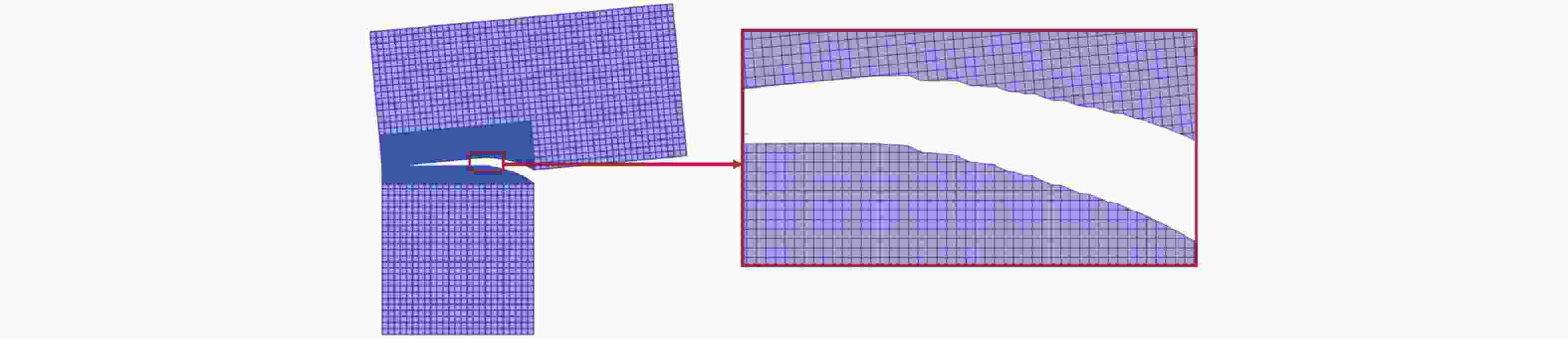
图 13 接触问题的分片测试. (a)虚单元法, (b)有限元点−线格式(修改自(Wriggers et al. 2016))

下载: 全尺寸图片幻灯片

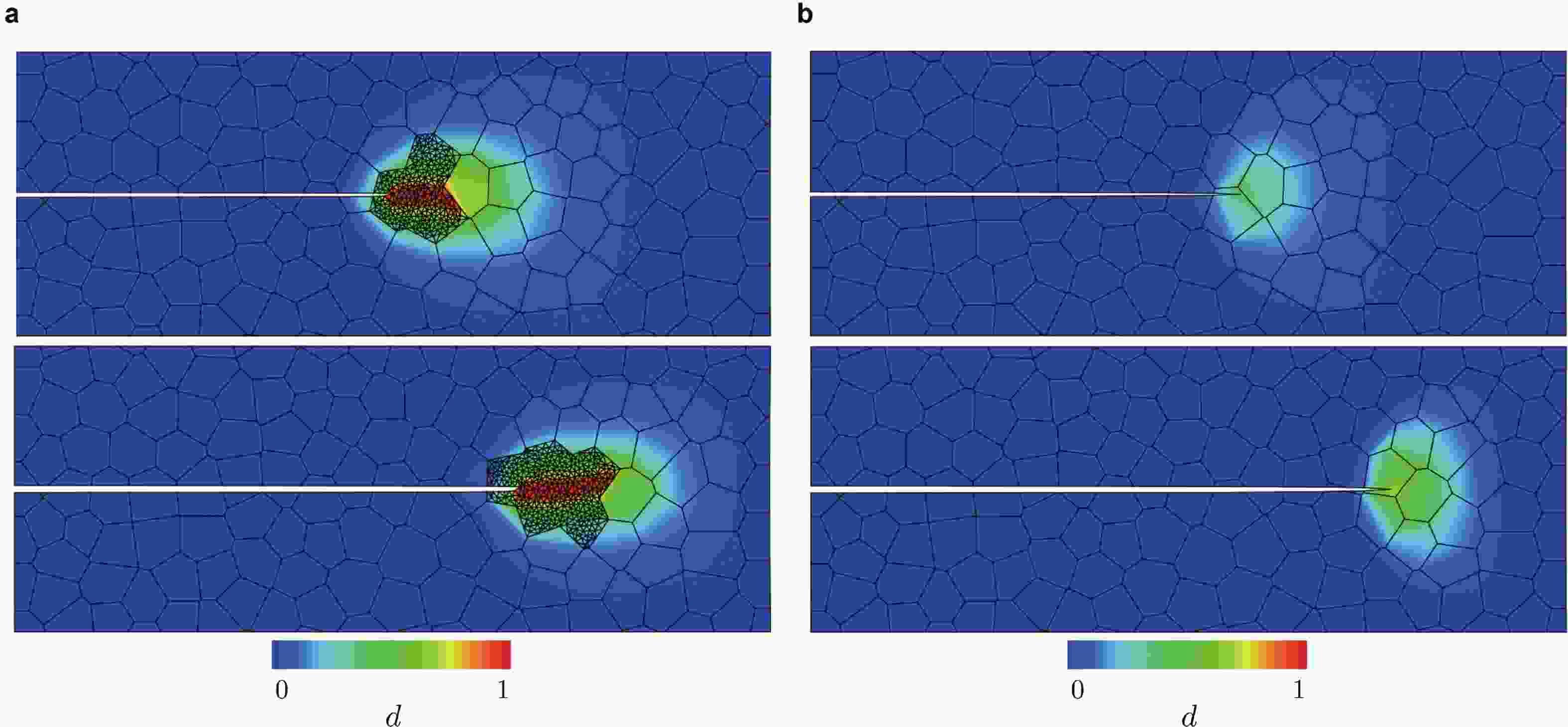
图 14 大变形条件下的赫兹接触问题(修改自 (Wriggers and Rust, 2019))

下载: 全尺寸图片幻灯片

图 15 颗粒材料沙漏过程(修改自 (Gay Neto et al. 2021))

下载: 全尺寸图片幻灯片

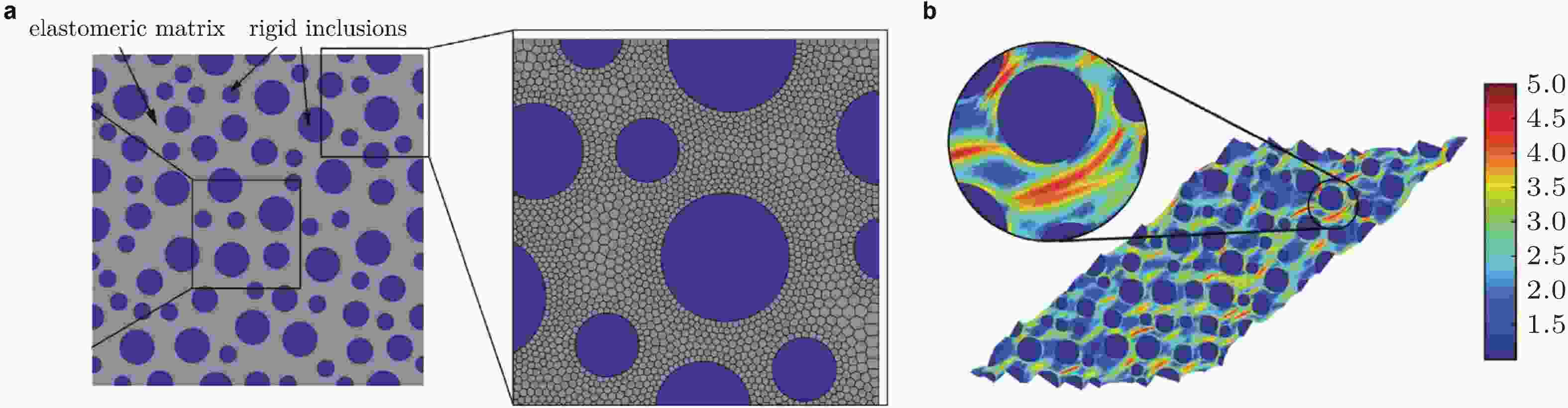
图 16 虚单元法与边界元法模拟非均匀材料. (a)网格, (b)单轴拉伸条件下的应力分布(修改自 (Lo Cascio et al. 2021))

下载: 全尺寸图片幻灯片

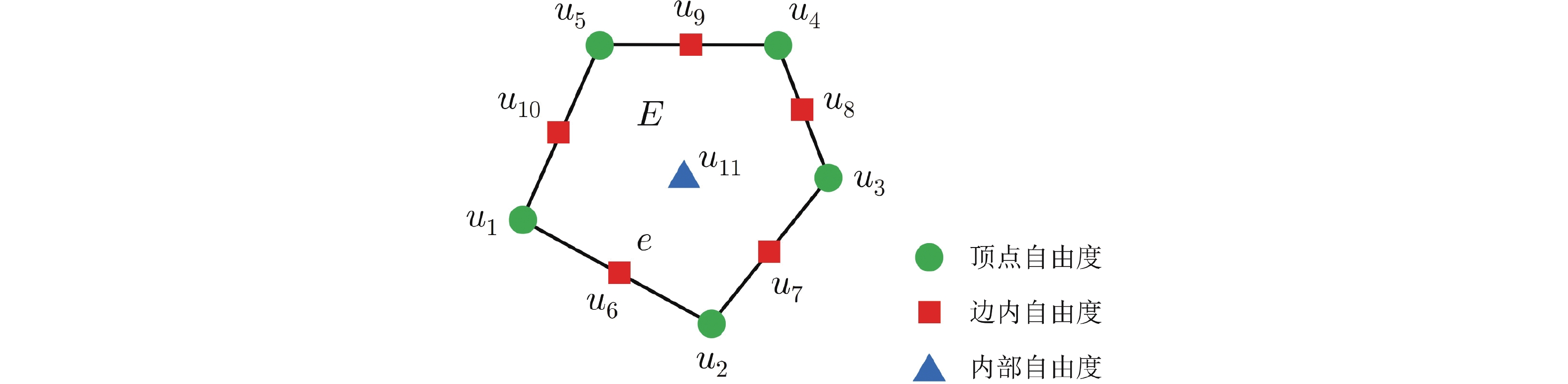
图 17 多晶结构均质化. (a)虚单元法网格, (b)粗糙的四面体网格, (c)精细的四面体网格, (d)$ \mathrm{BaNiO_3} $，中度各向异性，六方晶系晶胞(修改自 (Böhm et al. 2021b))

下载: 全尺寸图片幻灯片

图 18 虚单元法模拟晶体塑性. (a)单个晶格网格分解为界面网格与内部四面体网格, (b)单轴拉伸条件下 FCC 晶格结构的剪应变(修改自 (Böhm et al. 2021a))

下载: 全尺寸图片幻灯片

图 19 虚单元方法分析轴承衬套中不同材料连接区域的多晶塑性模型. (a)轴承衬套示意, (b)三种材料连接区域的代表性体积单元, (c) FCC 晶格在 $ (1 1 1) $ 滑移面上剪切应变率 $ \dot{\gamma}_1 $ 的分布, (d)等效 von Mises 应力分布(修改自 (Behrens et al. 2020))

下载: 全尺寸图片幻灯片

图 20 虚单元法在断裂问题中的应用. (a)单元切割, (b)裂纹扩展(修改自 (Hussein et al. 2019))

下载: 全尺寸图片幻灯片

图 21 单元切割与自适性相场耦合模拟裂纹扩展. (a)自适应网格求解相场, (b)单元切割模拟裂纹扩展(修改自 (Hussein et al. 2020))

下载: 全尺寸图片幻灯片

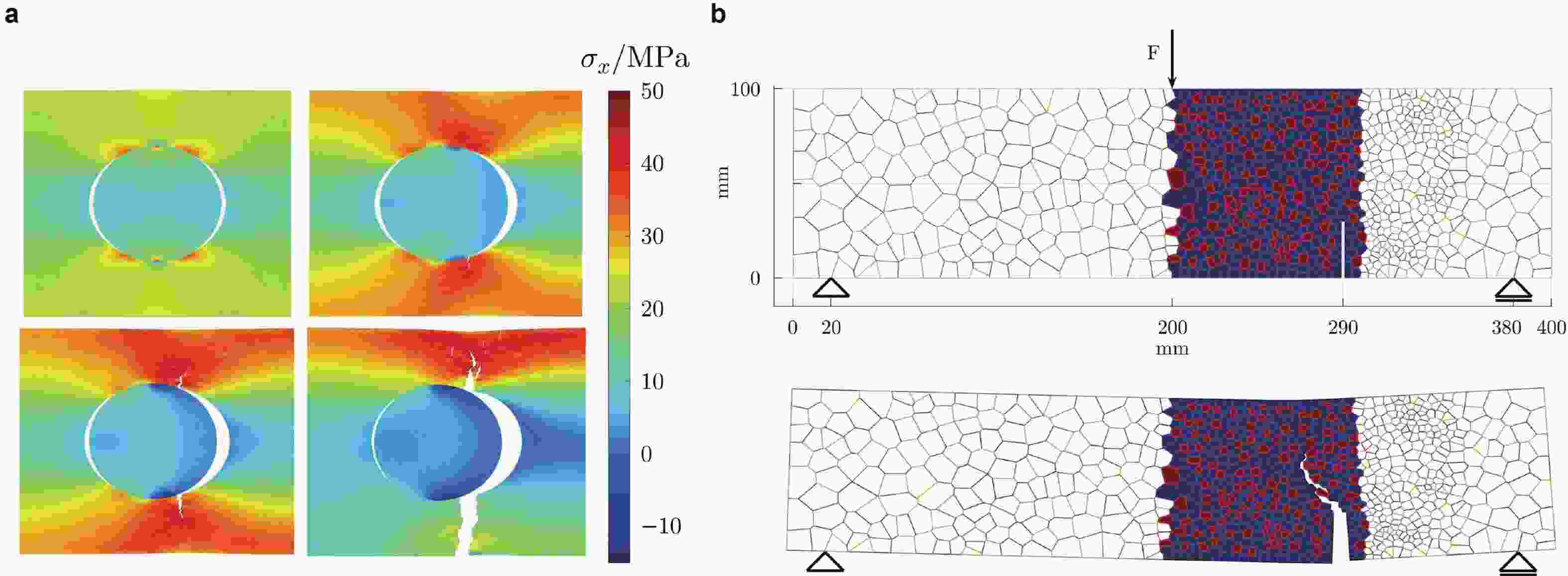
图 22 虚单元法与内聚力模型模拟 L 形状板的裂纹扩展(修改自 (Marfia et al. 2022))

下载: 全尺寸图片幻灯片

图 23 虚单元法与内聚力模型模拟耦合. (a)接触脱离模拟, (b)非均匀材料三点弯曲梁模拟(修改自 (Benedetto et al. 2018))

下载: 全尺寸图片幻灯片

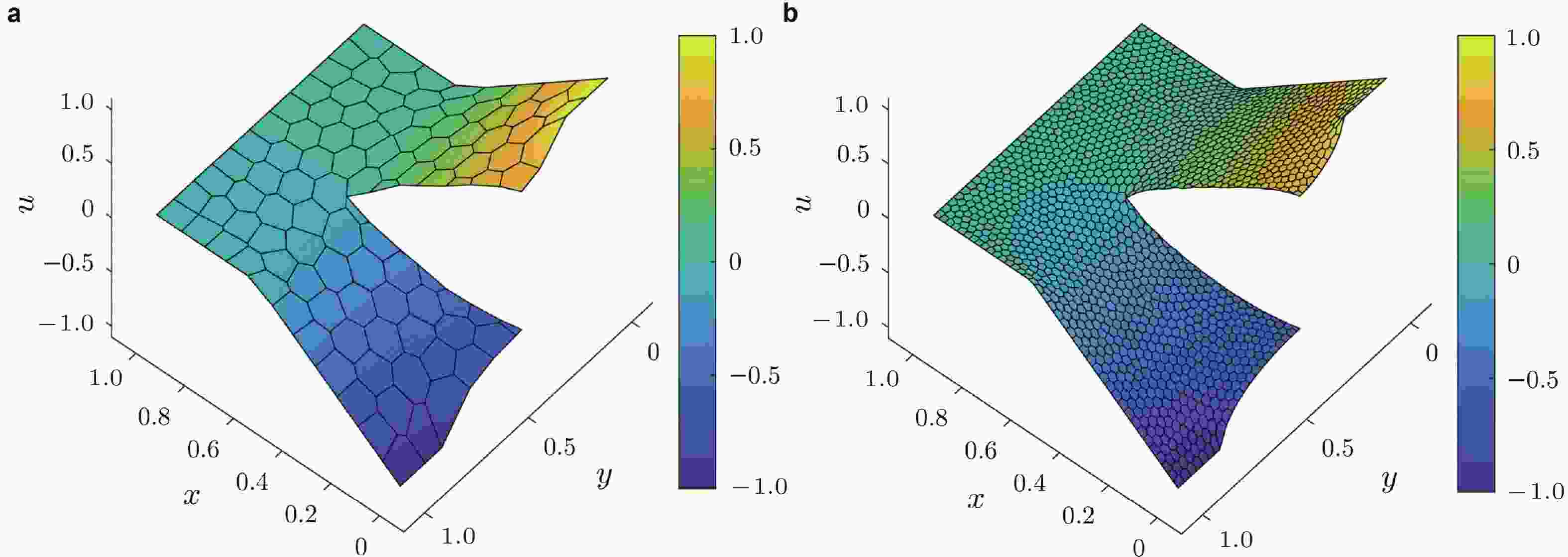
图 24 虚单元法与扩展有限元结合模拟含裂纹的薄膜在 III 型载荷作用下变形. (a)100 个网格, (b)1600个网格(修改自 (Benvenuti et al. 2019))

下载: 全尺寸图片幻灯片

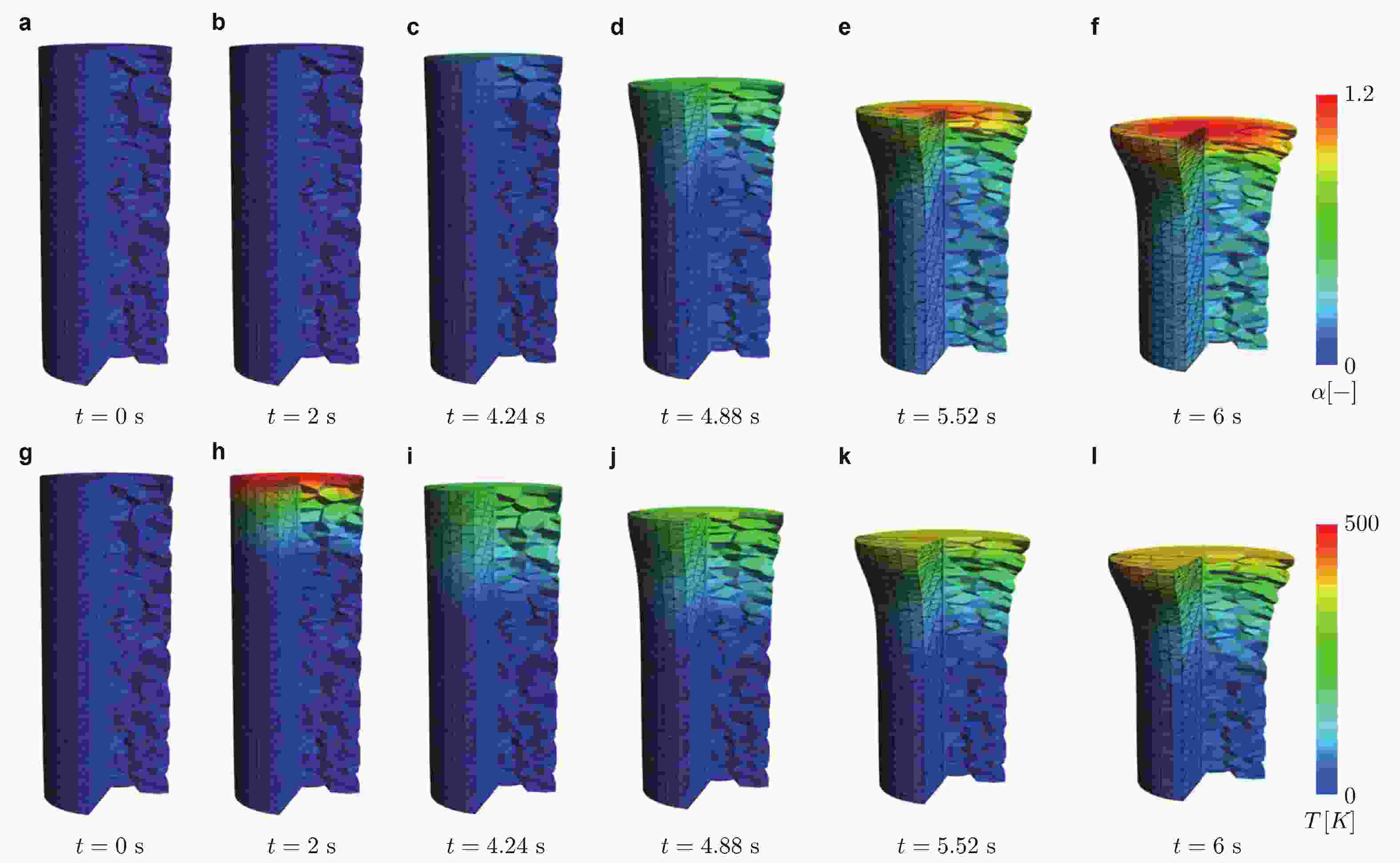
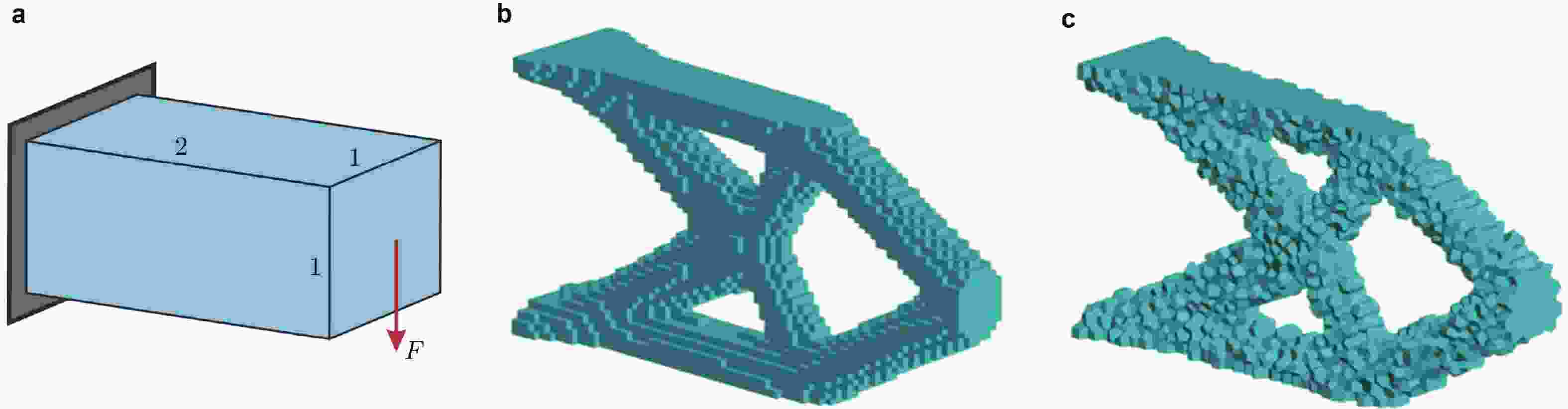
图 25 三维钢螺栓成型的热力耦合过程. (a)~(f)等效塑性应变, (g)~(l)绝对温度场 $ T $ (Aldakheel et al. 2019b)

下载: 全尺寸图片幻灯片

图 26 拓扑优化问题. (a)悬臂梁模型, (b)六面体网格, (c)非规则多面体网格(修改自 (Gain et al. 2015))

下载: 全尺寸图片幻灯片

图 27 拓扑优化问题. (a)目标承载体, (b)优化结果(修改自 (Zhang et al. 2021))

下载: 全尺寸图片幻灯片

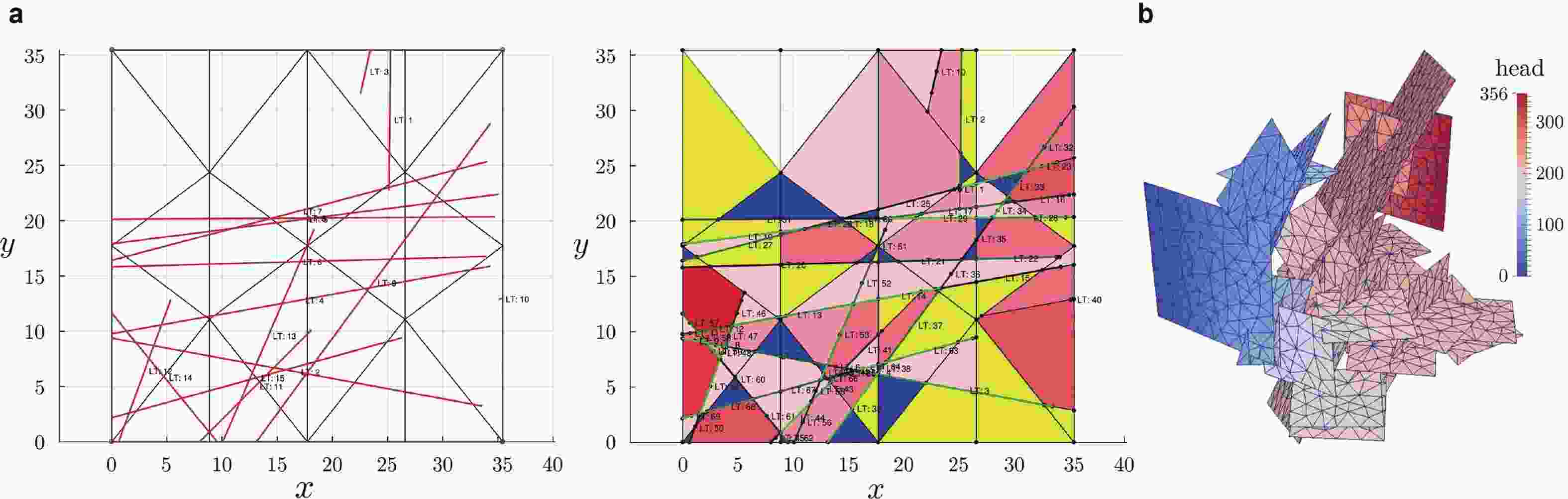
图 28 地下裂隙流体流动问题. (a)网格生成, (b)裂隙网络中水头分布(修改自 (Benedetto et al. 2016))

下载: 全尺寸图片幻灯片

参考文献(58)

Aldakheel F, Hudobivnik B, Artioli E, Beirão da Veiga L, Wriggers P. 2020. Curvilinear virtual elements for contact mechanics. Computer Methods in Applied Mechanics and Engineering, 372: 113394. doi: 10.1016/j.cma.2020.113394

Aldakheel F, Hudobivnik B, Wriggers P. 2019a. Virtual element formulation for phase-field modeling of ductile fracture. International Journal for Multiscale Computational Engineering, 17: 181-200. doi: 10.1615/IntJMultCompEng.2018026804

Aldakheel F, Hudobivnik B, Wriggers P, 2019b. Virtual elements for finite thermo-plasticity problems. Computational Mechanics, 64: 1347–1360.

Artioli E, Beirão da Veiga L, Dassi F. 2020. Curvilinear virtual elements for 2d solid mechanics applications. Computer Methods in Applied Mechanics and Engineering, 359: 112667. doi: 10.1016/j.cma.2019.112667

Artioli E, Beirão da Veiga L, Lovadina C, Sacco E. 2017a. Arbitrary order 2d virtual elements for polygonal meshes: Part i, elastic problem. Computational Mechanics, 60: 355-377. doi: 10.1007/s00466-017-1404-5

Artioli E, Beirão da Veiga L, Lovadina C, Sacco E. 2017b. Arbitrary order 2d virtual elements for polygonal meshes: Part ii, inelastic problem. Computational Mechanics, 60: 643-657. doi: 10.1007/s00466-017-1429-9

Behrens B A, Maier H J, Poll G, Wriggers P, Aldakheel F, Klose C, Nürnberger F, Pape F, Böhm C, Chugreeva A, et al. 2020. Numerical investigations regarding a novel process chain for the production of a hybrid bearing bushing. Production Engineering, 14: 569-581. doi: 10.1007/s11740-020-00992-7

Beirão da Veiga L, Brezzi F, Cangiani A, Manzini G, Marini L D, Russo A. 2013a. Basic principles of virtual element methods. Mathematical Models and Methods in Applied Sciences, 23: 199-214. doi: 10.1142/S0218202512500492

Beirão da Veiga L, Brezzi F, Marini L D. 2013b. Virtual elements for linear elasticity problems. SIAM Journal on Numerical Analysis, 51: 794-812. doi: 10.1137/120874746

Beirão da Veiga L, Brezzi F, Marini L D, Russo A. 2014. The hitchhiker's guide to the virtual element method. Mathematical Models and Methods in Applied Sciences, 24: 1541-1573. doi: 10.1142/S021820251440003X

Beirão da Veiga L, Brezzi F, Marini L D, Russo A. 2016. Serendipity nodal vem spaces. Computers & Fluids, 141: 2-12.

Beirão da Veiga L, Dassi F, Russo A. 2017a. High-order virtual element method on polyhedral meshes. Computers & Mathematics with Applications, 74: 1110-1122.

Beirão da Veiga L, Lovadina C, Mora D. 2015. A virtual element method for elastic and inelastic problems on polytope meshes. Computer Methods in Applied Mechanics and Engineering, 295: 327-346. doi: 10.1016/j.cma.2015.07.013

Beirão da Veiga L, Lovadina C, Russo A. 2017b. Stability analysis for the virtual element method. Mathematical Models and Methods in Applied Sciences, 27: 2557-2594. doi: 10.1142/S021820251750052X

Beirão da Veiga L, Russo A, Vacca G. 2019. The virtual element method with curved edges. ESAIM: Mathematical Modelling and Numerical Analysis, 53: 375-404. doi: 10.1051/m2an/2018052

Benedetto M F, Berrone S, Borio A, Pieraccini S, Scialò S. 2016. A hybrid mortar virtual element method for discrete fracture network simulations. Journal of Computational Physics, 306: 148-166. doi: 10.1016/j.jcp.2015.11.034

Benedetto M F, Borio A, Scialò S. 2017. Mixed virtual elements for discrete fracture network simulations. Finite Elements in Analysis and Design, 134: 55-67. doi: 10.1016/j.finel.2017.05.011

Benedetto M F, Caggiano A, Etse G. 2018. Virtual elements and zero thickness interface-based approach for fracture analysis of heterogeneous materials. Computer Methods in Applied Mechanics and Engineering, 338: 41-67. doi: 10.1016/j.cma.2018.04.001

Benvenuti E, Chiozzi A, Manzini G, Sukumar N. 2019. Extended virtual element method for the laplace problem with singularities and discontinuities. Computer Methods in Applied Mechanics and Engineering, 356: 571-597. doi: 10.1016/j.cma.2019.07.028

Benvenuti E, Chiozzi A, Manzini G, Sukumar N. 2022. Extended virtual element method for two-dimensional linear elastic fracture. Computer Methods in Applied Mechanics and Engineering, 390: 114352. doi: 10.1016/j.cma.2021.114352

Böhm C, Hudobivnik B, Aldakheel F, Wriggers P. 2021a. Modeling of single-slip finite strain crystal plasticity via the virtual element method. Proc. Appl. Math. Mech., 20: e202000205.

Böhm C, Hudobivnik B, Marino M, Wriggers P. 2021b. Electro-magneto-mechanically response of polycrystalline materials: Computational homogenization via the virtual element method. Computer Methods in Applied Mechanics and Engineering, 380: 113775. doi: 10.1016/j.cma.2021.113775

Braess D. 2007. Finite elements: Theory, fast solvers, and applications in solid mechanics. Cambridge University Press

Brenner S C, Scott L R. 2008. The mathematical theory of finite element methods. volume 15 of Texts in Applied Mathematics. Springer New York

Chi H, Beirão da Veiga L, Paulino G. 2017. Some basic formulations of the virtual element method (vem) for finite deformations. Computer Methods in Applied Mechanics and Engineering, 318: 148-192. doi: 10.1016/j.cma.2016.12.020

Cihan M, Hudobivnik B, Aldakheel F, Wriggers P. 2021a. 3d mixed virtual element formulation for dynamic elasto-plastic analysis. Computational Mechanics, 68: 1-18.

Cihan M, Hudobivnik B, Aldakheel F, Wriggers P. 2021b. Virtual element formulation for finite strain elastodynamics. Computer Modeling in Engineering & Sciences, 129: 1151-1180.

De Bellis M L, Wriggers P, Hudobivnik B. 2019. Serendipity virtual element formulation for nonlinear elasticity. Computers & Structures, 223: 106094.

Dhanush V, Natarajan S. 2019. Implementation of the virtual element method for coupled thermo-elasticity in abaqus. Numerical Algorithms, 80: 1037-1058. doi: 10.1007/s11075-018-0516-0

Gain A L, Paulino G H, Duarte L S, Menezes I F M. 2015. Topology optimization using polytopes. Computer Methods in Applied Mechanics and Engineering, 293: 411-430. doi: 10.1016/j.cma.2015.05.007

Gain A L, Talischi C, Paulino G H. 2014. On the virtual element method for three-dimensional linear elasticity problems on arbitrary polyhedral meshes. Computer Methods in Applied Mechanics and Engineering, 282: 132-160. doi: 10.1016/j.cma.2014.05.005

Gay Neto A, Hudobivnik B, Moherdaui T F, Wriggers P. 2021. Flexible polyhedra modeled by the virtual element method in a discrete element context. Computer Methods in Applied Mechanics and Engineering, 387: 114163. doi: 10.1016/j.cma.2021.114163

Hudobivnik B, Aldakheel F, Wriggers P. 2019. A low order 3d virtual element formulation for finite elasto–plastic deformations. Computational Mechanics, 63: 253-269. doi: 10.1007/s00466-018-1593-6

Hussein A, Aldakheel F, Hudobivnik B, Wriggers P, Guidault P A, Allix O. 2019. A computational framework for brittle crack-propagation based on efficient virtual element method. Finite Elements in Analysis and Design, 159: 15-32. doi: 10.1016/j.finel.2019.03.001

Hussein A, Hudobivnik B, Aldakheel F, Wriggers P, Guidault P A, Allix O. 2018. A virtual element method for crack propagation. Proc. Appl. Math. Mech., 18: e201800104.

Hussein A, Hudobivnik B, Wriggers P. 2020. A combined adaptive phase field and discrete cutting method for the prediction of crack paths. Computer Methods in Applied Mechanics and Engineering, 372: 113329. doi: 10.1016/j.cma.2020.113329

Lo Cascio M, Milazzo A, Benedetti I. 2021. A hybrid virtual–boundary element formulation for heterogeneous materials. International Journal of Mechanical Sciences, 199: 106404. doi: 10.1016/j.ijmecsci.2021.106404

Marfia S, Monaldo E, Sacco E. 2022. Cohesive fracture evolution within virtual element method. Engineering Fracture Mechanics, 269: 108464. doi: 10.1016/j.engfracmech.2022.108464

Marino M, Hudobivnik B, Wriggers P. 2019. Computational homogenization of polycrystalline materials with the virtual element method. Computer Methods in Applied Mechanics and Engineering, 355: 349-372. doi: 10.1016/j.cma.2019.06.004

Mengolini M, Benedetto M F, Aragón A M. 2019. An engineering perspective to the virtual element method and its interplay with the standard finite element method. Computer Methods in Applied Mechanics and Engineering, 350: 995-1023. doi: 10.1016/j.cma.2019.02.043

Park K, Chi H, Paulino G H. 2019. On nonconvex meshes for elastodynamics using virtual element methods with explicit time integration. Computer Methods in Applied Mechanics and Engineering, 356: 669-684. doi: 10.1016/j.cma.2019.06.031

Park K, Chi H, Paulino G H. 2020. Numerical recipes for elastodynamic virtual element methods with explicit time integration. International Journal for Numerical Methods in Engineering, 121: 1-31. doi: 10.1002/nme.6173

Ralston A, Rabinowitz P. 2001. A first course in numerical analysis. Courier Corporation

Reddy B D, van Huyssteen D. 2019. A virtual element method for transversely isotropic elasticity. Computational Mechanics, 64: 971-988. doi: 10.1007/s00466-019-01690-7

Taylor R L, Simo J C, Zienkiewicz O C, Chan A C H. 1986. The patch test—a condition for assessing fem convergence. International Journal for Numerical Methods in Engineering, 22: 39-62. doi: 10.1002/nme.1620220105

Timoshenko S P, Goodier J N. 1951. Theory of Elasticity. McGraw-Hill Book Company

van Huyssteen D, Reddy B. 2021. A virtual element method for transversely isotropic hyperelasticity. Computer Methods in Applied Mechanics and Engineering, 386: 114108. doi: 10.1016/j.cma.2021.114108

Wriggers P, De Bellis M, Hudobivnik B. 2021a. A taylor–hood type virtual element formulations for large incompressible strains. Computer Methods in Applied Mechanics and Engineering, 385: 114021. doi: 10.1016/j.cma.2021.114021

Wriggers P, Hudobivnik B. 2017. A low order virtual element formulation for finite elasto-plastic deformations. Computer Methods in Applied Mechanics and Engineering, 327: 459-477. doi: 10.1016/j.cma.2017.08.053

Wriggers P, Hudobivnik B, Aldakheel F. 2020. A virtual element formulation for general element shapes. Computational Mechanics, 66: 963-977. doi: 10.1007/s00466-020-01891-5

Wriggers P, Hudobivnik B, Aldakheel F. 2021b. Nurbs-based geometries: A mapping approach for virtual serendipity elements. Computer Methods in Applied Mechanics and Engineering, 378: 113732. doi: 10.1016/j.cma.2021.113732

Wriggers P, Hudobivnik B, Korelc J. 2018a. Efficient low order virtual elements for anisotropic materials at finite strains, in: Advances in Computational Plasticity: A Book in Honour of D. Roger J. Owen. Springer International Publishing. Computational Methods in Applied Sciences, pp. 417–434

Wriggers P, Hudobivnik B, Schröder J. 2018b. Finite and virtual element formulations for large strain anisotropic material with inextensive fibers, in: Multiscale Modeling of Heterogeneous Structures. Springer International Publishing. Lecture Notes in Applied and Computational Mechanics, pp. 205–231

Wriggers P, Reddy B D, Rust W, Hudobivnik B. 2017. Efficient virtual element formulations for compressible and incompressible finite deformations. Computational Mechanics, 60: 253-268. doi: 10.1007/s00466-017-1405-4

Wriggers P, Rust W T. 2019. A virtual element method for frictional contact including large deformations. Engineering Computations, 36: 2133-2161. doi: 10.1108/EC-02-2019-0043

Wriggers P, Rust W T, Reddy B D. 2016. A virtual element method for contact. Computational Mechanics, 58: 1039-1050. doi: 10.1007/s00466-016-1331-x

Zhang X S, Chi H, Paulino G H. 2020. Adaptive multi-material topology optimization with hyperelastic materials under large deformations: A virtual element approach. Computer Methods in Applied Mechanics and Engineering, 370: 112976. doi: 10.1016/j.cma.2020.112976

Zhang X S, Chi H, Zhao Z. 2021. Topology optimization of hyperelastic structures with anisotropic fiber reinforcement under large deformations. Computer Methods in Applied Mechanics and Engineering, 378: 113496. doi: 10.1016/j.cma.2020.113496

施引文献

资源附件(0)

访问统计