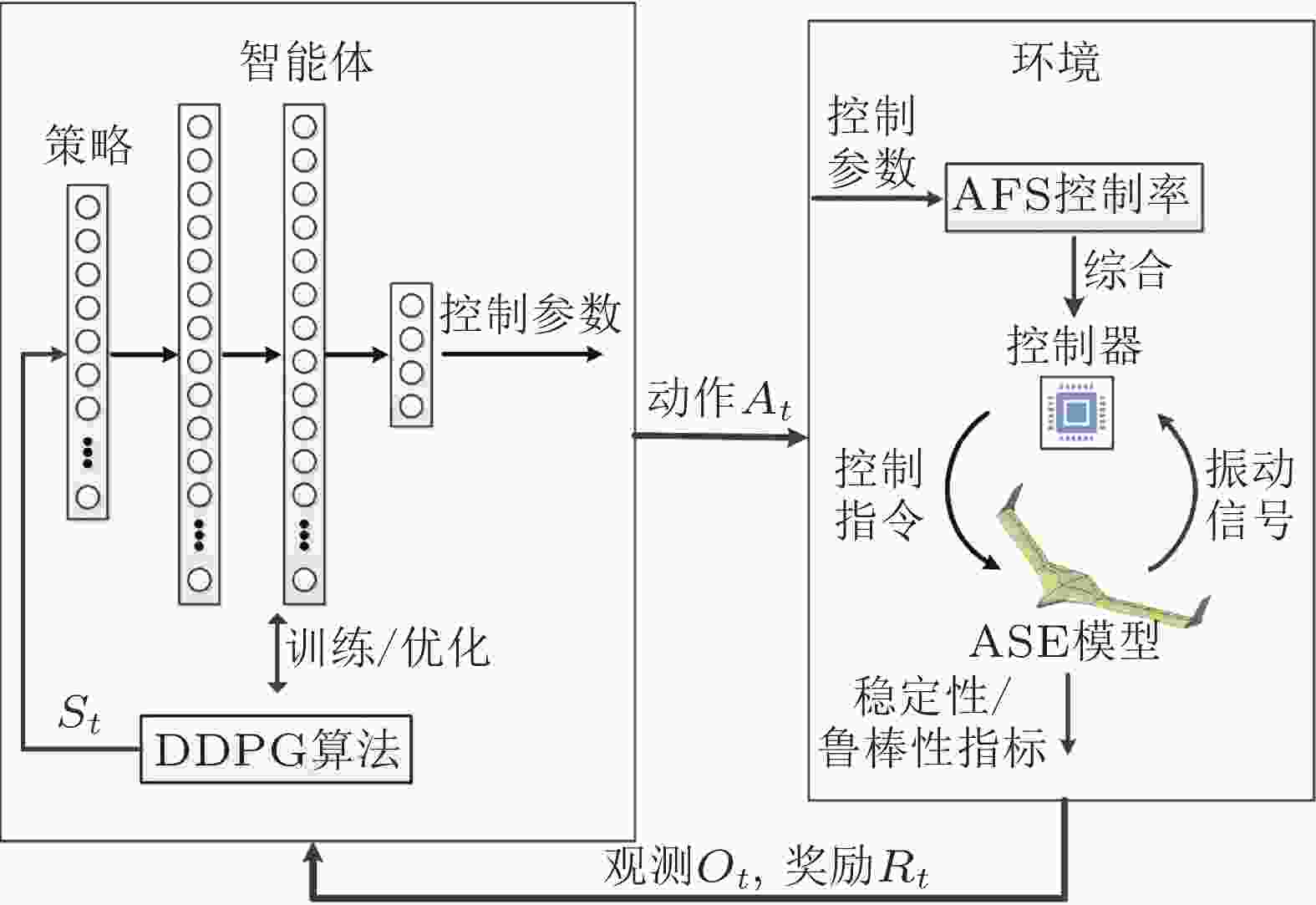
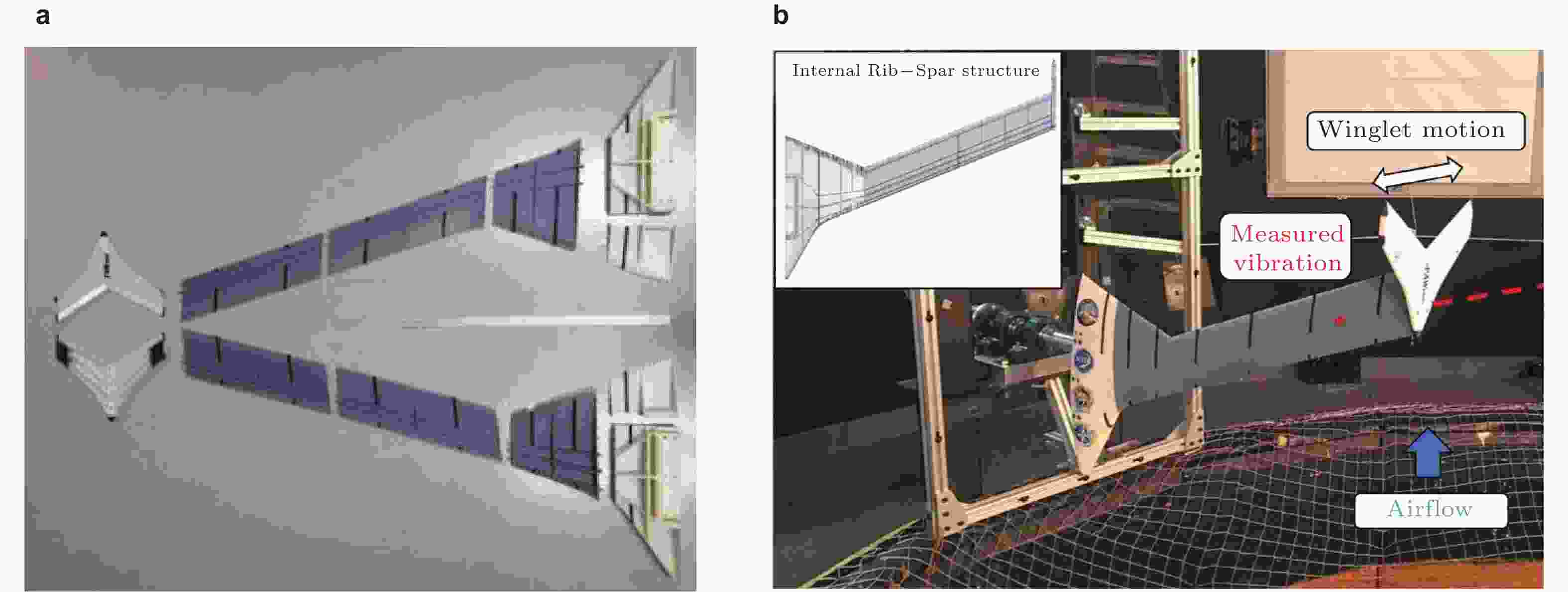
-
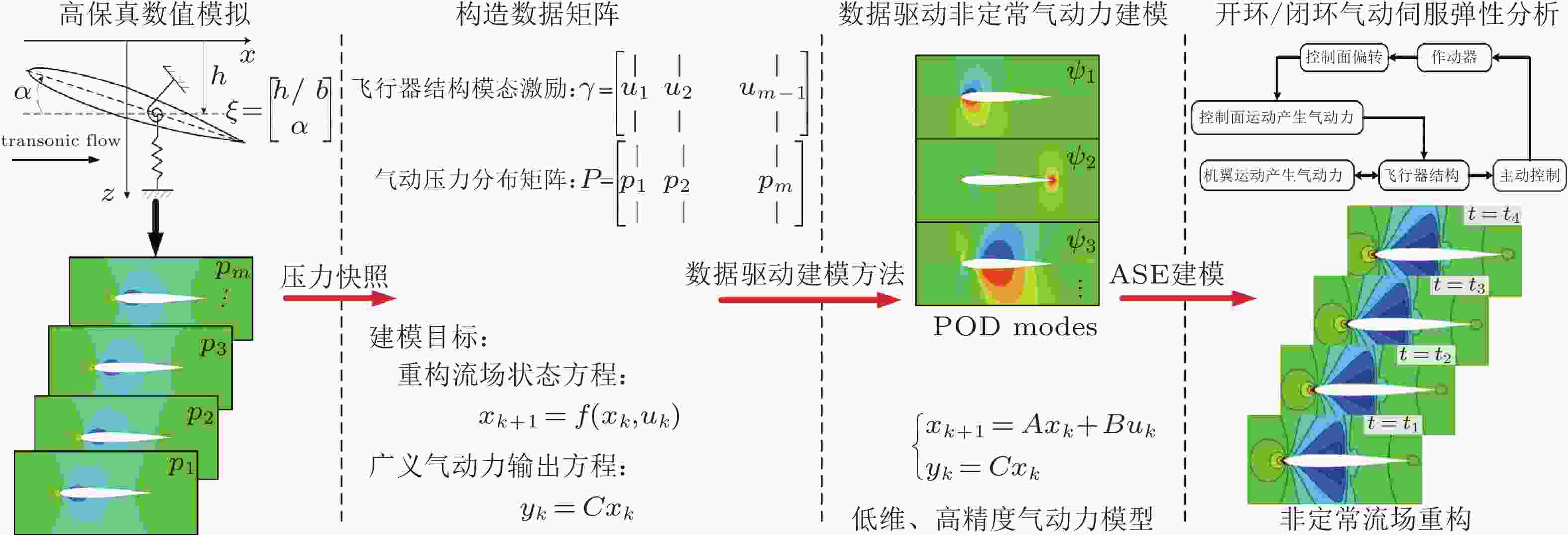
摘要: 现代飞行器日益呈现结构轻质化、控制系统宽通带和高权限的发展趋势. 因此, 非定常气动力、柔性结构和主动控制系统三者间的耦合力学成为重要的研究领域. 自20世纪80年代起, 航空界开始关注受控飞行器的气动弹性稳定性以及主动控制问题, 但对气动/结构的非线性效应、控制回路时滞对受控飞行器动力学行为的影响规律研究尚不充分. 研究这些影响规律不仅涉及非线性、高维数、多变参数和时滞效应等难题, 而且必须面对空气动力、飞行器结构、驱动机构、控制系统之间的强耦合问题. 其中的前沿难题是: 发展非线性气动伺服弹性动力学建模理论, 揭示上述因素诱发受控气动弹性振动的动力学机理, 开展气动伺服弹性控制风洞实验. 本文针对非线性气动伺服弹性力学所涉及的非线性非定常气动力建模、非线性结构动力学、气动伺服弹性控制律设计、气动伺服弹性实验, 总结相关研究现状和最新进展, 特别是近年来作者学术团队的研究成果, 并对进一步研究给出若干建议.Abstract: Advanced flight vehicles have been requiring lightweight structures and the expansion of bandwidth and authority of control systems. Hence, the coupled dynamics of the unsteady aerodynamics, the flexible aircraft structure, and the active control system have been an important research field in dynamics and control. The community of aeronautical technology has paid much attention to the aeroelastic stability and active control of aircraft since the 1980s, but has made less effort to study the effects of the aerodynamic and structural nonlinearities, as well as time delays in a control loop, on the aeroservoelastic behaviors of aircraft. The studies of these effects need to model the high-dimensional and parametric-varying dynamic systems with strong aerodynamic/structural nonlinearity, and hence, face with the coupling among unsteady aerodynamics, aircraft structure, and active control system. The cutting-edge problems include how to develop nonlinear aeroservoelastic modeling theory, how to reveal the dynamic mechanism behind the induced aeroelastic vibrations and how to carry out wind tunnel tests for aeroservoelasticity. This review article surveys the recent advances in reduced modeling of unsteady aerodynamics, nonlinear structural dynamics, design of aeroservoelastic control law, and experimental studies on aeroservoelastic systems, with an emphasis on the researches of the authors’ team in nonlinear aeroservoelasticity. The article also makes a number of suggestions for studies in the future.
-
Key words:
- dynamics /
- active control /
- aeroservoelasticity /
- active flutter suppression
-
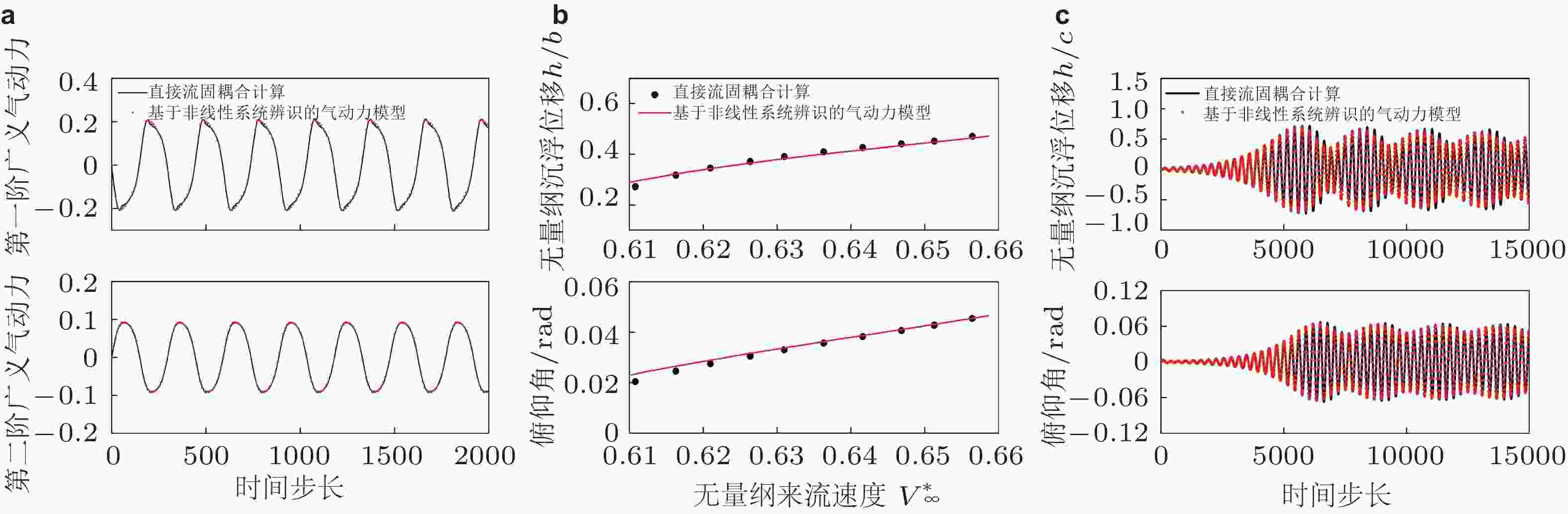
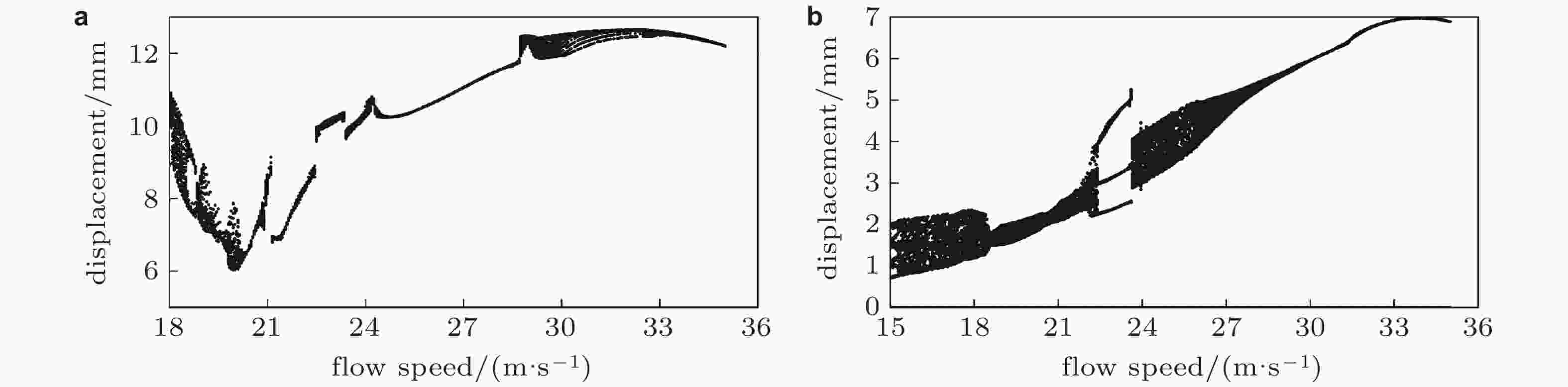
图 3 基于非线性系统辨识的跨声速气动弹性分析. (a) 大幅值激励下的非定常广义力响应, (b) 非线性气动力诱发的极限环振荡, (c) 非线性气动力诱发的机翼“拍振”现象 (Yang et al. 2020)
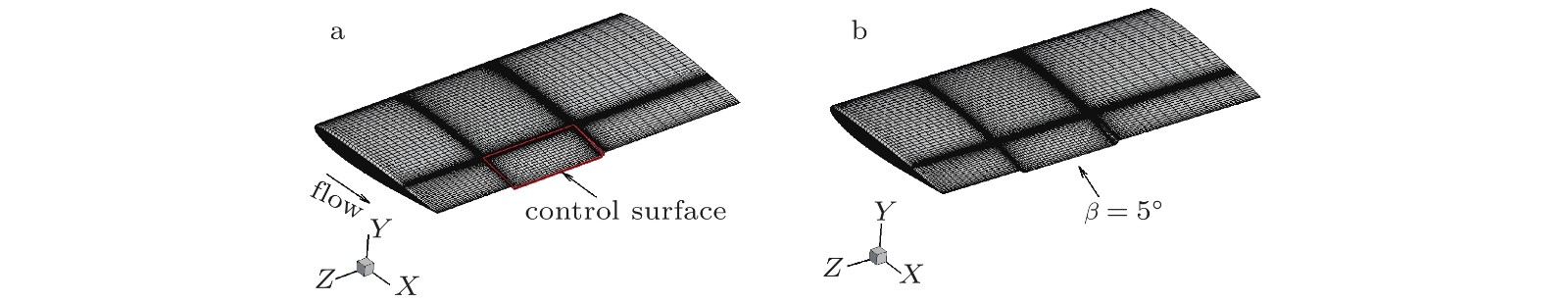
图 4 机翼控制面模态的定义. (a) BACT机翼表面气动网格, (b) 控制面偏转5度后的表面气动网格 (Huang et al. 2015a)
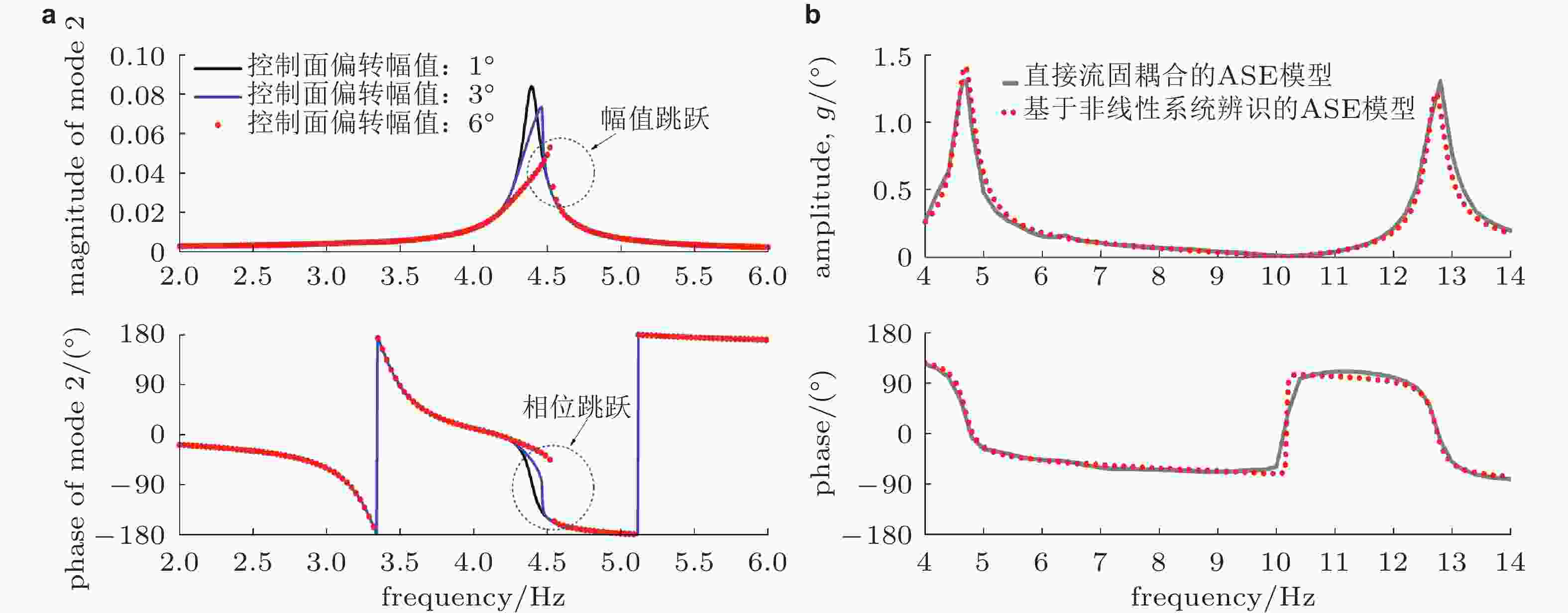
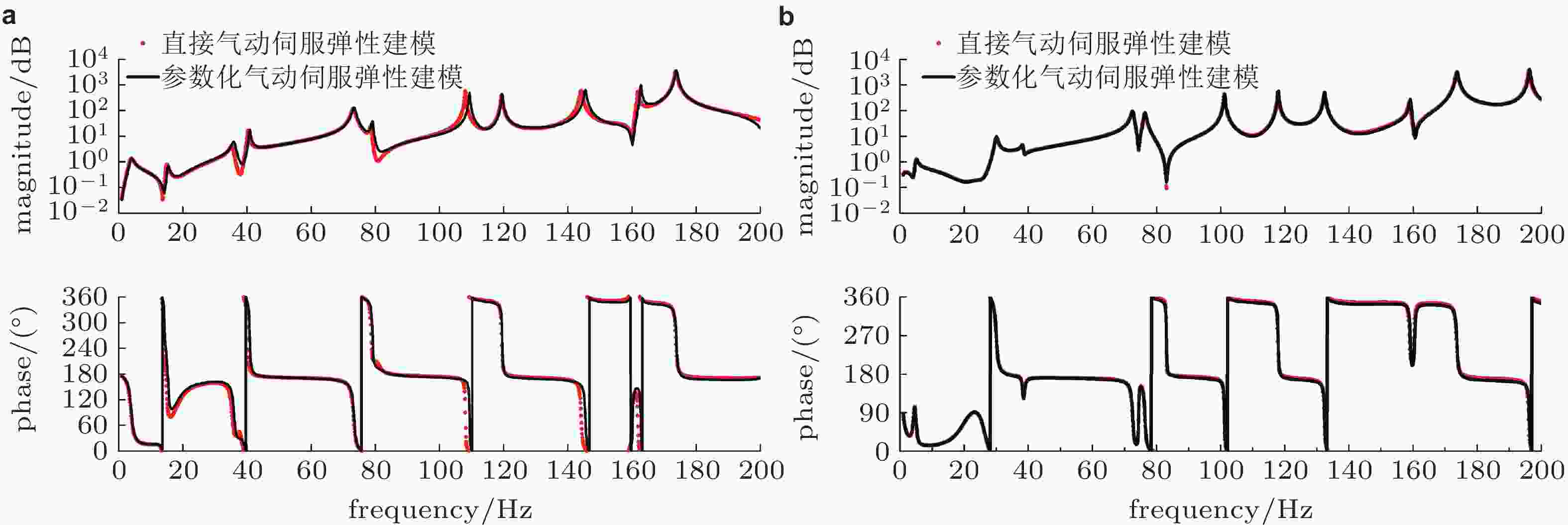
图 5 考虑非线性气动效应的ASE系统频响曲线. (a) BACT机翼第二阶模态频响曲线 (Huang et al. 2014), (b) 三维弹性机翼气动伺服弹性频响曲线 (Huang et al. 2018)
图 9 几类变体飞行器设计方案. (a) 可变展长机翼 (Yue et al. 2017), (b) 可变弯度机翼 (Chanzy & Keane 2018), (c) 可折叠式机翼 (Friswell & Inman 2006), (d) 智能变体机翼 (Weisshaar 2013)
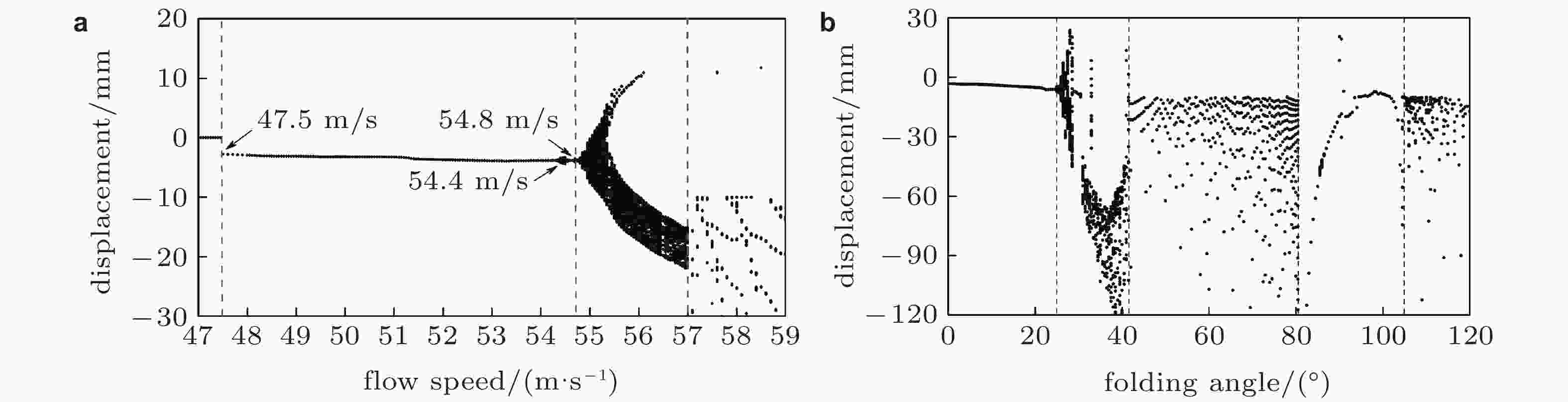
图 10 折叠翼模型气动伺服弹性频率响应随折叠角的变化规律. (a) 幅频响应曲面, (b) 相频响应曲面 (Huang et al. 2019)
图 18 飞翼布局飞行器的颤振研究. (a) 飞行器气动外形,(b) 鲁棒控制律设计框图 (Theis et al. 2016)
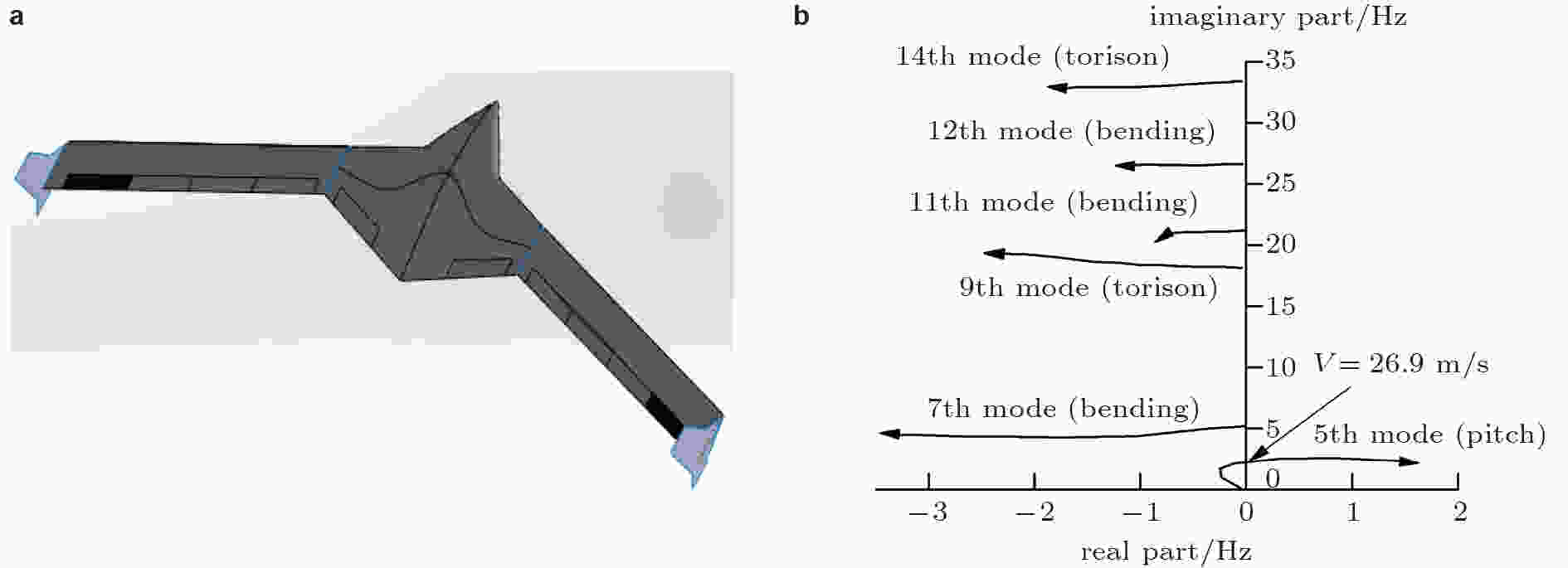
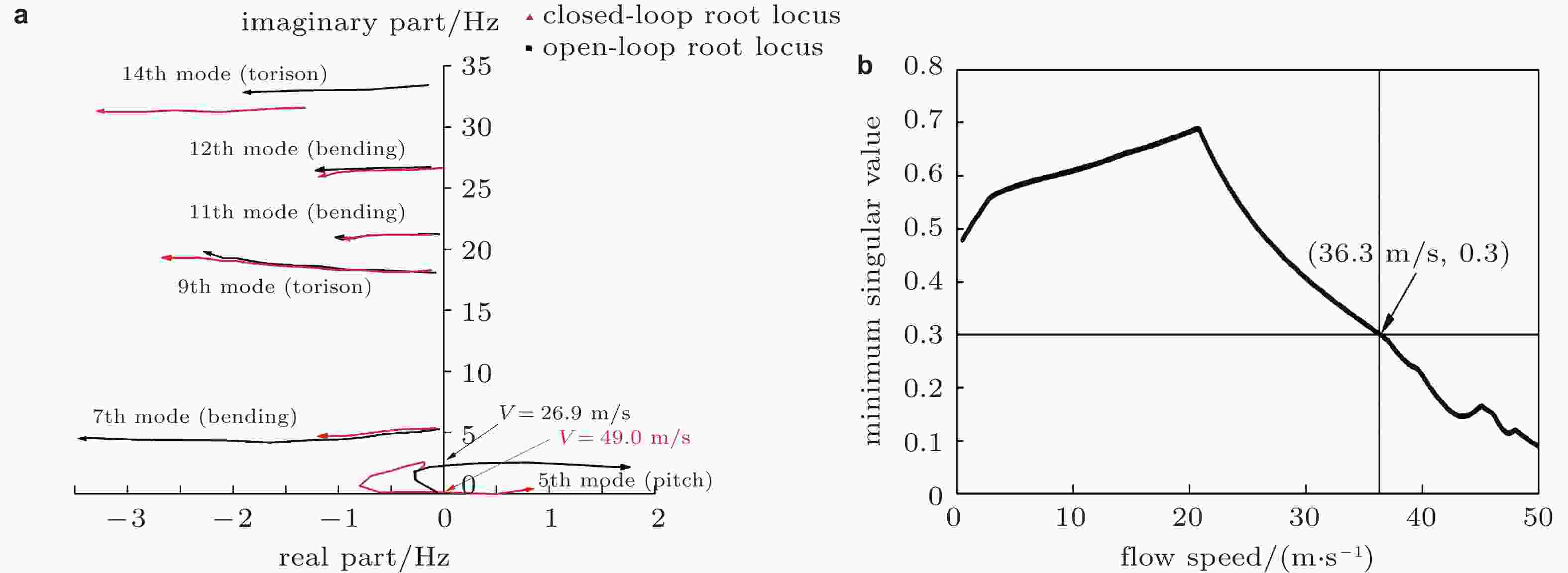
图 19 大展弦比飞翼布局飞行器的颤振研究. (a) 飞行器气动外形, (b) 开环系统颤振特性 (Zou et al. 2021)
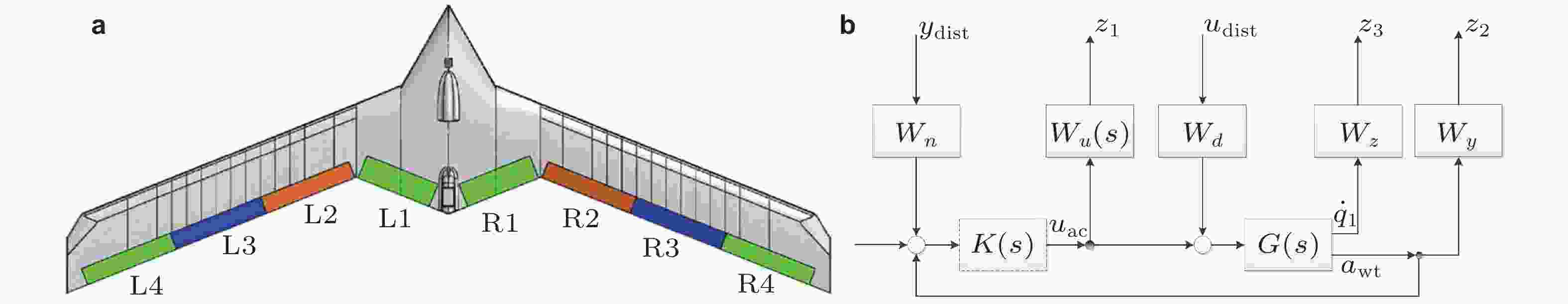
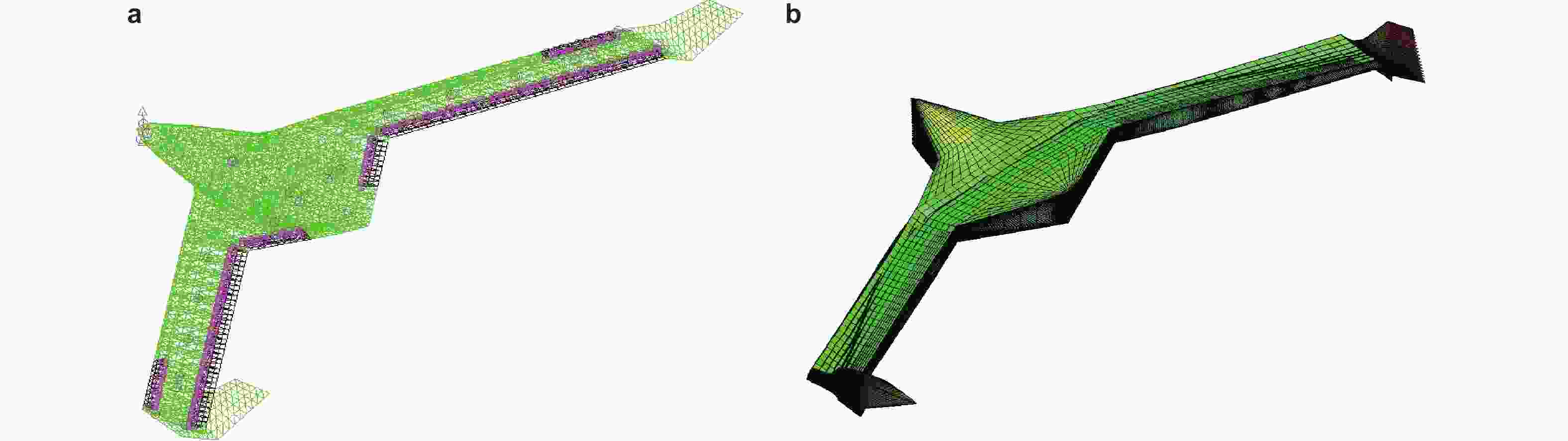
图 23 飞翼布局飞行器气动伺服弹性模型. (a) 飞翼布局飞行器有限元模型 (Schmidt 2016), (b) 飞翼布局飞行器体自由度颤振形态
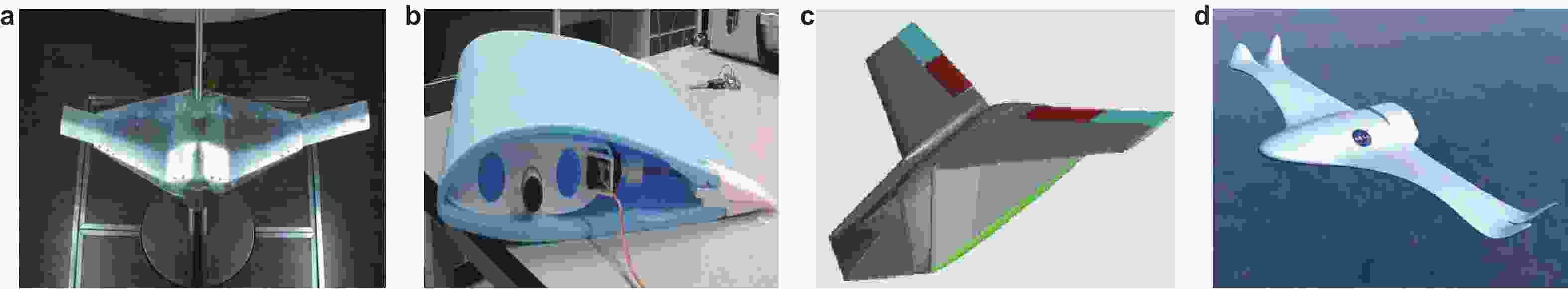
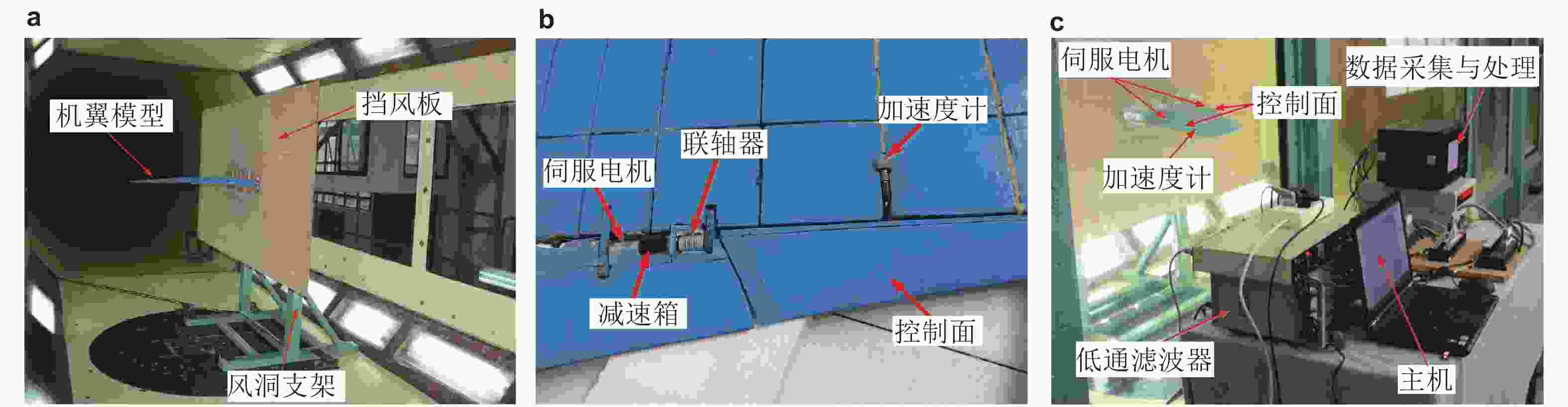
图 26 三维机翼模型颤振主动抑制风洞试验. (a) 机翼模型安装图, (b) 传感器、作动器与控制面布置, (c) 颤振主动控制系统 (Huang et al. 2015b)
图 27 三维机翼气动伺服弹性系统的理论建模与风洞实验对比. (a) 流速为20 m/s, (b) 流速为26 m/s (黄锐 2014)
图 28 气动伺服弹性实验硬件系统 (黄锐 2014)
图 30 计入时滞的颤振主动抑制. (a) 最优控制律执行框图, (b) 控制律施加前后的系统响应历程 (Huang et al. 2015b)
图 31 三维机翼体自由度颤振特性试验 (Li & Pak 2015)
图 32 3D打印大展弦比机翼气动弹性试验. (a) 半模飞翼布局无人机打印零件图, (b) 大展弦比机翼弯扭耦合颤振试验 (Pankonien et al. 2018)
图 33 飞翼布局无人机全机气动弹性飞行试验 (Danowsky et al. 2018)
表 1 预测非线性气动伺服弹性系统频率响应曲线的效率对比
BACT机翼 三维弹性机翼 非线性系统辨识的ASE模型 44.36 h 71.188 h 直接流固耦合的ASE模型 316 h 2990 h -
[1] 陈桂彬, 邹丛青, 杨超. 2004. 气动弹性设计基础. 北京: 北京航空航天大学出版社Chen G B, Zou C Q, Yang C. 2004. Aeroelastic Design Foundation. Beijing: Beihang University Press [2] 韩京清. 1998. 自抗扰控制器及其应用. 控制与决策, 13: 19-23 (Han J Q. 1998. Auto-disturbance rejection controller and it’s applications. Control and Decision, 13: 19-23). doi: 10.3321/j.issn:1001-0920.1998.01.005 [3] 韩京清. 1995a. 非线性状态误差反馈控制律–NLSEF. 控制与决策, 10: 221-226 (Han J Q. 1995a. Nonlinear state error feedback control law - NLSEF. Control and Decision, 10: 221-226). doi: 10.3321/j.issn:1001-0920.1995.03.007 [4] 韩京清. 1995b. 一类不确定对象的扩张状态观测器. 控制与决策, 10: 85-88 (Han J Q. 1995b. The “extended state observer” of a class of uncertain systems. Control and Decision, 10: 85-88). doi: 10.3321/j.issn:1001-0920.1995.01.020 [5] 韩京清, 王伟. 1994. 非线性跟踪–微分器. 系统科学与数学, 14: 177-183 (Han J Q, Wang W. 1994. Nonlinear tracking-differentiator. Journal of Systems Science and Mathematical Science, 14: 177-183). [6] 韩京清, 袁露林. 1999. 跟踪–微分器的离散形式. 系统科学与数学, 19: 268-273 (Han J Q, Yuan L L. 1999. The discrete form of tracking-differentiator. Journal of Systems Science and Mathematical Science, 19: 268-273). doi: 10.3969/j.issn.1000-0577.1999.03.003 [7] 胡海岩, 赵永辉, 黄锐. 2016. 飞机结构气动弹性分析与控制研究. 力学学报, 48: 1-27 (Hu H Y, Zhao Y H, Huang R. 2016. Studies on aeroelastic analysis and control of aircraft structures. Chinese Journal of Theoretical and Applied Mechanics, 48: 1-27). doi: 10.6052/0459-1879-15-423 [8] 黄锐. 2014. 亚/跨音速飞机结构气动弹性控制及其实验研究. [博士论文]. 南京: 南京航空航天大学Huang R. 2014. Aeroelastic control of aircraft structure in subsonic/transonic flows and its testification. [PhD Thesis]. Nanjing: Nanjing University of Aeronautics and Astronautics [9] 雷鹏轩, 余立, 陈德华, 吕彬彬. 2021. 飞行控制律对体自由度颤振特性影响试验研究. 航空学报, 42: 1-11 (Lei P X, Yu L, Chen D H, Lü B B. 2021. Experimental study on the influence of flight control law on the body freedom flutter characteristics. Acta Aeronautica et Astronautica Sinica, 42: 1-11). [10] 李杰, 齐晓慧, 万慧, 夏元清. 2017. 自抗扰控制: 研究成果总结与展望. 控制理论与应用, 34: 281-295 (Li J, Qi X H, Wan H, Xia Y Q. 2017. Active disturbance rejection control: theoretical results summary and future researches. Control Theory & Applications, 34: 281-295). doi: 10.7641/CTA.2017.60363 [11] 沐旭升, 邹奇彤, 黄锐, 胡海岩. 2020. 体自由度颤振主动抑制的多输入/多输出自抗扰控制律设计. 振动工程学报, 33: 910-920 (Mu X S, Zou Q T, Huang R, Hu H Y. 2020. Design of multiple-input/multiple-output active disturbance rejection controller for body-freedom flutter suppression. Journal of Vibration Engineering, 33: 910-920). [12] 桑为民, 陈年旭. 2009. 变体飞机的研究进展及其关键技术. 飞行力学, 27: 5-9 (Sang W M, Chen N X. 2009. Development and key technologies of the morphing aircraft. Flight Dynamics, 27: 5-9). [13] 杨超, 黄超, 吴志刚, 唐长红. 2015. 气动伺服弹性研究的进展与挑战. 航空学报, 36: 1011-1033 (Yang C, Huang C, Wu Z G, Tang C H. 2015. Progress and challenges for aeroservoelasticity research. Acta Aeronautica et Astronautica Sinica, 36: 1011-1033). [14] 杨超, 宋晨, 吴志刚, 张瞿辉. 2010. 多控制面飞机的全机颤振主动抑制设计. 航空学报, 31: 1501-1508 (Yang C, Song C, Wu Z G, Zhang Z H. 2010. Active flutter suppression of airplane configuration with multiple control surfaces. Acta Aeronautica et Astronautica Sinica, 31: 1501-1508). [15] 于明礼, 文浩, 胡海岩. 2006. 二维翼段颤振的H∞控制. 振动工程学报, 19: 326-330 (Yu M L, Wen H, Hu H Y. 2006. Active flutter suppression of a two dimensional airfoil using H∞ synthesis. Journal of Vibration Engineering, 19: 326-330). doi: 10.3969/j.issn.1004-4523.2006.03.007 [16] 于明礼, 文浩, 胡海岩, 赵永辉. 2007. 二维翼段颤振的μ控制. 航空学报, 28: 340-343 (Yu M L, Wen H, Hu H Y, Zhao Y H. 2007. Active flutter suppression of a two dimensional airfoil section using μ synthesis. Acta Aeronautica et Astronautica Sinica, 28: 340-343). doi: 10.3321/j.issn:1000-6893.2007.02.017 [17] 赵永辉, 黄锐. 2015. 高等气动弹性力学与控制. 北京: 科学出版社Zhao Y H, Huang R. 2015. Advanced Aeroelasticity and Control. Beijing: Science Press [18] Albano E, Rodden W P. 1969. A doublet-lattice method for calculating lift distributions on oscillating surfaces in subsonic flows. AIAA J., 7: 279-285. doi: 10.2514/3.5086 [19] Bagheri S. 2013. Koopman-mode decomposition of the cylinder wake. J. Fluid Mech., 726: 596-623. doi: 10.1017/jfm.2013.249 [20] Barbarino S, Bilgen O, Ajaj R M, Friswell M I, Inman D J. 2011. A review of morphing aircraft. J. Intell. Mater. Syst. Struct., 22: 823-877. doi: 10.1177/1045389X11414084 [21] Berkooz G, Holmes P, Lumley J. 1993. The proper orthogonal decomposition in the analysis of turbulent flows. Annu. Rev. Fluid Mech., 25: 539-575. doi: 10.1146/annurev.fl.25.010193.002543 [22] Brock B J, Griffin J A. 1975. The supersonic doublet-lattice method – A comparison of two approaches// 16th Structural Dynamics, and Materials Conference, Denver, CO, USA. [23] Brunton S L, Proctor J L, Kutz J N. 2016. Discovering governing equations from data by sparse identification of nonlinear dynamical systems. Proc. Natl. Acad. Sci. U.S.A., 113: 3932-3937. doi: 10.1073/pnas.1517384113 [24] Chanzy Q, Keane A J. 2018. Analysis and experimental validation of morphing UAV wings. Aeronaut J., 122: 390-407. doi: 10.1017/aer.2017.130 [25] Danowsky B P, Kotikalpudi A, Schmidt D K, Regan C, Seiler P. 2018. Flight testing flutter suppression on a small flexible flying-wing aircraft// 2018 Multidisciplinary Analysis and Optimization Conference, American Institute of Aeronautics and Astronautics Inc, AIAA. [26] Dowell E H. 2015. A Modern Course in Aeroelasticity, The Fifth Revised and Enlarged Edition. Springer, 1-649. [27] Felt L R, Huttsell L J, Noll T E, Cooley D E. 1979. Aeroservoelastic Encounters. J Aircr, 16: 477-483. doi: 10.2514/3.58551 [28] Friswell M I, Inman D J. 2006. Morphing concepts for UAVs// 21st Bristol UAV Concepts Conference. [29] Gao Z Q. 2003. Scaling and bandwidth-parameterization based controller tuning // The American control conference, IEEE, USA. [30] Glaz B, Friedmann P P, Liu L, Cajigas J G, Bain J, Sankar L N. 2010. Reduced-order nonlinear unsteady aerodynamic modeling using a surrogate-based recurrence framework. AIAA J., 48: 2418-2429. doi: 10.2514/1.J050471 [31] Hall K C, Thomas J P, Dowell E H. 2000. Proper orthogonal decomposition technique for transonic unsteady aerodynamic flows. AIAA J., 38: 1853-1862. doi: 10.2514/2.867 [32] Hall K C, Thomas J P, Clark W S. 2002. Computation of unsteady nonlinear flows in cascades using a harmonic balance technique. AIAA J., 40: 879-886. doi: 10.2514/2.1754 [33] Hu H Y, Wang Z H. 2002. Dynamics of Controlled Mechanical Systems with Delayed Feedback. Berlin: Springer-Verlag. [34] Hu W, Yang Z C, Gu Y S. 2016. Aeroelastic study for folding wing during the morphing process. J. Sound Vib., 365: 216-229. doi: 10.1016/j.jsv.2015.11.043 [35] Huang R, Hu H Y, Zhao Y H. 2014. Nonlinear reduced-order modeling for multiple-input/multiple-output aerodynamic systems. AIAA J., 52: 1219-1231. doi: 10.2514/1.J052323 [36] Huang R, Hu H Y, Zhao Y H. 2012. Designing active flutter suppression for high-dimensional aeroelastic systems involving a control delay. J Fluids Struct, 34: 33-50. doi: 10.1016/j.jfluidstructs.2012.05.012 [37] Huang R, Hu H Y, Zhao Y H. 2013. Nonlinear aeroservoelastic analysis of a controlled multiple-actuated-wing model with free-play. J Fluids Struct, 42: 245-269. doi: 10.1016/j.jfluidstructs.2013.06.007 [38] Huang R, Li H K, Hu H Y, Zhao Y H. 2015a. Open/closed-loop aeroservoelastic predictions via nonlinear, reduced-order aerodynamic models. AIAA J., 53: 1812-1824. doi: 10.2514/1.J053424 [39] Huang R, Liu H J, Yang Z J, Zhao Y H, Hu H Y. 2018. Nonlinear reduced-order models for transonic aeroelastic and aeroservoelastic problems. AIAA J., 56: 3718-3731. doi: 10.2514/1.J056760 [40] Huang R, Qian W M, Hu H Y, Zhao Y H. 2015b. Design of active flutter suppression and wind-tunnel tests of a wing model involving a control delay. J Fluids Struct, 55: 409-427. doi: 10.1016/j.jfluidstructs.2015.03.014 [41] Huang R, Yang Z J, Yao X J, Zhao Y H, Hu H Y. 2019. Parameterized modeling methodology for efficient aeroservoelastic analysis of a morphing wing. AIAA J., 57: 5543-5552. doi: 10.2514/1.J058211 [42] Huang R, Zhao Y H, Hu H Y. 2016. Wind-tunnel tests for active flutter control and closed-loop flutter identification. AIAA J., 54: 2089-2099. doi: 10.2514/1.J054649 [43] Huang R, Zhou X H. 2021. Parameterized fictitious mode of a morphing wing with bilinear hinge stiffness. AIAA J, 00: 1-16. [44] Hwangbo J, Sa I, Siegwart R, Hutter M. 2017. Control of a quadrotor with reinforcement learning. IEEE Robot. Autom. Lett., 2: 2096-2103. doi: 10.1109/LRA.2017.2720851 [45] Ivanco T G, Scott R C, Love M H, Zink S, Weisshaar T A. 2007. Validation of the Lockheed Martin morphing concept with wind tunnel testing// 48th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Honolulu, Hawaii, AIAA 2007-2235. [46] Kashki M, Abdel-Magid Y L, Abido M A. 2008. A reinforcement learning automata optimization approach for optimum tuning of PID controller in AVR system. // Lecture Notes in Computer Science, Springer, Berlin, Heidelberg, 684-692. [47] Keidel D, Molinari G, Ermanni P. 2019. Aero-structural optimization and analysis of a camber-morphing flying wing: Structural and wind tunnel testing. J Intell Mater Syst Struct, 30: 908-923. doi: 10.1177/1045389X19828501 [48] Koch W, Mancuso R, West R, Bestavros A. 2019. Reinforcement learning for UAV attitude control. ACM Trans. Cyber-Phys. Syst. 3(2): article 22. [49] Kou J Q, Zhang W W. 2019. Dynamic mode decomposition with exogenous input for data-driven modeling of unsteady flows. Phys. Fluids, 31: 057106. doi: 10.1063/1.5093507 [50] Li D, Zhao S, Ronch A Da, Xiang J, Drofelnik J, Li Y, Zhang L, Wu Y, Kintscher M, Monner H P, et al. 2018. A review of modelling and analysis of morphing wings. Prog. Aerosp. Sci., 100: 46-62. doi: 10.1016/j.paerosci.2018.06.002 [51] Li W W, Pak C G. 2015. Mass balancing optimization study to reduce flutter speeds of the X-56A aircraft. J Aircr., 52: 1359-1365. doi: 10.2514/1.C033044 [52] Lillicrap T P, Hunt J J., Pritzel A, Heess N, Erez T, Tassa Y, Silver D, Wierstra D. 2016. Continuous control with deep reinforcement learning// 4th International Conference on Learning Representations, ICLR 2016 - Conference Track Proceedings. [53] Liu H, Hu H Y, Zhao Y H, Huang R. 2014. Efficient reduced-order modeling of unsteady aerodynamics robust to flight parameter variations. J Fluids Struct, 49: 728-741. doi: 10.1016/j.jfluidstructs.2014.06.015 [54] Livne E. 2018. Aircraft active flutter suppression: State of the art and technology maturation needs. J Aircr., 55: 410-452. doi: 10.2514/1.C034442 [55] Luo K, Hu H Y, Liu C, et al. 2017. Model order reduction for dynamic simulation of flexible multibody system via absolute nodal coordinate formulation. Comp. Meth. Appl. Mech. Engng, 324: 573-594. doi: 10.1016/j.cma.2017.06.029 [56] Moradi M, Sadeghi M H, Dowell E H. 2018. Experimental and theoretical flutter investigation for a range of wing wind-tunnel models. J Aircr, 55: 891-897. doi: 10.2514/1.C034311 [57] Moulin B, Karpel M. 2007. Gust loads alleviation using special control surfaces. J Aircr., 44: 17-25. doi: 10.2514/1.19876 [58] Mukhopadhyay V. 1995. Flutter suppression control law design and testing for the active flexible wing. J Aircr, 32: 45-51. doi: 10.2514/3.46682 [59] Mukhopadhyay V. 2000. Transonic flutter suppression control law design and wind-tunnel test results. J. Guid. Control. Dyn., 23: 930-937. doi: 10.2514/2.4635 [60] Noack B R, Afanasiev K, Morzynski M, Tadmor G, Thiele F. 2003. A hierarchy of low-dimensional models for the transient and post-transient cylinder wake. J. Fluid Mech., 497: 335-363. doi: 10.1017/S0022112003006694 [61] Noack B R, Papas P, Monkewitz P A. 2005. The need for a pressure-term representation in empirical Galerkin models of incompressible shear flows. J. Fluid Mech., 523: 339-365. doi: 10.1017/S0022112004002149 [62] Noeel J P, Esfahani A F, Kerschen G, Schoukens J. 2017. A nonlinear state-space approach to hysteresis identification. Mech Syst Signal Process, 84: 171-184. doi: 10.1016/j.ymssp.2016.08.025 [63] Opgenoord M M J, Drela M, Willcox K E. 2018. Physics-based low-order model for transonic flutter prediction. AIAA J., 56: 1519-1531. doi: 10.2514/1.J056710 [64] Pankonien A M, Reich G W. 2018. Multi-Material printed wind-tunnel flutter model. AIAA J., 56: 793-807. doi: 10.2514/1.J056097 [65] Pendleton E W, Bessette D, Field P B, Miller G D, Griffin K E. 2000. Active aeroelastic wing flight research program: technical program and model analytical development. J Aircr, 37: 554-561. doi: 10.2514/2.2654 [66] Proctor J L, Brunton S L, Kutz J N. 2016. Dynamic mode decomposition with control. SIAM J. Appl. Dyn. Syst., 15: 142-161. doi: 10.1137/15M1013857 [67] Proctor J L, Brunton S L, Kutz J N. 2018. Generalizing Koopman theory to allow for inputs and control. SIAM J. Appl. Dyn. Syst., 17: 909-930. doi: 10.1137/16M1062296 [68] Rowley C W, Colonius T, Murray R M. 2004. Model reduction for compressible flows using POD and Galerkin projection. Physica D, 189: 115-129. doi: 10.1016/j.physd.2003.03.001 [69] Rowley C W, Mezic I, Bagheri S, Schlatter P, Henningson D S. 2009. Spectral analysis of nonlinear flows. J. Fluid Mech., 641: 115-127. doi: 10.1017/S0022112009992059 [70] Schmidt D K. 2016. Stability augmentation and active flutter suppression of a flexible flying-wing drone. J. Guid. Control. Dyn., 39: 409-422. doi: 10.2514/1.G001484 [71] Schmid P J. 2010. Dynamic mode decomposition of numerical and experimental data. J. Fluid Mech., 656: 5-28. doi: 10.1017/S0022112010001217 [72] Schmidt D K, Danowsky B P, Kotikalpudi A, Theis J, Regan C D, Seiler P J, Kapania R K. 2020. Modeling, design, and flight testing of three flutter controllers for a flying-wing drone. J Aircr, 57: 615-634. doi: 10.2514/1.C035720 [73] Seena A, Sung H J. 2011. Dynamic mode decomposition of turbulent cavity flows for self-sustained oscillations. Int J Heat Fluid Flow, 32: 1098-1110. doi: 10.1016/j.ijheatfluidflow.2011.09.008 [74] Silver D, Lever G, Heess N, Degris T, Wierstra D, Riedmiller M. 2014. Deterministic policy gradient algorithms // The 31st International Conference on Machine Learning, PMLR, Bejing, China, 387–395. [75] Simiriotis N, Fragiadakis M, Rouchon J F, Braza M. 2021. Shape control and design of aeronautical configurations using shape memory alloy actuators. Comput. Struct., 244: 106434. doi: 10.1016/j.compstruc.2020.106434 [76] Snyder M P, Sanders B, Eastep F E, Frank G J. 2009. Vibration and flutter characteristics of a folding wing. J Aircr, 46: 791-799. doi: 10.2514/1.34685 [77] Taira K, Brunton S L, Dawson S T M, Rowley C W, Colonius T, McKeon B J, Schmidt O T, Gordeyev S, Theofilis V, Ukeiley L S. 2017. Modal analysis of fluid flows: An overview. AIAA J., 55: 4013-4041. doi: 10.2514/1.J056060 [78] Taira K, Hemati M S, Brunton S L, Sun Y Y, Duraisamy K, Bagheri S, Dawson S T M, Yeh C A. 2020. Modal analysis of fluid flows: applications and outlook. AIAA J., 58: 998-1022. doi: 10.2514/1.J058462 [79] Tang D, Dowell E H. 2008. Theoretical and experimental aeroelastic study for folding wing structures. J Aircr, 45: 1136-1147. doi: 10.2514/1.32754 [80] Tang Y X, Hu H Y, Tian Q. 2019. Model order reduction based on successively local linearizations for flexible multibody dynamics, Int. J. Nume. Meth. Engng, 118: 159-180. [81] Theis J, Pfifer H, Seiler P. 2016. Robust control design for active flutter suppression// AIAA Atmospheric Flight Mechanics Conference, American Institute of Aeronautics and Astronautics Inc, AIAA. [82] Theodorsen T. 1935. General theory of aerodynamic instability and the mechanism of flutter. Tech.Rep.496, NACA. [83] Wang X, Zhou W, Zhang Z, Jiang J, Wu Z. 2021. Theoretical and experimental investigations on modified LQ terminal control scheme of piezo-actuated compliant structures in finite time. J. Sound Vib., 491: 115762. doi: 10.1016/j.jsv.2020.115762 [84] Wang Y, Wynn A, Palacios R. 2016. Nonlinear modal aeroservoelastic analysis framework for flexible aircraft. AIAA J., 54: 3075-3090. doi: 10.2514/1.J054537 [85] Waszak M R. 2001. Robust multivariable flutter suppression for Benchmark Active Control Technology wind-tunnel model. J. Guid. Control. Dyn., 24: 147-153. doi: 10.2514/2.4694 [86] Waszak M R, Srinathkumar S. 1995. Flutter suppression for the active flexible wing: a classical design. J Aircr, 32: 61-67. doi: 10.2514/3.46684 [87] Watkins C J C H, Dayan P. 1992. Q-Learning. Mach. Learn, 8: 279-292. [88] Weisshaar T A. 2013. Morphing aircraft systems: historical perspectives and future challenges. J Aircr, 50: 337-353. doi: 10.2514/1.C031456 [89] Willcox K, Peraire J. 2002. Balanced model reduction via the proper orthogonal decomposition. AIAA J., 40: 2323-2330. doi: 10.2514/2.1570 [90] Williams M O., Kevrekidis I G., Rowley C W. 2015. A data-driven approximation of the Koopman operator: extending dynamic mode decomposition. J Nonlinear Sci, 25: 1307-1346. doi: 10.1007/s00332-015-9258-5 [91] Winter M, Breitsamter C. 2016. Neurofuzzy-model-based unsteady aerodynamic computations across varying freestream conditions. AIAA J., 54: 2705-2720. doi: 10.2514/1.J054892 [92] Xie D, Xu M, Dowell E H. 2013. Projection-free proper orthogonal decomposition method for a cantilever plate in supersonic flow. J. Sound Vib., 333: 6190-6208. [93] Yang Z J, Huang R, Liu H J, Zhao Y H, Hu H Y. 2020. An improved nonlinear reduced-order modeling for transonic aeroelastic systems. J Fluids Struct, 90: 102926. [94] Yang Z J, Huang R, Zhao Y H, Hu H Y. 2017. Design of an active disturbance rejection control for transonic flutter suppression. J. Guid. Control. Dyn, 40: 2905-2916. doi: 10.2514/1.G002690 [95] Yang Z J, Huang R, Zhao Y H, Hu H Y. 2019. Transonic flutter suppression for a three-dimensional elastic wing via active disturbance rejection control. J. Sound Vib., 445: 168-187. doi: 10.1016/j.jsv.2019.01.006 [96] Yao W G, Marques S. 2017. Nonlinear aerodynamic and aeroelastic model reduction using a discrete empirical interpolation method. AIAA J., 55: 624-637. doi: 10.2514/1.J055143 [97] Yao W G, Marques S. 2015. Prediction of transonic limit-cycle oscillations using an aeroelastic harmonic balance method. AIAA J., 53: 2040-2051. doi: 10.2514/1.J053565 [98] Yue T, Zhang X Y, Wang L X, Ai J Q. 2017. Flight dynamic modeling and control for a telescopic wing morphing aircraft via asymmetric wing morphing. Aerosp Sci Technol, 70: 328-338. doi: 10.1016/j.ast.2017.08.013 [99] Zeng J, Kukreja S L, Moulin B. 2012. Experimental model-based aeroelastic control for flutter suppression and gust-load alleviation. J. Guid. Control. Dyn., 35: 1377-1390. doi: 10.2514/1.56790 [100] Zhang W W, Wang B, Ye Z, Quan J. 2012. Efficient method for limit cycle flutter analysis by nonlinear aerodynamic reduced-order models. AIAA J., 50: 1019-1028. doi: 10.2514/1.J050581 [101] Zhao, Y H. 2009. Stability of a two-dimensional airfoil with time-delayed feedback control. J Fluids Struct, 25: 1-25. doi: 10.1016/j.jfluidstructs.2008.03.003 [102] Zhao, Y H. 2011. Stability of a time-delayed aeroelastic system with a control surface. Aerosp Sci Technol, 15: 72-77. doi: 10.1016/j.ast.2010.05.008 [103] Zhao Y H, Hu H Y. 2012. Parameterized aeroelastic modeling and flutter analysis for a folding wing. J. Sound Vib., 331: 308-324. doi: 10.1016/j.jsv.2011.08.028 [104] Zhao Y H, Yue C Y, Hu H Y. 2016. Gust load alleviation on a large transport airplane. J Aircr, 53: 1-15. [105] Zou Q T, Mu X S, Li H K, Huang R, Hu H Y. 2021. Robust active suppression for body-freedom flutter of a flying-wing unmanned aerial vehicle. J Franklin I., 358: 2642-2660. doi: 10.1016/j.jfranklin.2021.01.012 -




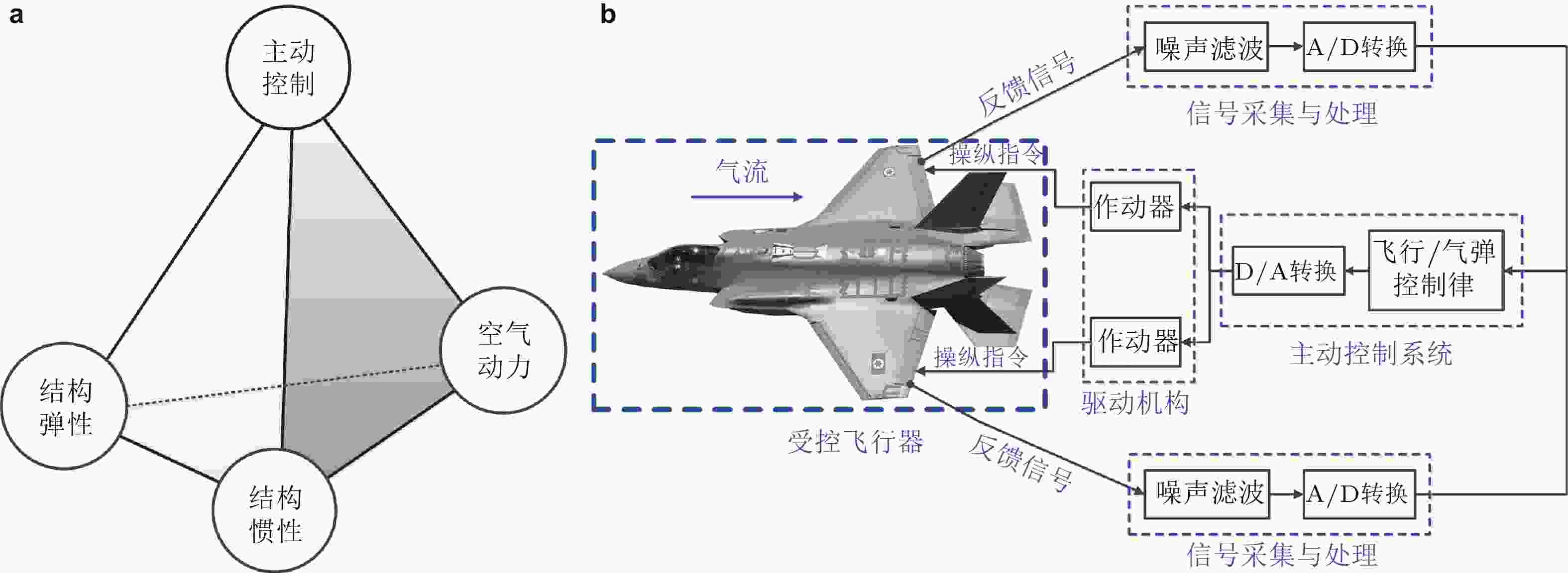
 下载:
下载: