-
摘要: 在高超声速飞行技术领域, 特别是涉及到高焓气体流动的研究, 高超声速风洞试验仍然是目前最可靠的研究手段. 风洞流场的品质是高超声速风洞研发最重要的一项性能指标, 其取决于喷管设计采用的理论与方法, 也是风洞设计最关注的一项核心技术. 针对二维轴对称型面喷管设计, 本文首先综述了传统高超声速喷管设计的主要理论和常用方法, 它们在高超声速喷管设计中曾经发挥了重要作用, 包括理论方法, 近似方法和基于两者的修正方法. 然后, 考虑高温气体效应, 分析了高焓喷管设计时面临的困难与问题, 从流动介质物性变化、高温边界层发展和非平衡过程效应三方面, 综述了国内外在高超声速高焓喷管设计方面的研究进展. 最后, 对于高焓喷管的设计理论和方法的发展作了展望, 期望对于推动我国高超声速高焓喷管设计技术的发展提供一些有意义的启示.Abstract: In the field of hypersonic flight technology, especially the study of high-enthalpy flows, the hypersonic wind tunnel test, at present, is still the most reliable and convenient research method. For the development of hypersonic wind tunnels, one of the most important performance indicators is the uniform flow quality, which depends on the theories and methods of hypersonic nozzle design, and is the core technique of wind tunnel design. For the design of a two-dimensional axisymmetric contour nozzle, this paper first reviews the main theories and traditional design methods that have played an important role in the design of hypersonic nozzles, including the theoretical methods, the approximate methods, and the correction methods for both. Then, due to the high-temperature gas effects, the difficulties and problems faced in the design of high-enthalpy nozzles are analyzed in detail. In terms of the changes in the physical properties of the test gases, the development of high-temperature boundary-layer, and the effects of non-equilibrium processes, this paper also reviews the domestic and foreign research progresses in the design of hypersonic and high-enthalpy nozzles. Finally, the development of theory and method for designing the high-enthalpy nozzle is prospected, and we expect it to promote the development of hypersonic nozzle design technology in China.
-
Key words:
- hypersonic /
- high-enthalpy gas /
- shock tunnel /
- nozzle design /
- real-gas effects
-
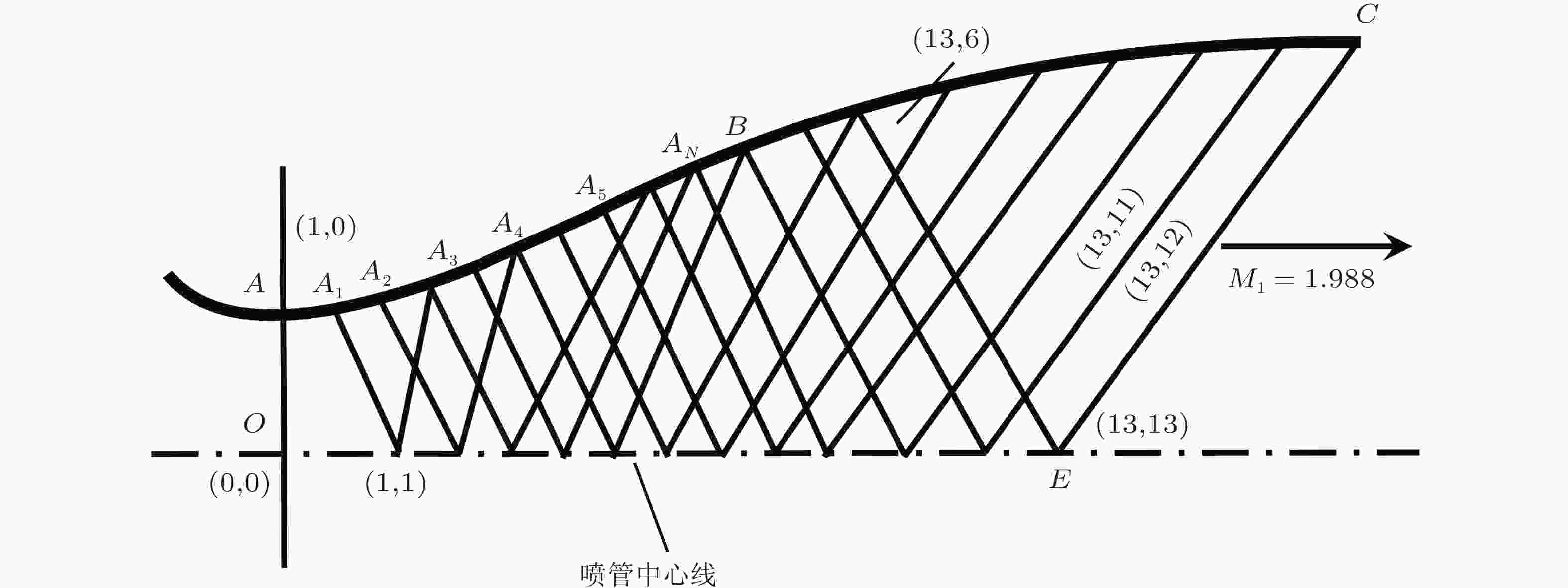
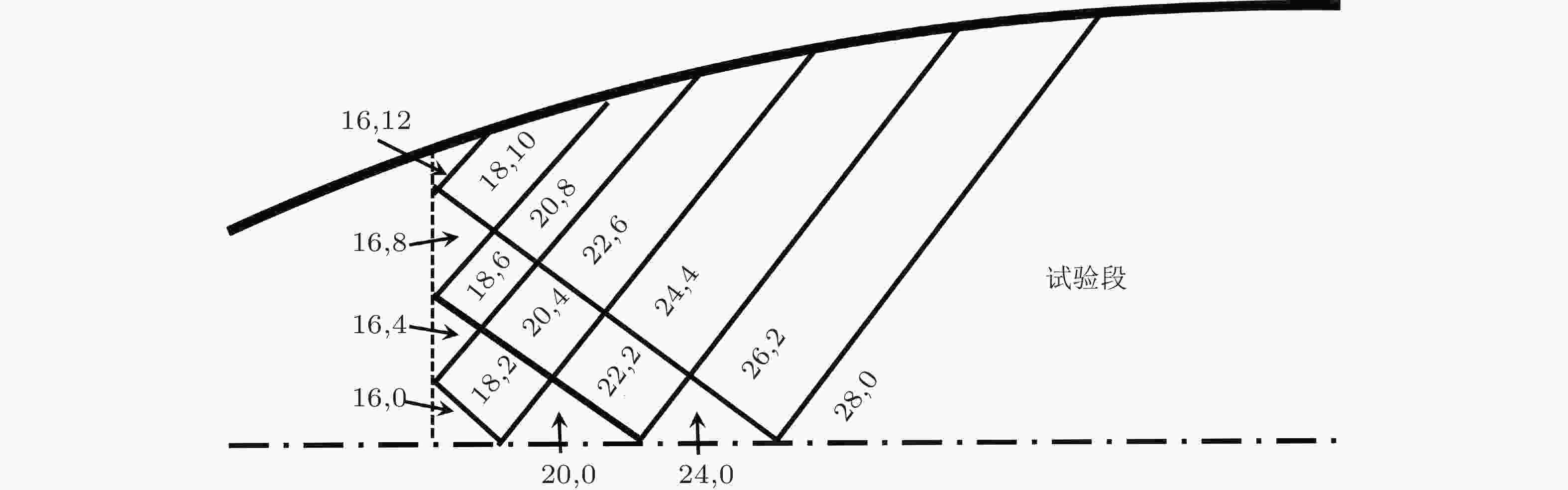
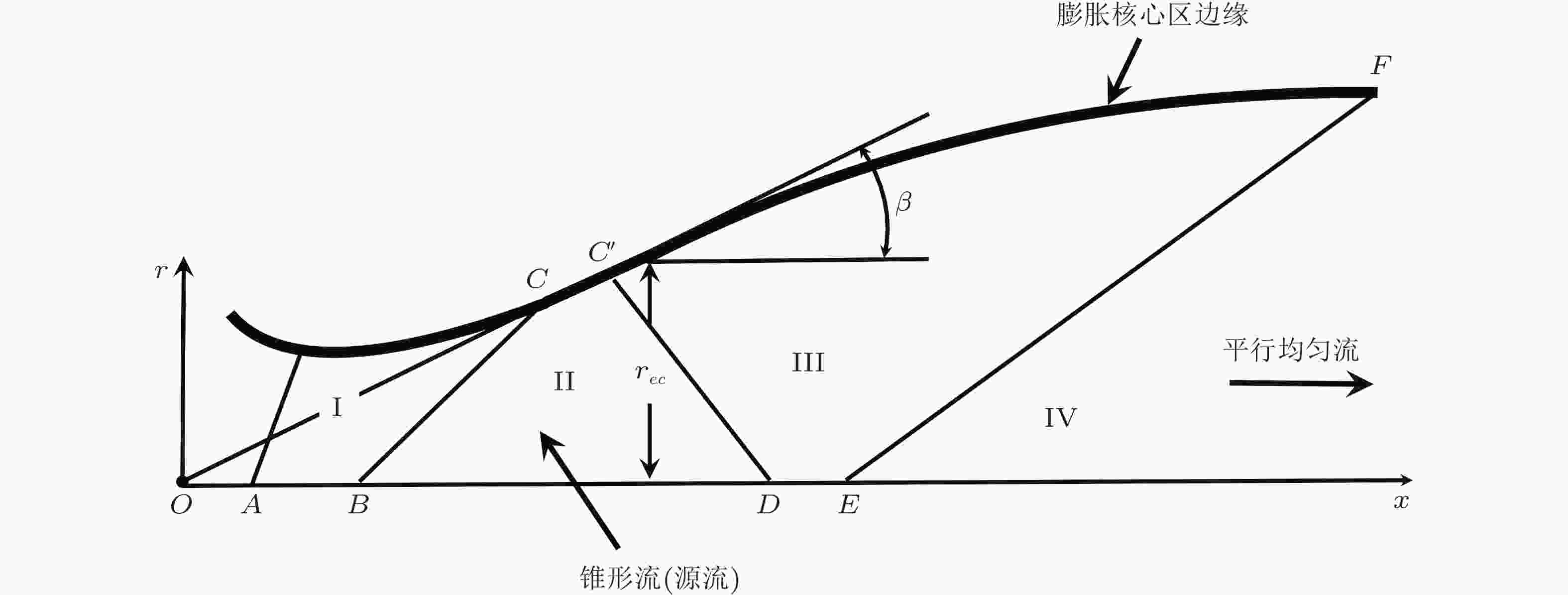
图 3 喷管设计特征线网格(伍荣林等1985)
图 4 喷管设计Puckett法(Puckett 1946)
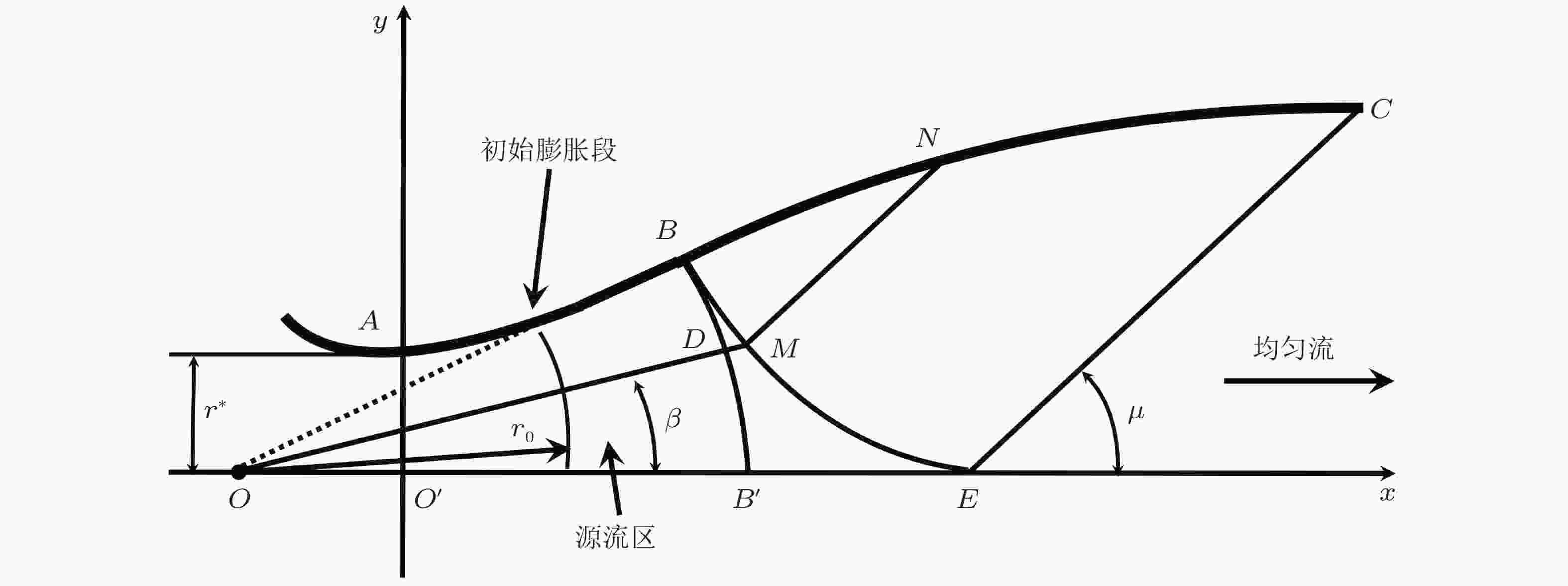
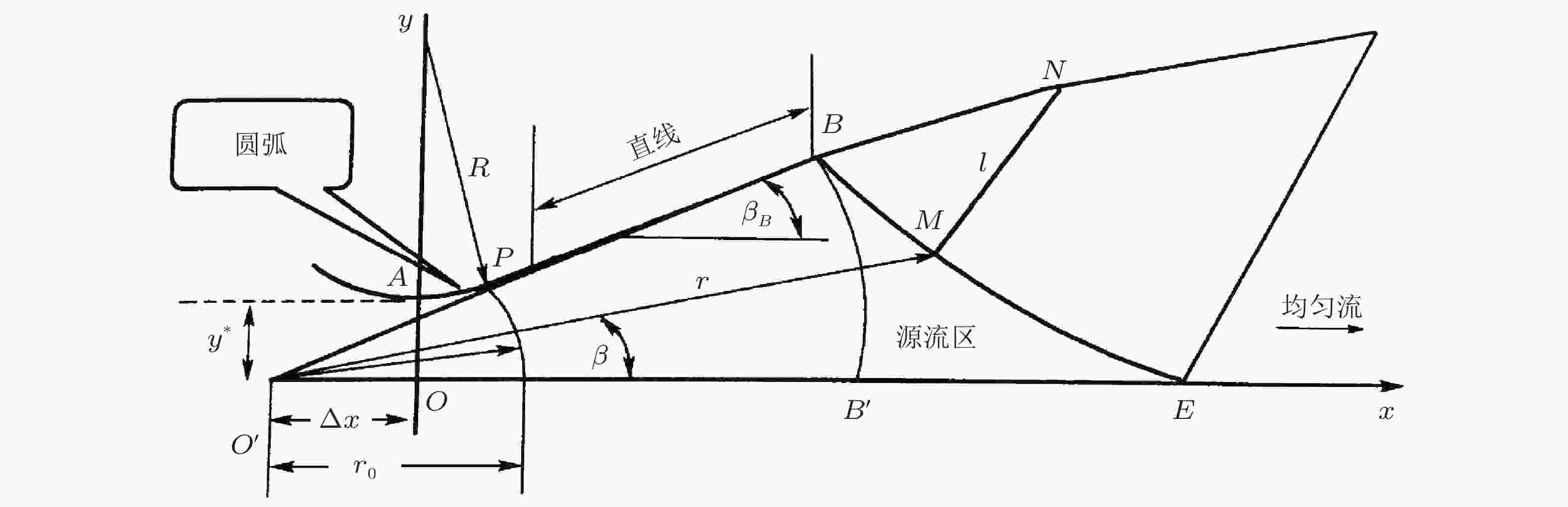
图 5 喷管设计Foelsch法 (Foelsch 1946)
图 7 喷管设计Cresci法 (Cresci 1958)
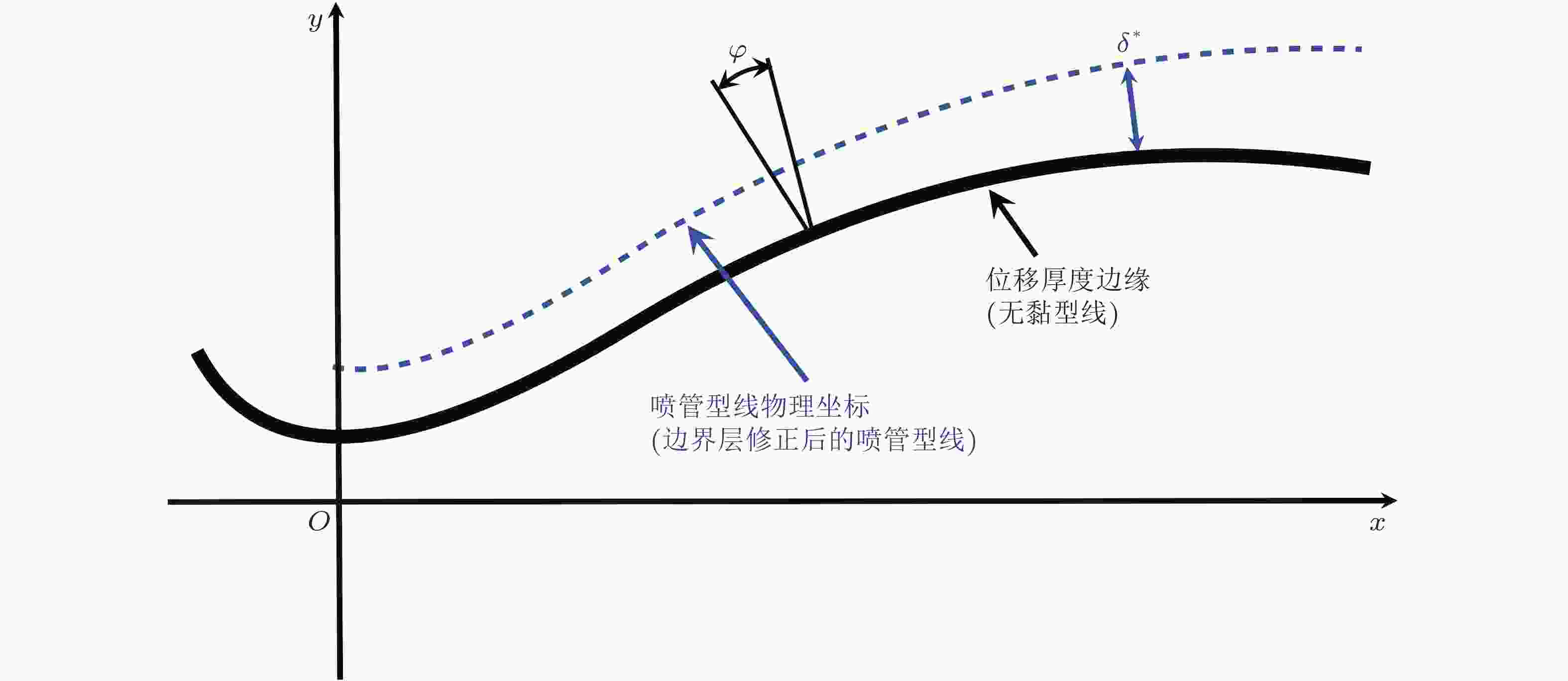
图 9 喷管无黏位流型线设计示意图 (Potter & Carden 1968)
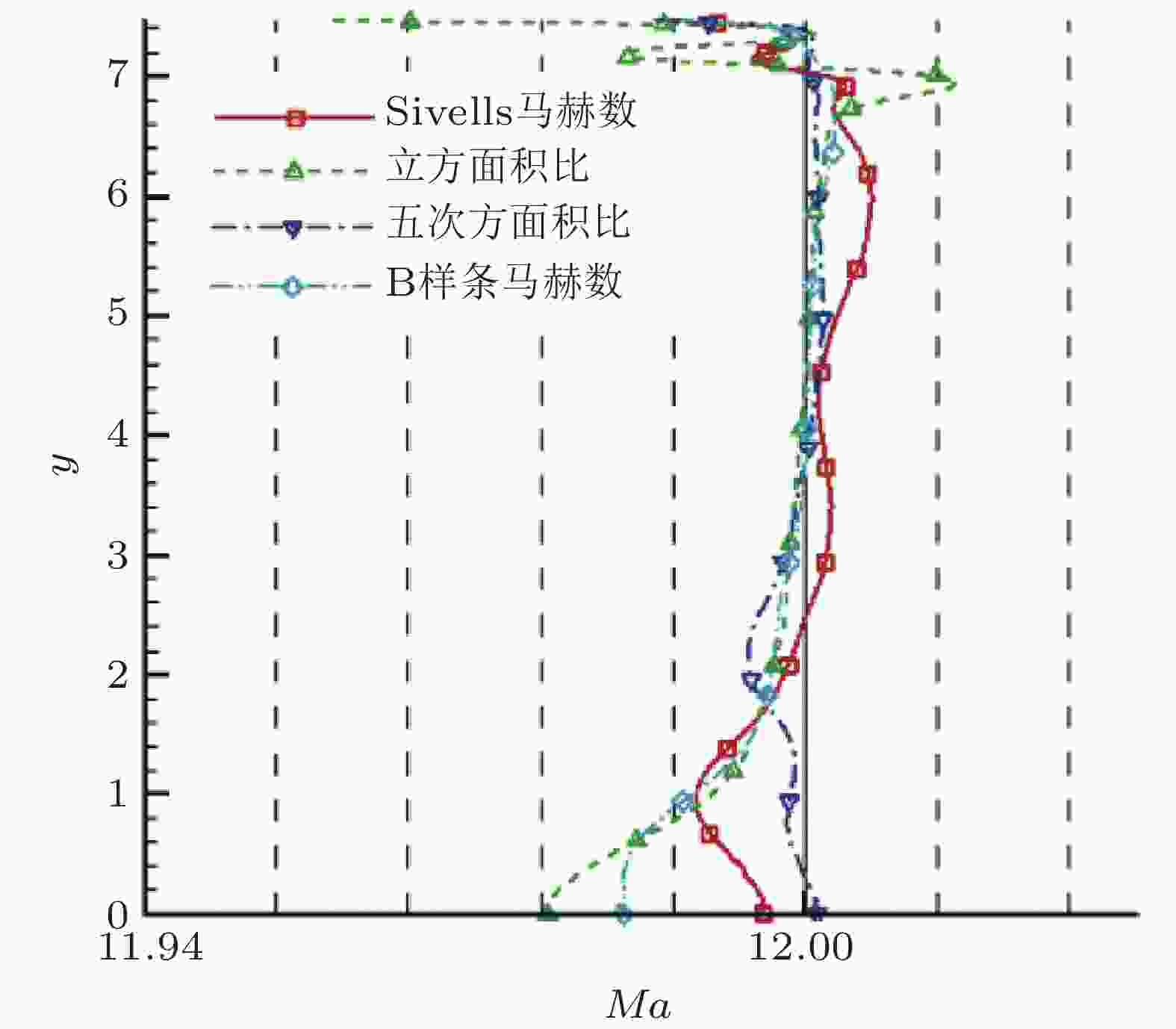
图 10 不同方法喷管黏型型面流场马赫数等值线分布 (张敏莉等2007). (a) Sivells方法喷管, (b) 新的短化喷管
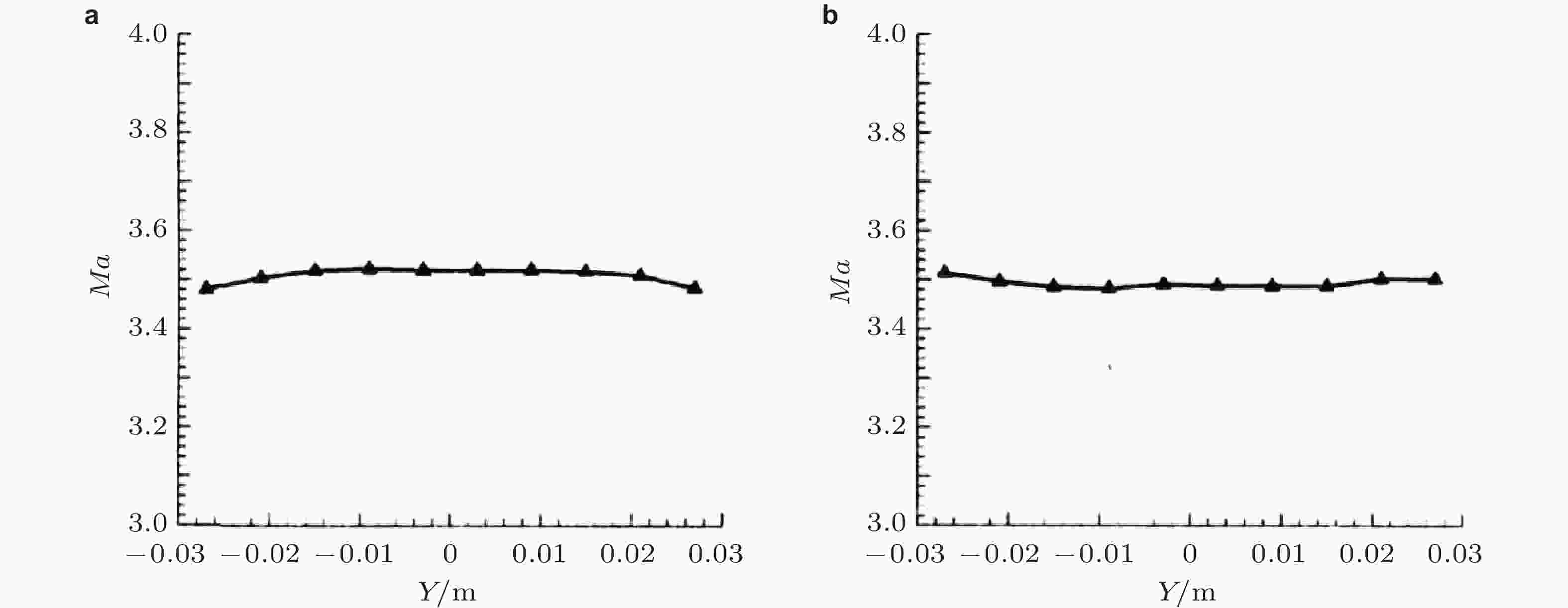
图 11 不同方法喷管流场校测喷管出口马赫数分布 (张敏莉等2007). (a) Sivells方法喷管, (b) 新的短化喷管
图 12 不同轴线马赫数预设方法的喷管出口马赫数 (胡振震等2016)
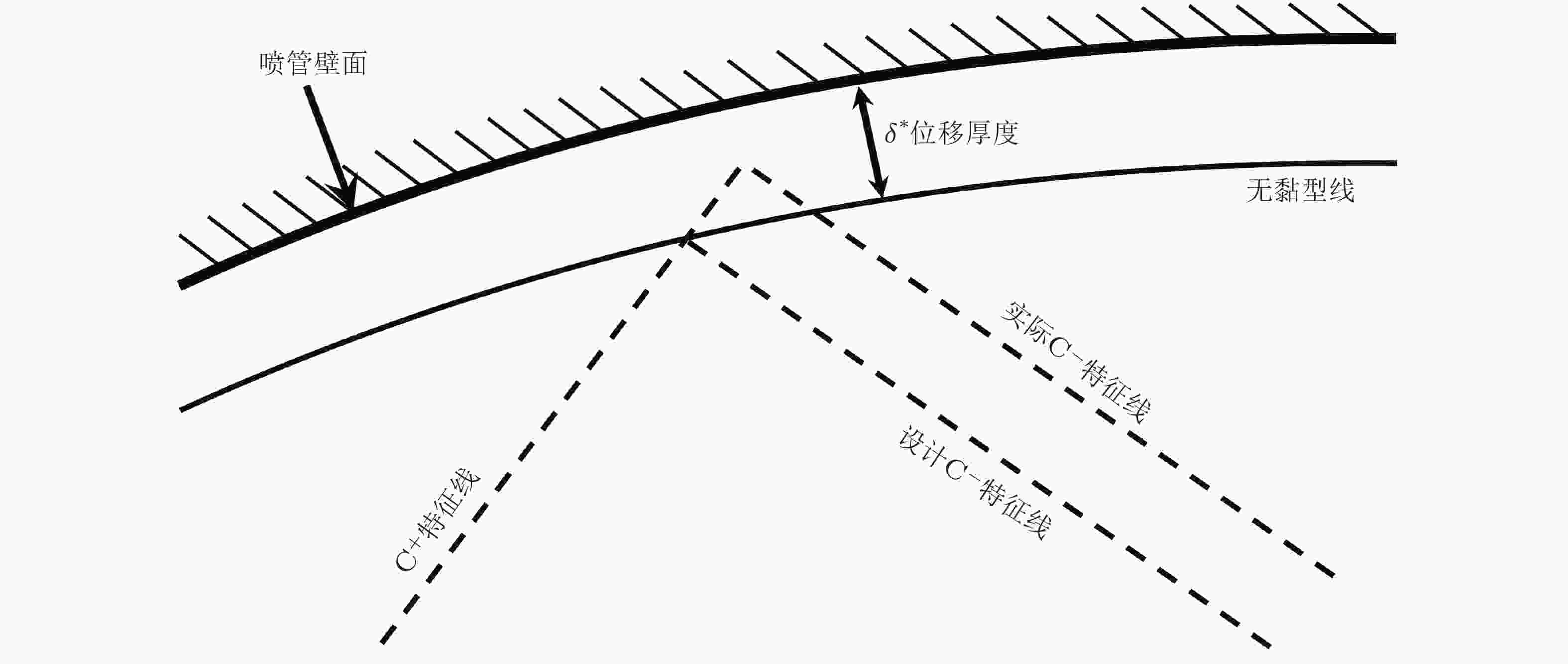
图 14 实际特性线反射与设计特性线反射之间的滞后性问题 (Craddock 2000, Chan et al. 2018)
图 15 基于CFD的设计优化流程 (Korte et al. 1992d)
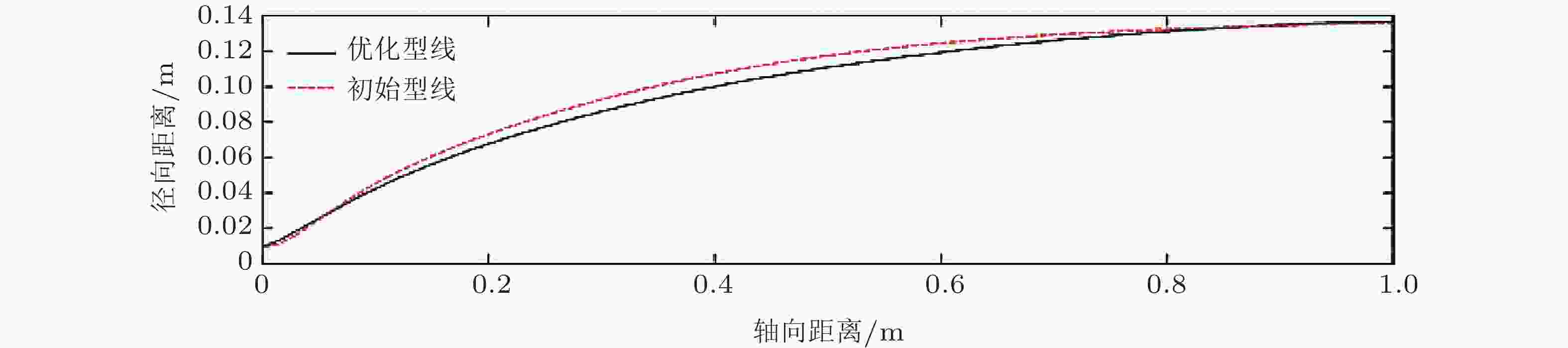
图 16 Chan等方法优化喷管的初始型线和最终型线对比
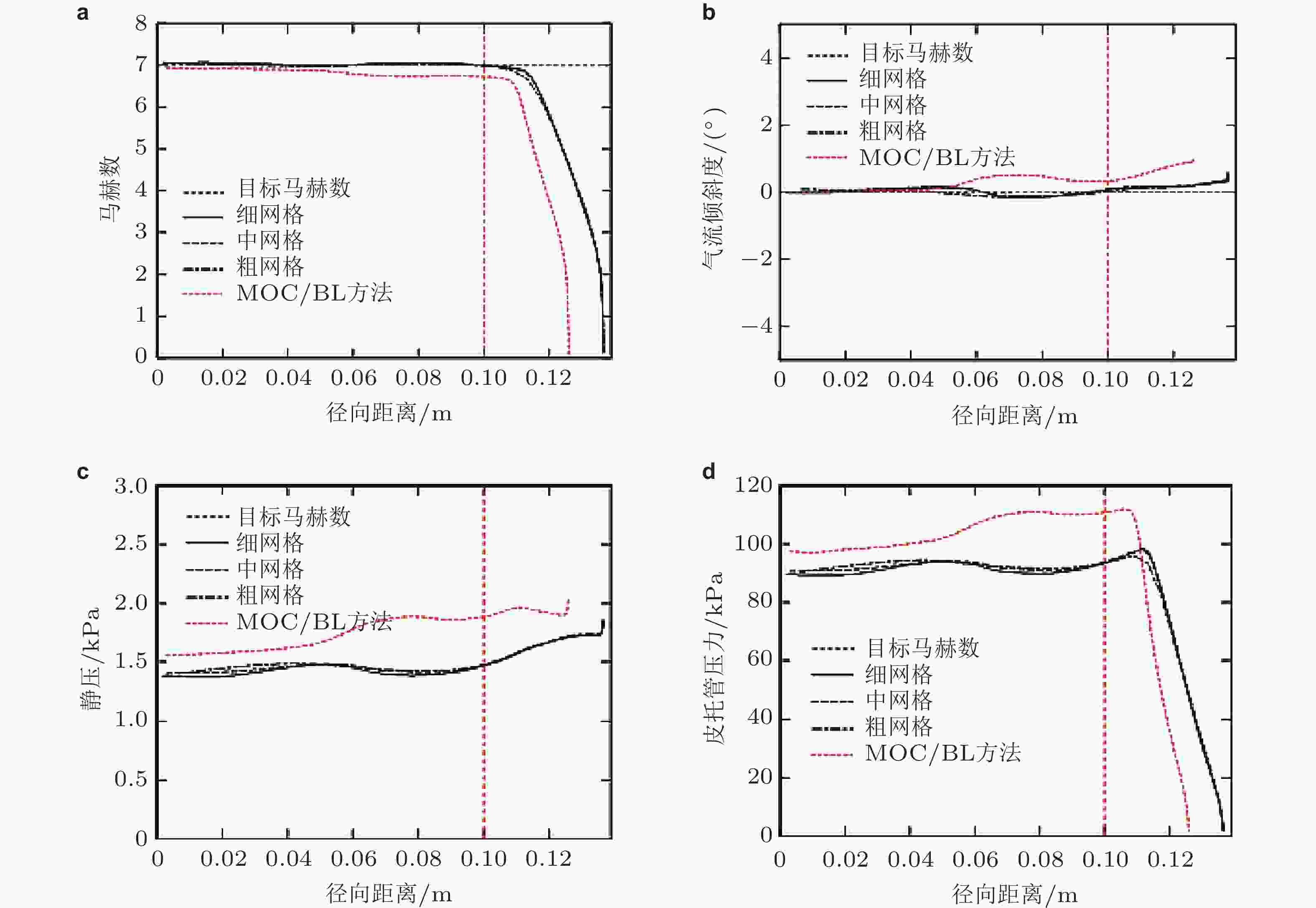
$ (Ma = 7) $ (Chan et al. 2018)图 17 Chan等CFD分析设计方法和MOC/BL的喷管出口流动特性比较 (Chan et al. 2018). (a) 马赫数, (b)气流倾斜度, (c) 静压, (d)皮托管压力
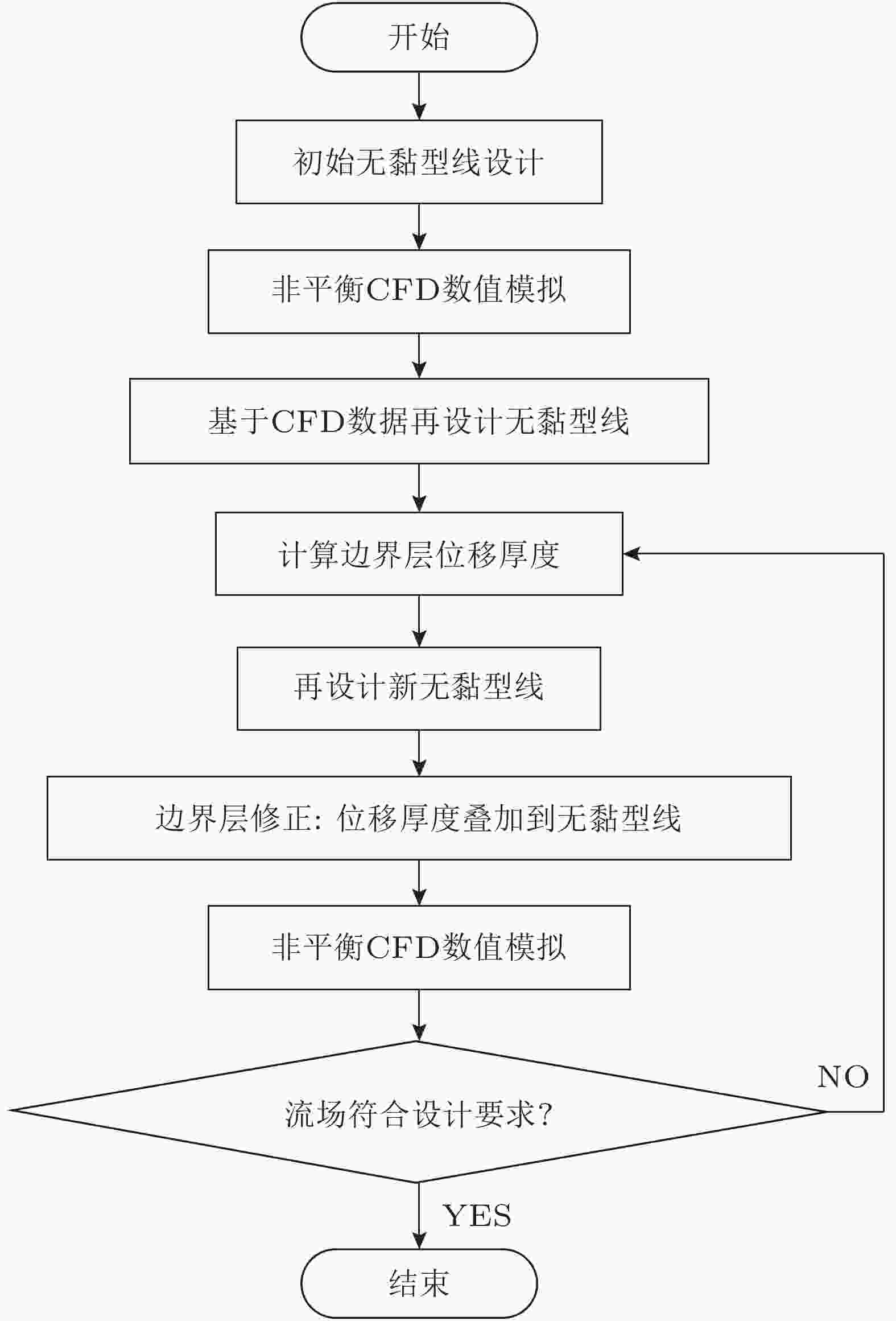
图 18 基于CFD的迭代优化方法流程图 (唐蓓等2019)
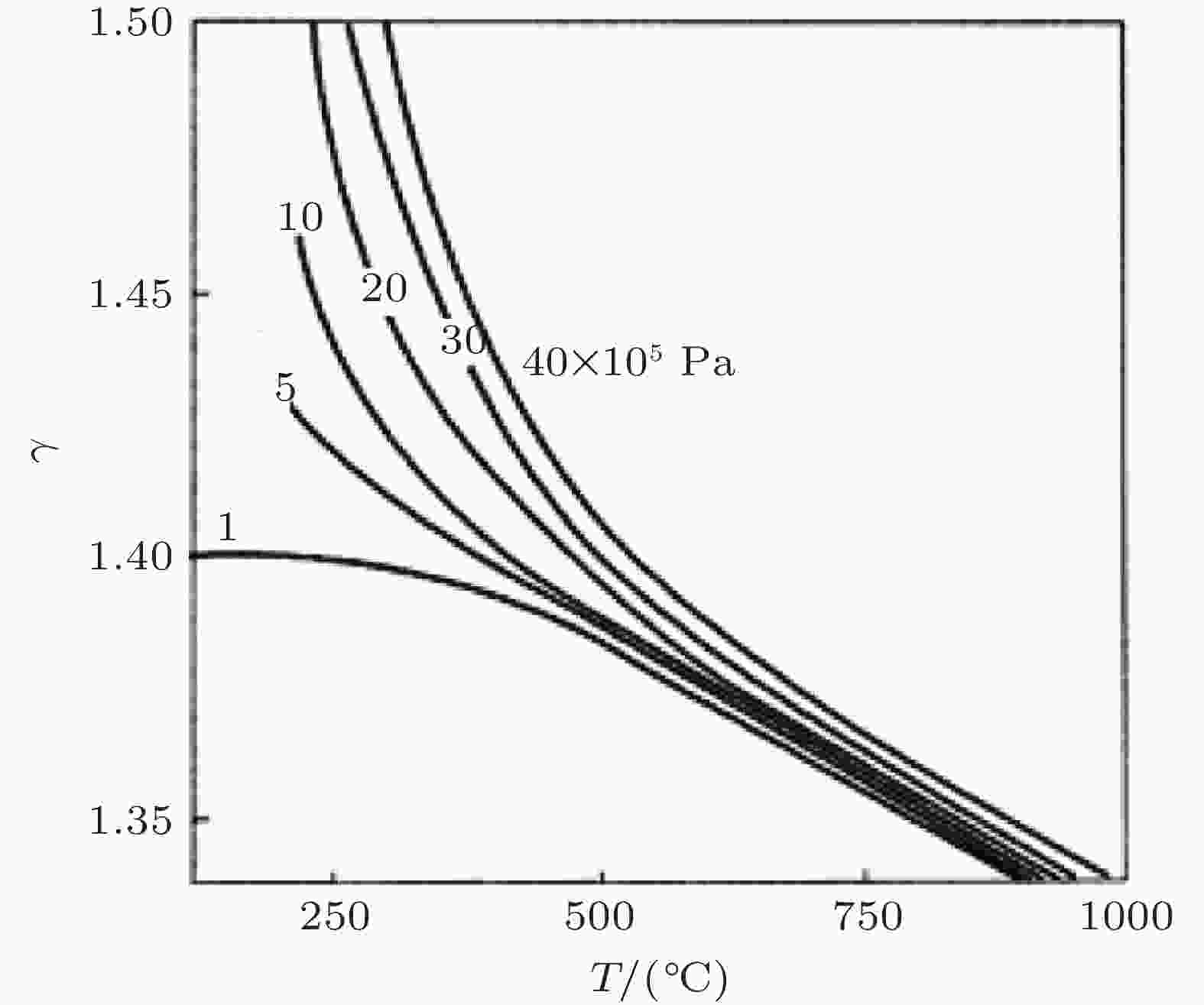
图 19 温度、压力对比热容比的影响 (易仕和等2013)
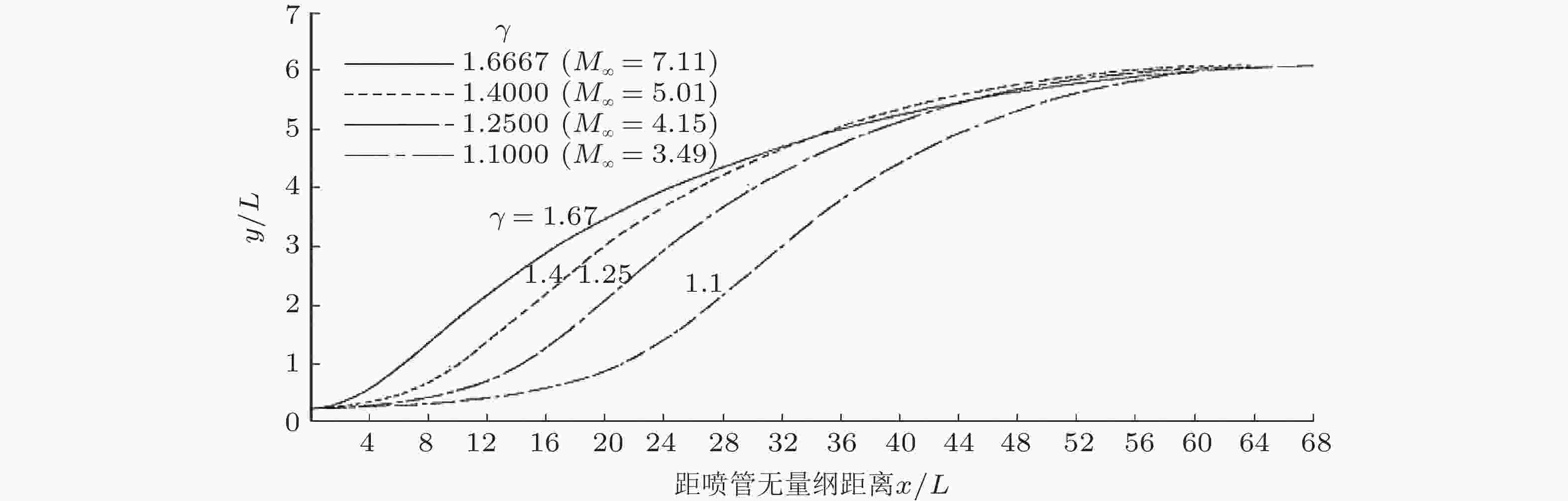
图 20 在相同面积比时比热容比对喷管型线的影响 (Johnson et al. 1975)
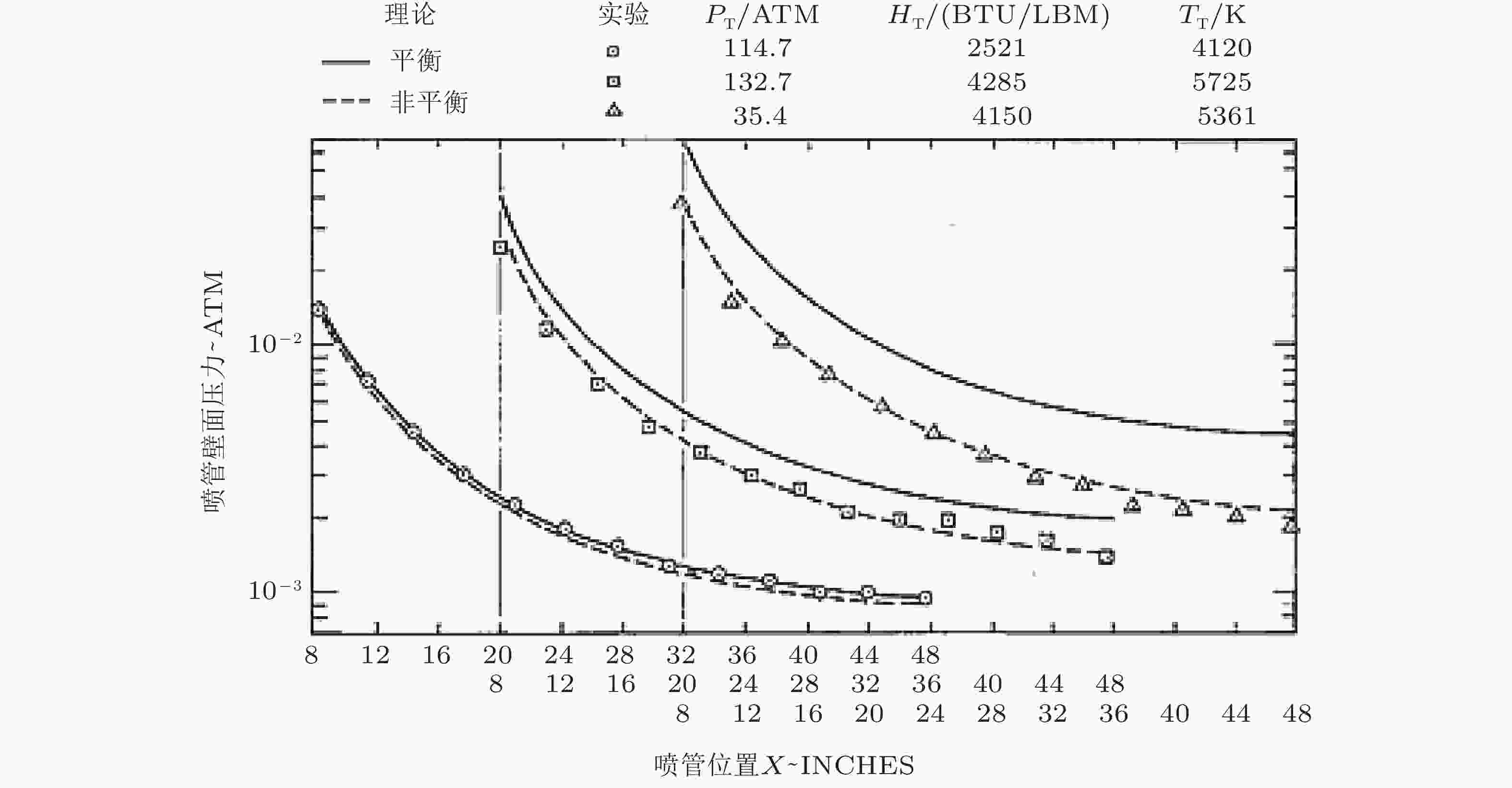
图 21 喷管壁面压力对比 (Zonars 1967)
图 22 真实气体和理想气体喷管设计数值结果比较 (Korte 2000). (a) 轴线马赫数分布, (b) 喷管出口马赫数分布
图 23 喷管型线的样条插值修正 (Shope 2005)
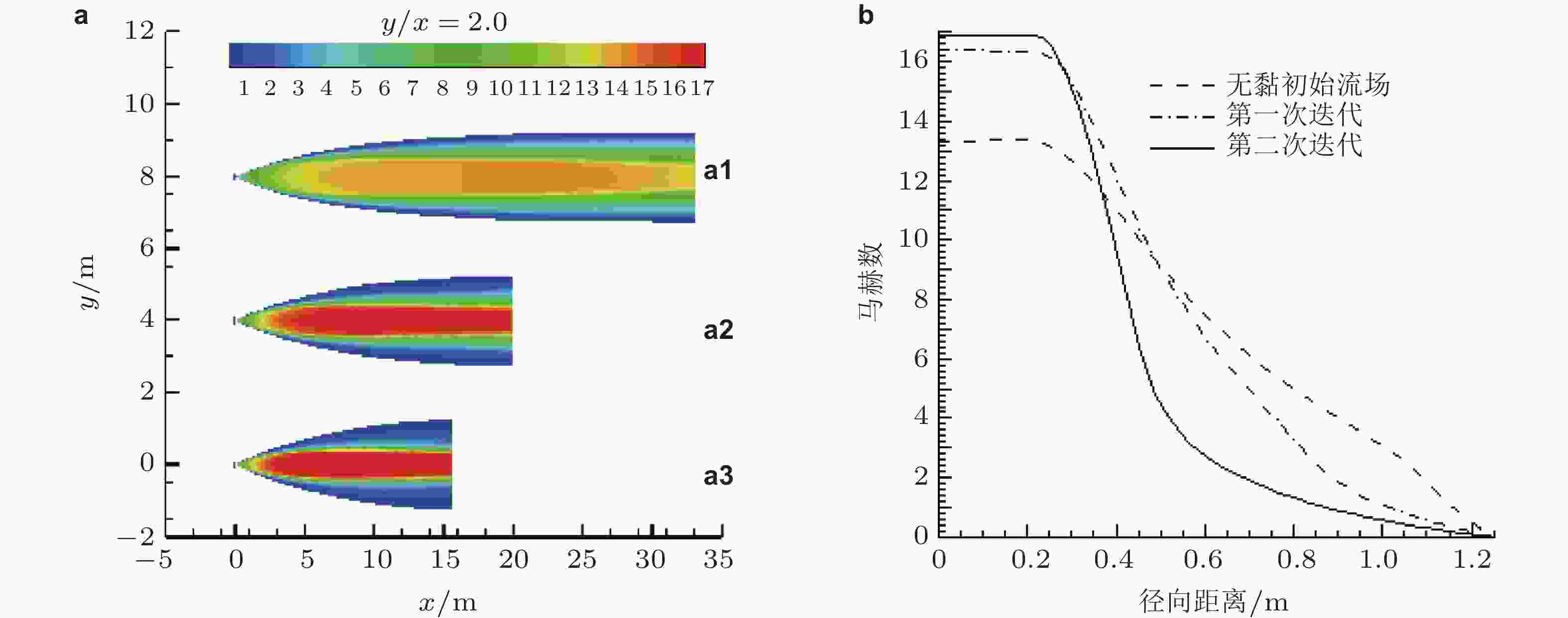
图 24
$ Ma = 17 $ 喷管迭代修正优化结果. (a1) 无黏型线计算得到的初值流场, (a2) 迭代第一次结果, (a3) 迭代第二次结果; (b) 喷管出口马赫数分布 (唐蓓等2019)图 25 马赫数与面积比关系 (唐蓓等2019)
图 26 Ma=6喷管出口马赫数分布对比 (唐蓓等2019)
表 1
$ Ma = 6 $ 喷管流场马赫数均匀性 (唐蓓等2019)出口平均马赫数 最大马赫数偏差 马赫数分布均方根 模型1 6.24 0.076 0.039 模型2 6.70 0.038 0.013 模型3 5.96 0.025 0.010 -
[1] 胡振震, 李震乾, 石义雷, 陈爱国. 2016. 基于轴线马赫数分布的喷管扩张段无黏型面设计. 实验流体力学, 30: 97-104 (Hu Z Z, Li Z Q, Shi Y L, et al. 2016. Design of nozzle inviscid contour based on axial Mach number distribution. Journal of Experiments in Fluid Mechanics, 30: 97-104). [2] 姜宗林, 李进平, 赵伟, 刘云峰, 俞鸿儒. 2012. 长试验时间爆轰驱动激波风洞技术研究. 力学学报, 44: 824-831 (Jiang Z L, Li J P, Zhao W, Liu Y F, Yu H R. 2012. Investigating into techniques for extending the test-duration of detonationdriven shock tunnels. Chinese Journal of Theoretical and Applied Mechanics, 44: 824-831). [3] 罗长童, 汪运鹏, 姜宗林. 2019. 一种高焓风洞喷管型线的光滑化定型方法. 专利号: 2018115040917Luo C T, Wang Y P, Jiang Z L. 2019. A smoothing forming method for nozzle profile of high enthalpy wind tunnel. Patent No.: 2018115040917 [4] 孙启志. 2005. Φ1米高超声速风洞M7, 8喷管设计及流场校测. [硕士论文]. 长沙: 国防科学技术大学Sun Q Z. 2005. Mach number seven and eight nozzle design of the diameter one meter hypersonic wind tunnel and flow field testing. [Master Thesis]. Changsha: National University of Defense Technology [5] 唐蓓, 汪运鹏, 姜宗林. 2019. 大尺度高焓激波风洞喷管设计研究. 中国科学: 物理学力学天文学, 49: 074701 (Tang B, Wang Y P, Jiang Z L. 2019. Nozzle design for a large-scale high-enthalpy shock tunnel. Sci Sin-Phys Mech Astron, 49: 074701). doi: 10.1360/SSPMA2018-00414 [6] 伍荣林, 王振羽. 1985. 风洞设计原理. 北京: 北京航空学院出版社Wu R L, Wang Z Y. 1985. Wind Tunnel Design Principles. Beijing: Beijing Aviation Institute Press [7] 徐立功. 1992. 自由活塞激波风洞. 力学进展, 22: 324-331 (Xu L G. 1992. A free-piston shock tunnel. Advances in Mechanics, 22: 324-331). [8] 童秉纲, 孔祥言, 邓国华. 1990. 气体动力学. 北京: 高等教育出版社Tong B G, Kong X Y, Deng G H. 1990. Aerodynamics. Beijing: Higher Education Press [9] 易仕和, 赵玉新, 何霖, 张敏莉. 2013. 超声速与高超声速喷管设计. 北京: 国防工业出版社Yi S H, Zhao Y X, He L, Zhang M L. 2013. Supersonic and Hypersonic Nozzle Design. Beijing: National Defense Industry Press [10] 张涵信. 2004. 2020年中国空气动力学发展研究. 2020年中国科学和技术发展研究 (下)//2020年中国科学和技术发展研究暨科学家讨论会Zhang H X. 2004. Research on the development of China’s aerodynamics in 2020. Research on China’s Science and Technology Development in 2020 (part 2) [11] 张敏莉, 易仕和, 赵玉新. 2007. 超声速短化喷管的设计和试验研究. 空气动力学学报, 25: 500-503 (Zhang M L, Yi S H, Zhao Y X. 2007. The design and experimental investigations of supersonic length shorted nozzle. Acta Aerodynamica Sinica, 25: 500-503). [12] 赵一龙, 赵玉新, 王振国, 易仕和. 2012. 超声速型面可控喷管设计方法. 国防科技大学学报, 34: 1-4 (Zhao Y L, Zhao Y X, Wang Z G, et al. 2012. Designing method of supersonic nozzle with controllable contour. Journal of National University of Defense Technology, 34: 1-4). [13] Ali M H, Mashud M, Bari A A, Islam M M U. 2012. Numerical solution for the design of minimum length supersonic nozzle. ARPN Journal of Engineering and Applied Sciences, 7: 605-612. [14] Anderson J D Jr. 1970a. A true-dependent analysis for vibrational and chemical nonequilibrium nozzle flows. AIAA Journal, 8: 545-550. doi: 10.2514/3.5703 [15] Anderson J D Jr. 1970b. Time-dependent solutions of nonequilibrium nozzle flows—a sequel. AIAA Journal, 8: 2280-2282. doi: 10.2514/3.6105 [16] Anderson J D Jr. 2003. Modern Compressible Flow: With Historical Perspective. 3rd ed. New York: McGraw–Hill. [17] Anderson J D Jr. 2006. Hypersonic and High-temperature Gas Dynamic. 2nd ed. Virginia: American Institute of Aeronautics and Astronautics. [18] Armstrong A H, Smith M G. 1951. Two-dimensional supersonic: Nozzle design, part I: Theory, Armament Research Establishment. Report No. 5/51. [19] Beckwith I E, Moore J A. 1995. An accurate and rapid method for the design of supersonic nozzles. NACA TN 3322. [20] Benton J. 1989. Design and Navier-Stokes analysis of hypersonic wind tunnel nozzles. [Master Thesis]. Raleigh, NC: North Carolina State University. [21] Benton J, Edwards A, Perkins J. 1990. Limitations of the method of characteristics when applied to axisymmetric hypersonic nozzle design//28th Aerospace Sciences Meeting. AIAA Paper 90-0192. [22] Bird G A. 1957. A Note on Combustion Driven Shock Tubes. AGARD Report No. 146. [23] Busemann A. 1931. Gasdynamik. Handbuch der Experimental-physik. Akademische Verlagsgesellschaft, Leipzig, 4: 341-460. [24] Candler G, Perkins J. 1991. Effects of vibrational nonequilibrium on axisymmetric hypersonic nozzle design//29th Aerospace Sciences Meeting. AIAA Paper 91-0297. [25] Candler G. 2004. APTU Nozzle Code Manual, version 3.0. [26] Candler G. 2005. Hypersonic nozzle analysis using an excluded volume equation of state//38th AIAA Fluid Dynamics Conference and Exhibit, Toronto, Ontario, Canada. AIAA-2005-5202. [27] Chan W Y K, Jacobs P A, Smart M K, Grieve S, Craddock C S, Doherty L J. 2018. Aerodynamic design of nozzles with uniform outflow for hypervelocity ground-test facilities. Journal of Propulsion and Power, 34: 1467-1478. doi: 10.2514/1.B36938 [28] Conte S D. Boor C. 1972. Elementary Numerical Analysis. 2nd ed. New York: McGraw-Hill. [29] Craddock C S. 2000. Design of the axisymmetric hyshot nozzle for T4. Dept. of Mechanical Engineering, Univ. of Queensland Rept. 2/00, Brisbane, QLD, Australia. [30] Cresci R J. 1958. Tabulation of coordinates for hypersonic axisymmetric nozzles. WADC Technical Note 58-300, Pts. I and II. Wright Air Development Div. [31] Crown J C. 1948. Supersonic nozzle design. NACA Technical Note No. 1651. Ames Aeronautical Laboratory. Moffett Field, Calif. [32] Eggers Jr A J. 1949. One-dimensional flows of an imperfect diatomic gas. NACA TN 1861. Ames Aeronautical Laboratory, Moffett Field. [33] Enkenhus K R, Maher E F. 1962. The aerodynamic design of axisymmetric nozzles for high-temperature air. NAVWEPS Rep.7395, U.S. Naval Ord. Lab., White Oak, Md. [34] Erickson W D, Creekmore H S. 1960. A study of equilibrium real-gas effects in hypersonic air nozzles, including charts of thermodynamic properties for equilibrium air. NASA TN D-231. [35] Erickson W D, 1963. Vibrational nonequilibrium flow of nitrogen in hypersonic nozzles. NASA TN D-1810. [36] Foelsch K. 1946. A new method of designing two-dimensional laval nozzles for a parallel and uniform jet. North American Aviation Rep. No. NA-46-235. [37] Foelsch K. 1949. The analytical design of an axially symmetric laval nozzle for a parallel and uniform jet. Int J Orthod, 20: 39-40. [38] Gaffney R L J, Korte J J. 2004. Analysis and design of rectangular-cross-section nozzles for scramjet engine testing//42nd AIAA Aerospace Sciences Meeting and Exhibit. AIAA Paper 2004-1137. [39] Gaffney R L J. 2006. Design of a pulse-facility nozzle using the rotational method of characteristics. Journal of Spacecraft and Rockets, 43: 1216-1224. doi: 10.2514/1.20193 [40] Gaffney R L J. 2007. Erratum on design of a pulse-facility nozzle using the rotational method of characteristics. Journal of Spacecraft and Rockets, 44: 496-504. doi: 10.2514/1.30729 [41] Gollan R J, Jacobs P A. 2013. About the formulation, verification and validation of the hypersonic flow solver eilmer. International Journal for Numerical Methods in Fluids, 73: 19-57. doi: 10.1002/fld.3790 [42] Gordon S, McBride B J. 1996. Computer program for calculation of complex chemical equilibrium compositions and applications, i. analysis, ii. users manual and program description. NASA Reference Publication 1311. [43] Guentert E C, Neumann H E. 1959. Design of axisymmetric exhaust nozzles by method of characteristics incorporating a variable isentropic exponent. NASA TR R-33. [44] Hall I M. 1962. Transonic flow in two-dimensional and axially-symmetric nozzles. Quarterly J Mech Appl Math XV, 4: 487-508. [45] Hall J G, Treanor C E. 1968. Nonequilibrium effects in supersonic nozzle flows. AGARDograph No. 124. [46] Harris E L, Albacete L M. 1964. Vibrational relaxation of nitrogen in the nol hypersonic tunnel No. 4. Naval Ordnance Lab., TR 63-221, White Oak, MD. [47] Hannemann K, Itoh K, Mee D J, Hornung H G. 2016. Free Piston Shock Tunnels HEG, HIEST, T4 and T5. Experimental Methods of Shock Wave Research. New York: Springer. [48] Hollis B R. 1992. Real gas flow parameters for NASA langley 22-inch Mach 20 helium tunnel. NASA CR-4462. [49] Huddleston D H. 1989. Aerodynamic design optimization using computational fluid dynamics. [PhD Thesis]. Knoxville, TN: Univ. of Tennessee. [50] Jiang Z, Yu H. 2017. Theories and technologies for duplicating hypersonic flight conditions for ground testing. National Science Review, 4: 290-296. doi: 10.1093/nsr/nwx007 [51] Johnson C B, Boney L R, Ellison J C, Erickson W D. 1963. Real-gas effects on hypersonic nozzle contours with a method of calculation. NASA TN D-1622. [52] Johnson C B, Boney L R. 1975. A method for calculating a real-gas two-dimensional nozzle contour including the effects of gamma. NASA TM X-3243. [53] Keeling S L. 1993. A strategy for the optimal design of nozzle contours//28th AIAA Thermophysics Conference. AIAA Paper 1993-2720. [54] Korte J J. 1991. An explicit upwind algorithm for solving the parabolized navier-stokes equations. NASA TP 3050. [55] Korte J J. 1992a. Aerodynamic design of axisymmetric hypersonic wind-tunnel nozzles using least-squares/parabolized navier-stokes procedure. Journal of Spacecraft and Rockets, 29: 685-691; also AIAA Paper 92-0332. [56] Korte J J, Hedlund E, Anandakrishnan S. 1992b. A comparison of experimental data with CFD for the NSWC hypervelocity wind tunnel Mach14 nozzle//17th Aerospace Ground Testing Conference. AIAA Paper 92-4010. [57] Korte J J, Kumar A, Singh D J, Grossman B. 1992c. Least-Squares parabolized navier-stokes procedure for optimizing hypersonic wind-tunnel nozzles. Journal of Propulsion and Power, 8: 1057-1063. doi: 10.2514/3.23592 [58] Korte J, Kumar A, Singh D, White J. 1992d. CAN-DO, CFD-based aerodynamic nozzle design and optimization program for supersonic/hypersonic wind tunnels//17th Aerospace Ground Testing Conference, Reston, Virigina: American Institute of Aeronautics and Astronautics. [59] Korte J J. 1993. A CFD-based aerodynamic design procedure of hypersonic wind-tunnel nozzles//AGARD Conference Proceedings, 514: 36.1-36.10. [60] Korte J J, Hodge J S. 1994. Prediction/measurement of flow quality in hypersonic wind-tunnel nozzles designed using CFD//18th AlAA Aerospace Ground Testing Conference. AIAA-94-2544. [61] Korte J J, Hodge J S. 1995. Flow quality of hypersonic wind-tunnel nozzles designed using computational fluid-dynamics. Journal of Spacecraft and Rockets, 32: 569-580. doi: 10.2514/3.26655 [62] Korte J J. 2000. Inviscid design of hypersonic wind tunnel nozzles for a real gas//38th Aerospace Sciences Meeting and Exhibit. AIAA Paper 2000-0677. [63] Lukasiewicz J. 1973. Experimental methods of hypersonics, Gasdynamics, part I. New York: Dekker. 3: 80-86. [64] McBride B J, Gordon S, Reno M A. 1993. Coefficients for calculating thermodynamic and transport properties of individual species. NASA Technical Memorandum 4513. [65] McBride B J, Zehe M J, Gordon S. 2002. NASA Glenn coefficients for calculating thermodynamic properties of individual species. NASA/TP-2002-211556. Glenn Research Center, Cleveland, Ohio. [66] Nelder J A, Mead R. 1965. A simplex method for function minimization. Computer Journal, 7: 308-313. doi: 10.1093/comjnl/7.4.308 [67] Potter J L, Carden W H. 1968. Design of axisymmetric contoured nozzles for laminar hypersonic flow. Journal of Spacecraft and Rockets, 5: 1095-1100. doi: 10.2514/3.29427 [68] Prandtl L, Busemann A. 1929. Naherungsverfahren zur zeichnerischen ermittlung von ebenen strömungen mit übershllgeschwindigkeit. Füssli Verlag, Zurich, Switzerland. [69] Preiswerk E. 1940. Application of the methods of gas dynamics to water flows with free surface, part I. flows with no energy dissipation. NACA TM 934. [70] Puckett Allen E. 1946. Supersonic nozzle design. Journal of Applied Mechanics, 13: A265-A270. doi: 10.1115/1.4009584 [71] Ruptash J. 1952. Supersonic wind tunnels theory, design and performance. University of Toronto, Institute of Aerophysics. [72] Scales L S. 1985. Introduction to Non-linear Optimization. New York: Springer-Verlag. [73] Shapiro A H. 1953. The Dynamics and Thermodynamics of Compressible Fluid Flow. New York: The Ronald Press Company. [74] Shope F L. 2004. Design optimization of hypersonic test facility nozzle contours using splined corrections. AEDC-TR-2005-2. [75] Shope F L, Tatum K E. 2005. Reacting navier-stokes nozzle contour design optimization//23rd AIAA Applied Aerodynamics Conference. AIAA Paper 2005-5081. [76] Shope F L. 2006. Contour design techniques for super/hypersonic wind tunnel nozzles//24th AIAA Applied Aerodynamics Conference. AIAA Paper 2006-3665. [77] Sivells J C, Payne R G. 1959. A method of calculating turbulent boundary growth at hypersonic Mach numbers. AEDCTR-59-3. [78] Sivells J C. 1963. Aerodynamic design and calibration of the VKF 50-inch hypersonic wind tunnels. AEDC-TDR-62-230 (AD 299774), Arnold Engineering Development Center, Tullahoma, Tenn. [79] Sivells J C. 1969. Aerodynamic design of axisymmetric hypersonic wind tunnel nozzles//4th Aerodynamic Testing Conference. AAIA Paper 69-337. [80] Sivells J C. 1970. Aerodynamic design of axisymmetric hypersonic wind-tunnel nozzles. Journal of Spacecraft and Rockets, 7: 1292-1299. doi: 10.2514/3.30160 [81] Sivells J C. 1978. A computer program for the aerodynamic design of axisymmetric and planar nozzles for supersonic and hypersonic wind tunnels. AEDC-TR-78-63. [82] Stalker R J. 1967. A study of the free-piston shock tunnel. AIAA Journal, 5: 2160-2165. doi: 10.2514/3.4402 [83] Stalker R J. 1989. Hypervelocity aerodynamics with chemical nonequilibrium. Ann. Rev. Fluid Mech, 21: 37-60. doi: 10.1146/annurev.fl.21.010189.000345 [84] Tajfar A H, Hall I M. 1991. Design of a nozzle for a hypersonic wind tunnel. AERO-REPT-9113, ETN-92-92779. [85] Tolle R. 1997. A new optimum design code for hypersonic nozzles, utilizing response surface methodology//35th AIAA Aerospace Sciences Meeting and Exhibit. AIAA Paper 1997-0519. [86] Wright M J, Candler G V. 1998. Data-parallel line relaxation method for the Navier-Stokes equations. AIAA Journal, 36: 1603-1609. doi: 10.2514/2.586 [87] Yu H R, Esser B, Lenartz M, Groenig H. 1992. Gaseous detonation driver for a shock tunnel. Shock Waves, 2: 245-254. doi: 10.1007/BF01414760 [88] Zonars D. 1967. Nonequilibrium regime of airflows in contoured nozzles: Theory and experiments. AIAA Journal, 5: . [89] Zucrow M J, Hoffman J D. 1997. Gas Dynamics, Volume 2. Multidimensional Flow. New York: John Wiley & Sons, Inc. -




 下载:
下载: